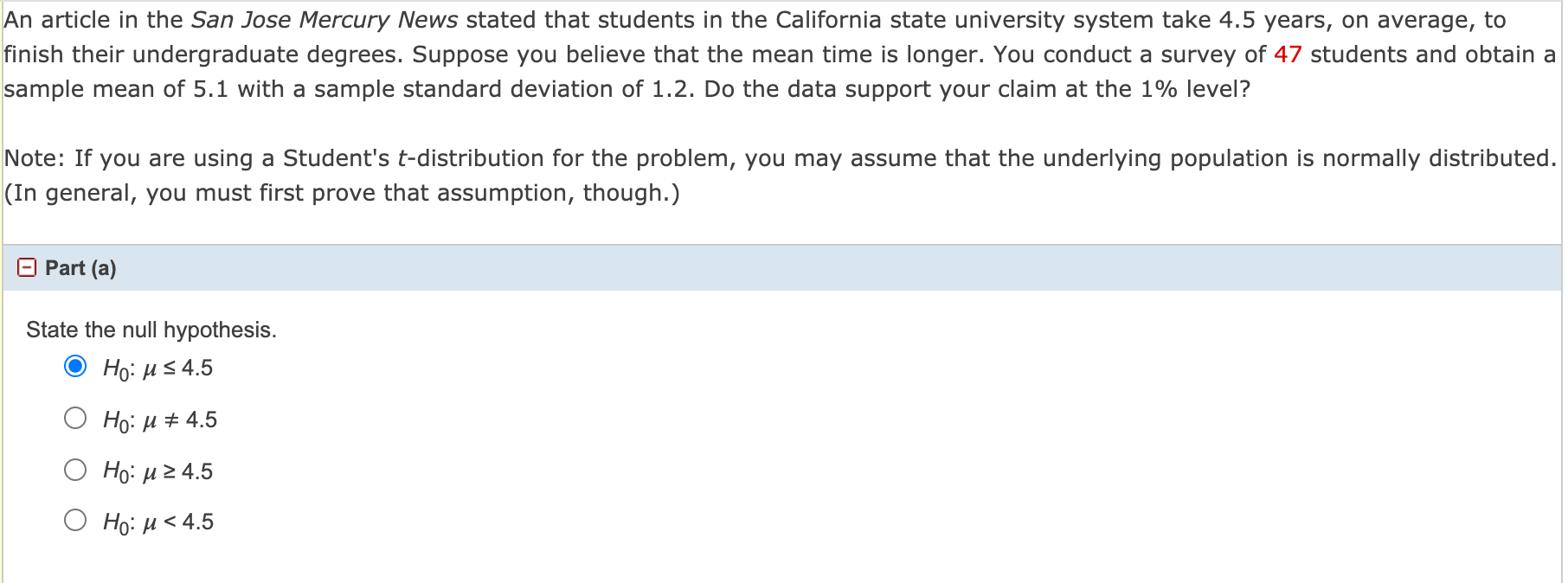
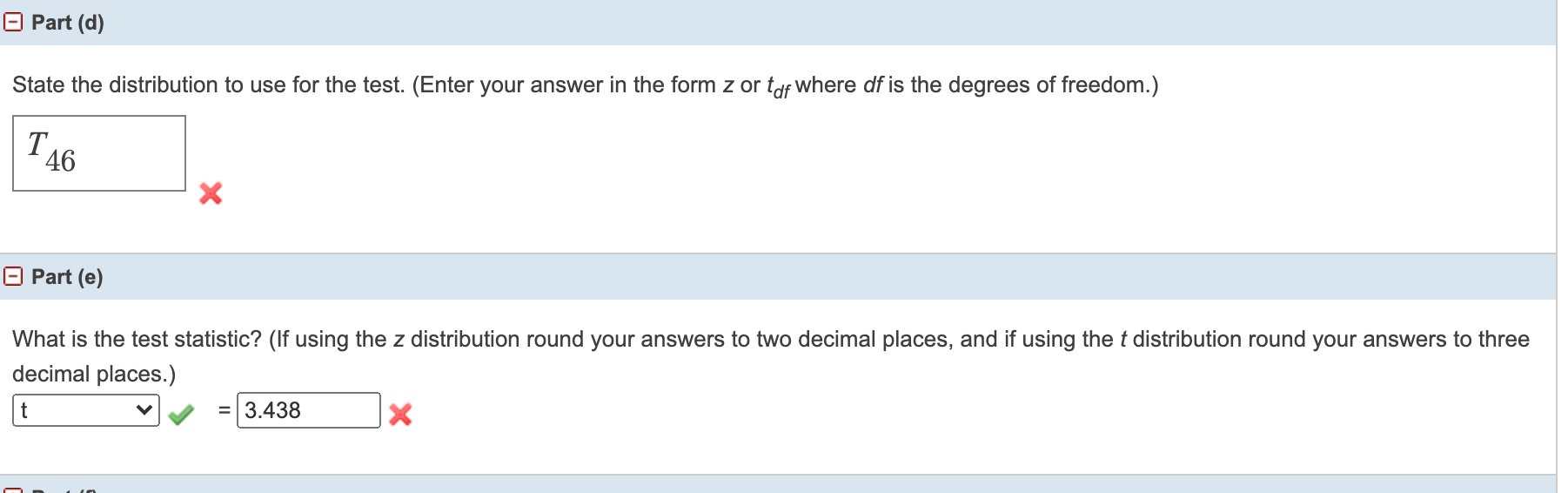
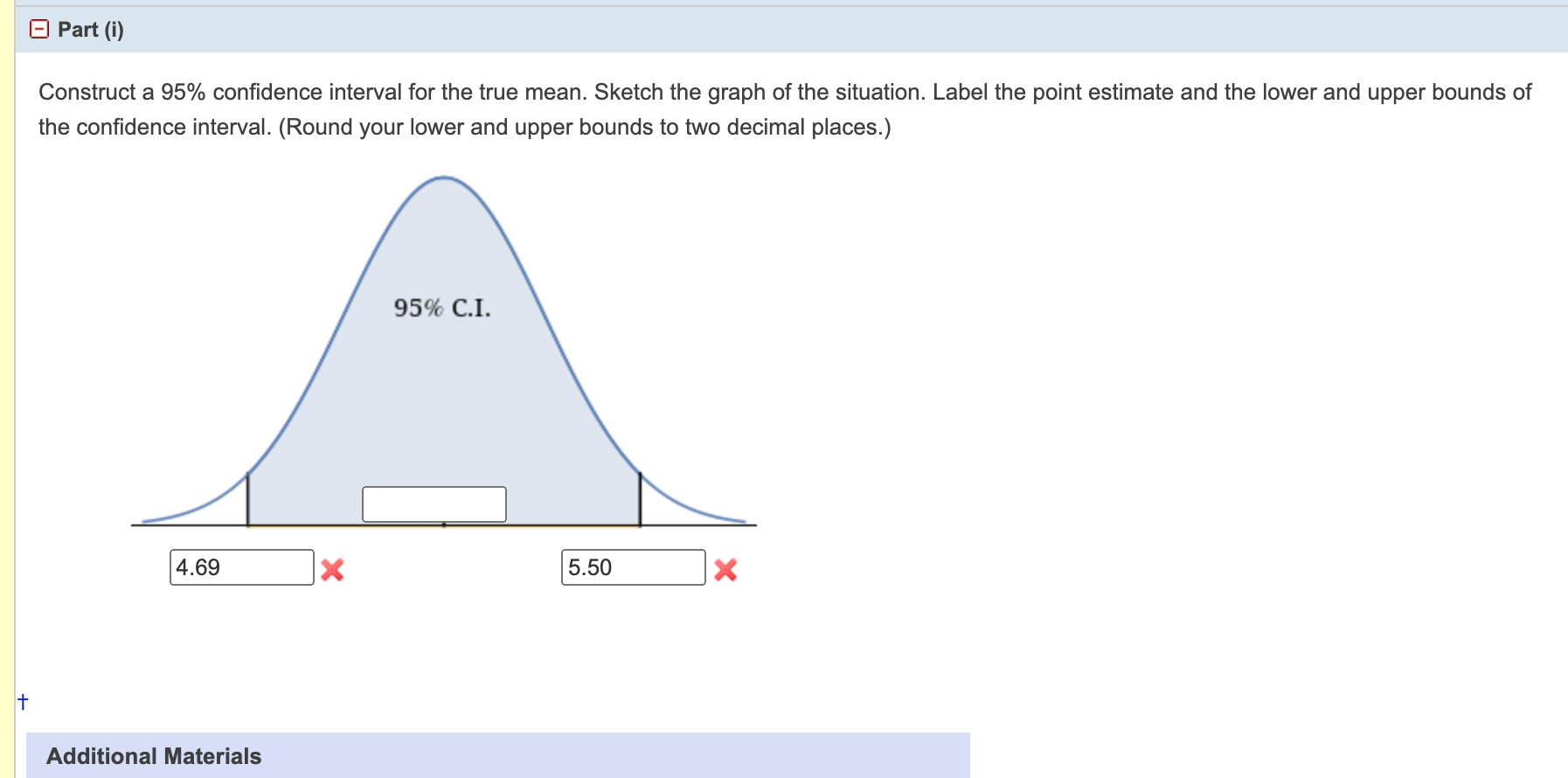
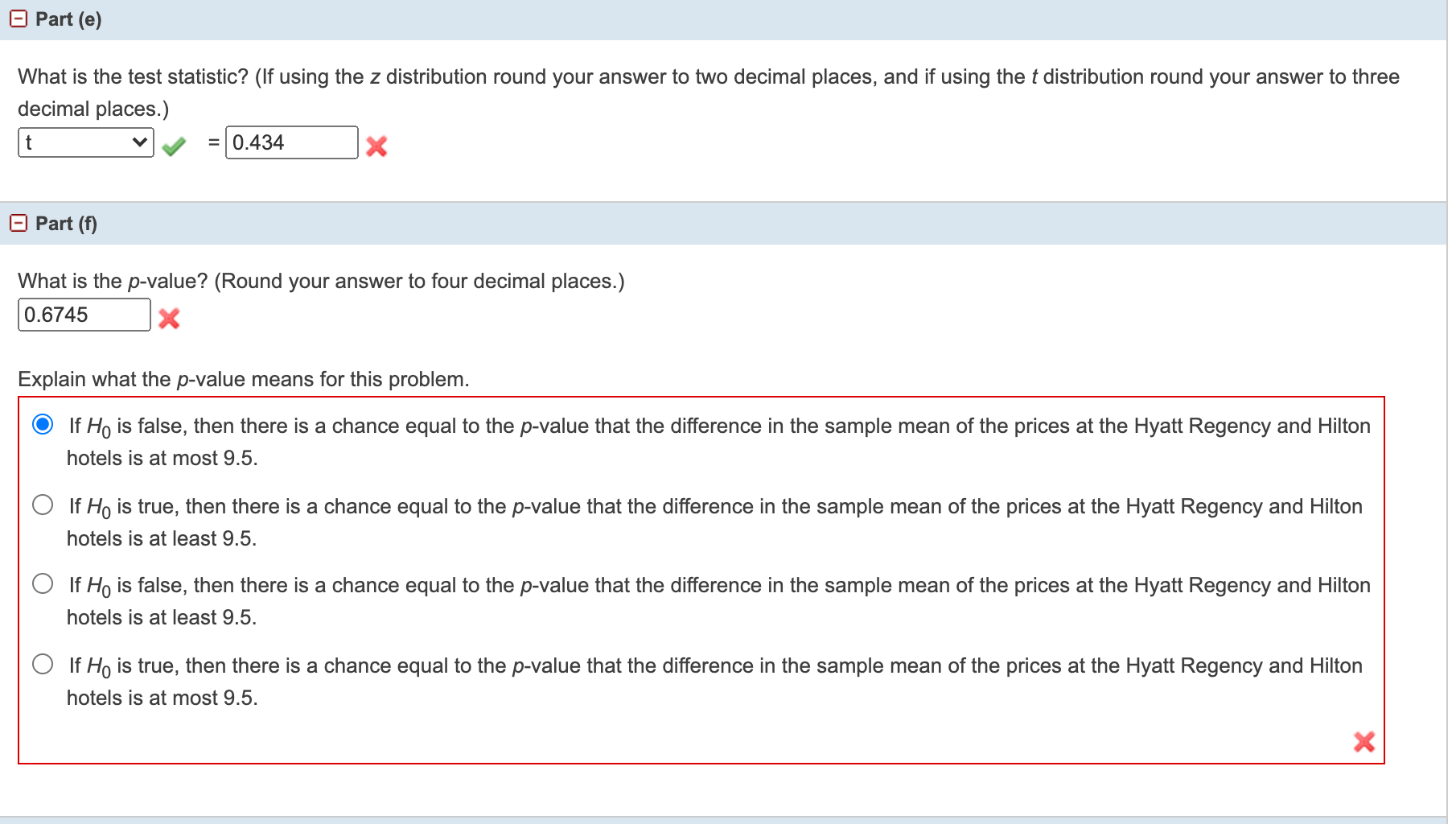
An article in the San Jose Mercury News stated that students in the California state university system take 4.5 years, on average, to finish their undergraduate degrees. Suppose you believe that the mean time is longer. You conduct a survey of 47 students and obtain a sample mean of 5.1 with a sample standard deviation of 1.2. Do the data support your claim at the 1% level? Note: If you are using a Student's t-distribution for the problem, you may assume that the underlying population is normally distributed. (In general, you must first prove that assumption, though.) Part (a) State the null hypothesis. Ho: M 4.5 Ho: #4.5 : > 4.5 Ho: Part (f) What is the p-value? (Round your answer to four decimal places.) 0.6745 Explain what the p-value means for this problem. If Ho is false, then there is a chance equal to the p-value that the difference in the sample mean of the prices at the Hyatt Regency and Hilton hotels is at most 9.5. If Ho is true, then there is a chance equal to the p-value that the difference in the sample mean of the prices at the Hyatt Regency and Hilton hotels is at least 9.5. If Ho is false, then there is a chance equal to the p-value that the difference in the sample mean of the prices at the Hyatt Regency and Hilton hotels is at least 9.5. If Ho is true, then there is a chance equal to the p-value that the difference in the sample mean of the prices at the Hyatt Regency and Hilton hotels is at most 9.5.