Answered step by step
Verified Expert Solution
Question
1 Approved Answer
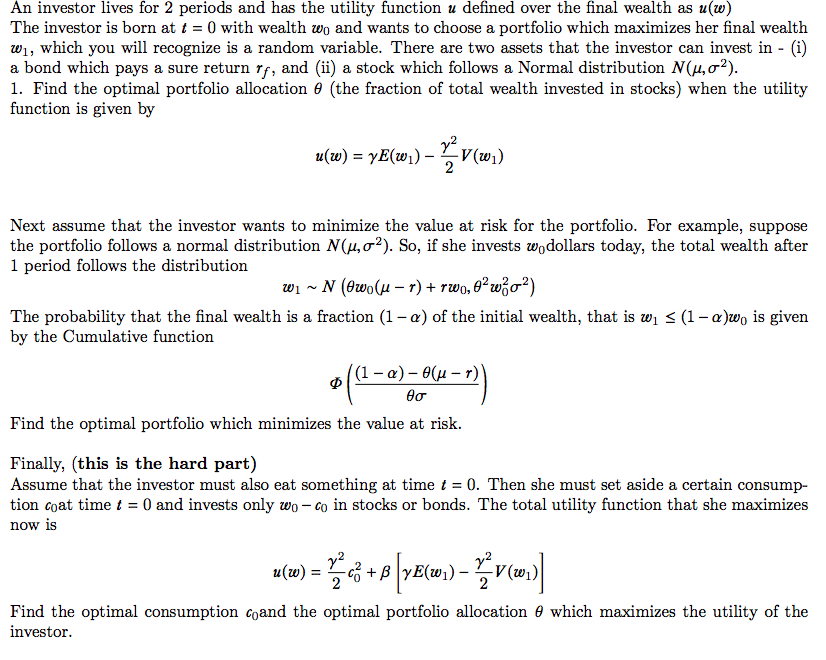
An investor lives for 2 periods and has the utility function u defined over the final wealth as u(w) The investor is born at t
An investor lives for 2 periods and has the utility function u defined over the final wealth as u(w) The investor is born at t = 0 with wealth w0 and wants to choose a portfolio which maximizes her final wealth w1, which you will recognize is a random variable. There are two assets that the investor can invest in - (i) a bond which pays a sure return rf , and (ii) a stock which follows a Normal distribution N (, ?2 ).
- Find the optimal portfolio allocation ? (the fraction of total wealth invested in stocks) when the utility function is given by u(w) = ?E(w1) ? ?^2/2*V (w1).
- Next assume that the investor wants to minimize the value at risk for the portfolio. For example, suppose the portfolio follows a normal distribution N (, ?2 ). So, if she invests w0dollars today, the total wealth after 1 period follows the distribution w1 ? N (?w0( ? r) + rw0, ?^2w0^2?^2). The probability that the final wealth is a fraction (1 ? ?) of the initial wealth, that is w1 ? (1 ? ?)w0 is given by the Cumulative function ? [ (1 ? ?) ? ?( ? r) /?? ].Find the optimal portfolio which minimizes the value at risk.
- Finally, assume that the investor must also eat something at time t = 0. Then she must set aside a certain consumption c0at time t = 0 and invests only w0 ?c0 in stocks or bonds. The total utility function that she maximizes now is u(w) = ?^2/2*c0^2 + ?* [?E(w1) ? ?^2/2*V (w1)]. Find the optimal consumption c0and the optimal portfolio allocation ? which maximizes the utility of the investor.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


