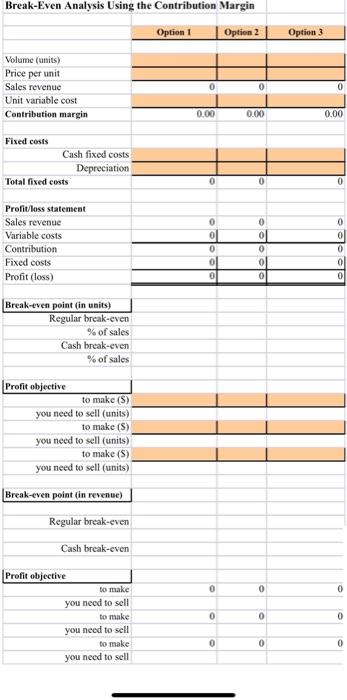
Analyze the following three options and preform a Break-Even analysis for each using the contribution margin method for Karl's T-Shirt Company. Option 1 Option 1 is to import the finished goods internationally for sale domestically in a retail outlet, this option requires a very high minimum purchase order (250,000 Units) however has the advantage of a lower per unit cost and lower fixed costs. This plan has the following costs and revenue associated: Volume (units): 250,000 Selling Price Per unit: $11.99 Unit Variable Cost: $9.50 Fixed Costs:$255,000 Depreciation: $5,000 Option 2 This option requires the purchase of a textile machine that can produce fabrics with a higher thread count. Unfinished goods are imported internationally in form of threads and then processed for final sale domestically. It will require an additional investment of $200,000 compared to option 1 but has the advantage of higher quality goods and as such will sell for a higher price. This plan has the following costs and revenue associated: Volume (units): 180,000 Selling Price Per unit: $25.00 Unit Variable Cost: $21.00 Fixed Costs:$455,000 Depreciation: $10.00 Option 3 The last option is to sell custom T-shirts that are made to order. This option requires the highest fixed costs because it requires a printing machine to print custom labels or designs for customers. Variable costs for this option are low compared to other options and has the advantage of charging a premium for the shirts being custom and "made-to-order". This plan has the following costs and revenue associated: Volume (units): 80,000 Selling Price Per unit: $35.00 Unit Variable Cost: $15.00 Fixed Costs:$1,200,000.00 Depreciation: $40,000 Break-Even Analysis Using the Contribution Margin Option 1 Option 2 Option 3 Volume (units) Price per unit Sales revenue Unit variable cost Contribution margin 0 0 0.00 0.00 0.00 Fixed costs Cash fixed costs Depreciation Total fixed costs 0 0 0 0 0 0 Profit/loss statement Sales revenue Variable costs Contribution Fixed costs Profit (loss) 0 0 0 le ole 0 0 Break-even point in units) Regular break-even % of sales Cash break-even % of sales Profit objective to make (S) you need to sell (units) to make (S) you need to sell (units) to make (S) you need to sell (units) Break-even point (in revenue) Regular break-even Cash break-even 0 0 0 Profit objective to make you need to sell to make you need to sell to make you need to sell 0 0 0 0 0 0