
Answer 1-13
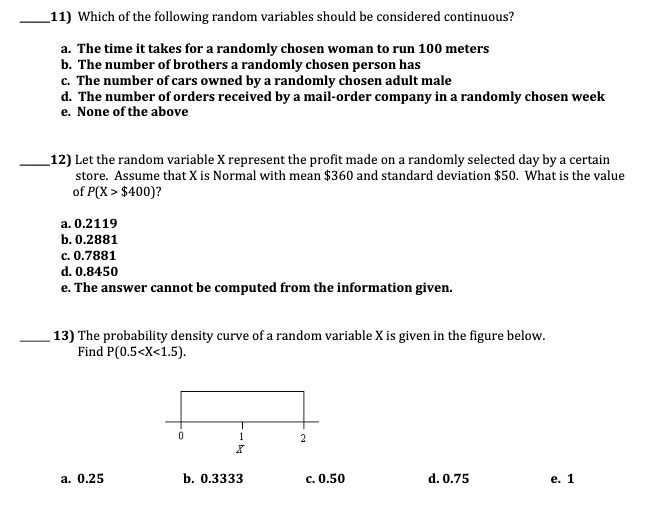
_1] An inspector randomly selects 5 packages of ground beef and will consider the store in violation if more than 2 of the packages are either Incorrectly marked or overweight. Experience has shown him that the packages are either incorrectly marked or overweight 85% of the time What is the probability that the store will he considered in violation? a. 8.8244 b. 8.8288 c. 8.1382 d. 8.8588 2. 8.9234 2] A fair die is tossed 3 times. The probability that a 1 occurs on exactly one roll ofthe die is a a 1 m (.1) E) (i) 11-8838) r GJG) (E) i [3(2) 3] Mr. and Mrs. [ones have ve children. The live births were independent with no multiple births. Assuming that the chances of having a boy or girl are the same, what is the probability that the family has at least two girls? a. 8.1875 b. 8.3125 c. 8.4888 d. 8.5888 2. 8.8125 _11-] A weigh station has two scales available to measure the weight of a truck a local weigh station. it\" the true weight of a truck is 18.8 88 pounds measurements with the rst instrument vary with mean 18l888 pounds and standard deviation I18 pounds. Measurements with the second scale vary widt mean 18,888 pounds and standard deviation 28 pounds. Tt'ou malte one measurement with each scale. Your results are L for the rst scale and I: for the second scale and the measurements are independent. it makes sense to give more effect to the less variable measurement because it is more likely to be closer to the truth Eonsider the following weighted average W: l 3 Find and interpret the standard deviation of W = the weighted average of the two measurements. [a] 18.83; The weighted average tends to vary from the mean weight of 18888 pounds by about 18.83 pounds. [b] 18. 83 ; The true weighted average tends to be 18.83 pounds greater than the weighted average of the measurements. [c] 25.88; The weighted average tends to vary from the mean weight of 18,888 pounds by about 25.88 pounds. [:1] 25.88 ; The true weighted average tends to be 25.88 pounds greater than the weighted average of the measurements. [e] 28.48; The weighted average tends to vary from the mean weight of 18888 pounds by about 28.48 pounds. 5) Suppose we pick two oranges at random from the bin. The difference in the weights of the two oranges selected (the weight of the first orange minus the weight of the second orange) is a random variable with a mean (in ounces) of a. 10 b. 1 c. 1.41 d. o e. 5 6) Suppose we pick two oranges at random from the bin. The difference in the weights of the two oranges selected (the weight of the first orange minus the weight of the second orange) is a random variable with a standard deviation (in ounces) of a. 0 b. 1 C. 2 d. 2.2 e. 1.41 7) In a large population of college students, 20% of the students have experienced feelings of math anxiety. If you take a random sample of 10 students from this population, the probability that exactly 2 students have experienced math anxiety is a. 0.3020 b. 0.2634 c. 0.2013 d. 0.5 e. 1 f. None of the above 8) Refer to the previous problem. The standard deviation of the number of students in the sample who have experienced math anxiety is a. 0.0160 b. 1.265 c. 0.2530 d. 1 e. 0.2070 9) Karen is playing an instant lottery game. What is the probability that she will not win until the 8th try if the probability of winning is 1 out of 100 on a single try? a. 0.0009 b. 0.0093 c. 0.0993 d. 0.9321 e. 0.9907 10) A factory makes silicon chips for use in computers. It is known that about 90% of the chips meet specifications. Every hour a sample of 18 chips is selected at random for testing. Assume a binomial distribution is valid. Suppose we collect a large number of these samples of 18 chips and determine the number meeting specifications in each sample. What is the mean of the number of chips meeting specifications? a. 16.20 b. 1.62 C. 4.02 d. 16.0011) Which of the following random variables should be considered continuous? a. The time it takes for a randomly chosen woman to run 100 meters b. The number of brothers a randomly chosen person has c. The number of cars owned by a randomly chosen adult male d. The number of orders received by a mail-order company in a randomly chosen week e. None of the above 12) Let the random variable X represent the profit made on a randomly selected day by a certain store. Assume that X is Normal with mean $360 and standard deviation $50. What is the value of P(X > $400)? a. 0.2119 b. 0.2881 c. 0.7881 d. 0.8450 e. The answer cannot be computed from the information given. 13) The probability density curve of a random variable X is given in the figure below. Find P(0.5











