

Answer all of the following questions pleasejQuery22406888650807895691_1622500162137?
A work power head meeting 11 senior fashioners for four business openings has arranged seven gatherings for the essential day and four for the second day of talking. Expect that the up-and-comers are met in an unpredictable solicitation.
What number of the fundamental four candidates can be needed to be met directly from the beginning? 1(Round your reaction to two decimal spots.)
Improve issue 11 in portion 2.3 of your perusing material about picking three (3) coins, yet anticipate that there are 4 dimes, 4 nickels, and 2 quarters.
According to various perspectives can the assurance be made so the value of the coins is in any occasion a quarter?
I have offered a couple of various ways a chance this and can't figure it out.
For a particular stream, accept the dry season length Y is the amount of consecutive time intervals where the water supply stays under an essential worth y0 (a lack), went before by and followed by periods in which the store outperforms this fundamental worth (an abundance). An article proposes a numerical dissemination with p = 0.397 for this subjective variable. (Round your reactions to three decimal spots.)
(a) What is the probability that a drought continues to go correctly 3 ranges?
(b) At most 3 ranges?
+18+
(c) What is the probability that the length of a drought outperforms its mean worth by in any occasion one standard deviation?
This issue incorporates a reasonable fishpond. Expect that the lake contains 110 fish: 91 green, 18 blue, and 1 silver. A challenger pays $0.60 to erratically get one fish and gets $0.25 if the fish is green, $1.00 if the fish is blue, and $13.00 if the fish is silver.
(All around) does the carnival gain on each play? (If the celebration looses cash, enter a negative dollar total) .
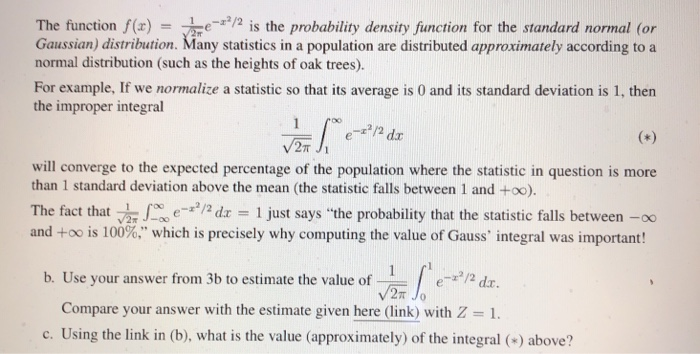
a) Create a vector of 24 elements that represents a carton. Each one of the 24 elements in the vector is an exponentially distributed random variable (T) as shown above, with mean lifetime equal to B. Use the same procedure as in the previous problem to generate the exponentially distributed random variable T. Use the Python function "numpy.random.exponential(beta,n)" to generate n values of the random variable T with exponential probability distribution. Its mean and variance are given by: M =B ; OT=B b) The sum of the elements of this vector is a random variable (C), representing the life of the carton, i.e. C =1+72+ .+124 where Ty, j=1,2,..,24 each is an exponentially distributed random variable. Create the random variable C, i.e simulate one carton of batteries. This is considered one experiment. c) Repeat this experiment for a total of N=10,000 times, i.e. for N cartons. Use the values from the N=10,000 experiments to create the experimental PDF of the lifetime of a carton, f(c). d) According to the Central Limit Theorem the PDF for one carton of 24 batteries can be approximated by a normal distribution with mean and standard deviation given by: He = 24My = 248 ; Gc= GV24 = BV24 Plot the graph of normal distribution with mean /c and standard deviation Or over plot of the experimental PDF on the same figure, and compare the results. e) Create and plot the CDF of the lifetime of a carton, F(c) . To do this use the Python "numpy . cumsum" function on the values you calculated for the experimental PDF. Since the CDF is the integral of the PDF, you must multiply the PDF values by the barwidth to calculate the areas, i.e. the integral of the PDF. If your code is correct the CDF should be a nondecreasing graph, starting at 0.0 and ending at 1.0.4. I've decided to cut PART V of the Gauss' integral problem, the last steps to showing that edx = vn However, I'll put a handout on the course website to finish it up (don't want to leave you hanging). There are still some problems to do around the integral above: a. Explain why we must have (_e s /2 dx = v2x. The easiest argument is a geometric one: What "happens" to the graph if we transform y = e" into y = e- /27 Be careful-it's important that the 2 in the final answer is inside the radical.The function f(x) = e-/2 is the probability density function for the standard normal (or Gaussian) distribution. Many statistics in a population are distributed approximately according to a normal distribution (such as the heights of oak trees). For example, If we normalize a statistic so that its average is 0 and its standard deviation is 1, then the improper integral e -E /2 dx (* ) V27T will converge to the expected percentage of the population where the statistic in question is more than 1 standard deviation above the mean (the statistic falls between 1 and +oo). The fact that 1 V2# ( ex/2 dx = 1 just says "the probability that the statistic falls between -oo and +oo is 100%," which is precisely why computing the value of Gauss' integral was important! b. Use your answer from 3b to estimate the value of e -'/2 dx. V27 Jo Compare your answer with the estimate given here (link) with Z = 1. c. Using the link in (b), what is the value (approximately) of the integral (*) above?Many people trade in their old cars when buying a new one. A car dealer want to understand how the number of miles driven on used Toyota Camrys (a midsize Japanese car) should impact the trade-in value that she offers her customers. She, therefore, select 100 3-year old Toyota Camrys that were sold at auction during the past month. Each car was in top condition and equipped with all the features that come standard with this car. The dealer recorded the price ($1,000) and the number of miles (thousands) on the odometer













