


Answer all questions correctly.,,,
. Consider three alternative policies, each with a different set of outcomes in terms of output and inflation, as shown in following table:
Output Output Output Inflation Inflation Inflation
Policy A Policy B Policy C Policy A Policy B Policy C
500.0 500.0 500.0 3.0 3.0 3.0
515.0 500.0 520.0 3.0 2.8 3.2
530.5 520.0 535.0 3.0 2.0 4.0
546.4 540.0 550. 3.0 1.5 4.5
562.8 562.8 562.8 3.0 1.5 4.5
Potential output is 500 in period 1 and rises 3% per year. The Fed's objective function is ?{-2+(w x -2)}
yt nt
where w=1, and the inflation target is 2.0%.
A. Calculate the value of the objective function over the 5-year period for each of the 3 policies.
B. Which policy is best? Why?
C. Would the answer to part B change if w =5? If so explain.
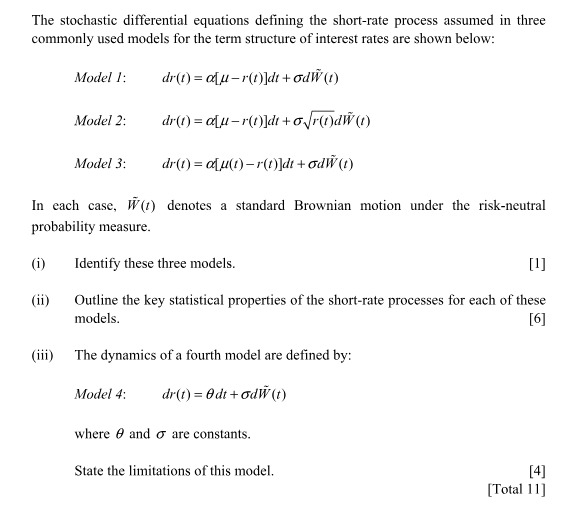
Chapter 2 Section 2.5 Unknown rate of Interest 1. Find the nominal rate of interest convertible semiannually at which the accumulated value of $1000 at the end of 15 years is $3000. 2. Find an expression for the exact effective rate of interest at which payments of $300 at the present, $200 at the end of one year, and $100 at the end of two years will accumulate to $700 at the end of two years. 3. You can receive one of the following two payment streams: (1) 100 at time 0, 200 at time n, and 300 at time 2n. (ii) 600 at time 10. At an annual effective interest rate of i, the present values of the two streams are equal. Given v" = 0.75941, determine i. 4. It is known that an investment of $1000 will accumulate to $1825 at the end of 10 years. If it is assumed that the investment earns simple interest at rate { during the 1" year, 2i during the 2" year, ... 10i during the 10 year, find i. 5. The sum of the accumulated value of 1 at the end of three years at a certain effective rate of interest i, and the present value of 1 to be paid at the end of three years at an effective rate of discount numerically equal to i is 2.0096. Find the rate i.If A() is a strictly positive supermartingale, then zero-coupon bond prices can be modelled using the formula B(, T) = =PLA() | F,] where P is a suitably-chosen A(1) probability measure. (i) (a) Express mathematically the fact that A(t) is a strictly positive supermartingale. (b) Verify that the function A(1) =e-0.05/+0.02#(), where W() denotes standard Brownian motion, satisfies the properties in (1)(a). (c) State why the supermartingale property is required. (d) Write down the name given to this type of process. [7] (ii) By writing A() in the form A(() =e"), or otherwise, show that A(t) satisfies a stochastic differential equation of the form: dA(!) = A(D)[u(D)di +(D)dw()] State the forms of the functions #(t) and GA(!). [4] (iii) (a) Write down or derive a formula for B(t, 7) based on the process A(!) specified in (i)(b). (b) Write down expressions for the instantaneous forward rate f(t,7) and the spot rate R(t, 7) based on this model. (c) State one problem that this model of interest rates has. [4] (iv) Calculate the prices at time 5 according to the model in (ii) of the following risk- free bonds: (a) a 10-year zero-coupon bond (b) a 10-year bond that pays a coupon of 5% at the end of each year. [4] [Total 19](i) Explain what is meant by a default-free bond. [1] (ii) State the possible outcomes of a default. [2] (iii) List four types of credit event. [2] (iv) Explain what is meant by the recovery rate for a bond. [1] [Total 6]The stochastic differential equations defining the short-rate process assumed in three commonly used models for the term structure of interest rates are shown below: Model 1: dr(1) = atu- ro)]di + odw(n) Model 2: dr(1) = atu-r(0)]detour(odw() Model 3: dr() = a[u(1)-r(0)]di + odw() In each case, W() denotes a standard Brownian motion under the risk-neutral probability measure. (i) Identify these three models. [1] (ii) Outline the key statistical properties of the short-rate processes for each of these models. [6] (iii) The dynamics of a fourth model are defined by: Model 4: dr (t) = 0di + odw() where # and of are constants. State the limitations of this model. [4] [Total 11](i) What are the advantages and disadvantages of hire purchase agreements and credit sale agreements from the point of view of the supplier? (ii) State the main distinction between an operating lease and a finance lease. (iii) State the main distinction between a bank loan and a loan facility. (iv) State the main distinction between a loan facility and an overdraft. (v) State the main distinction between a credit sale agreement and trade credit. (vi) What are the advantages and disadvantages of invoice discounting and non- recourse factoring from the point of view of the supplier? (vii) Describe how a company raises finance using: (a) bills of exchange (b) sterling commercial paper and indicate the most important differences between these two financial instruments















