Answer all those that have an X or that haven't been answered yet
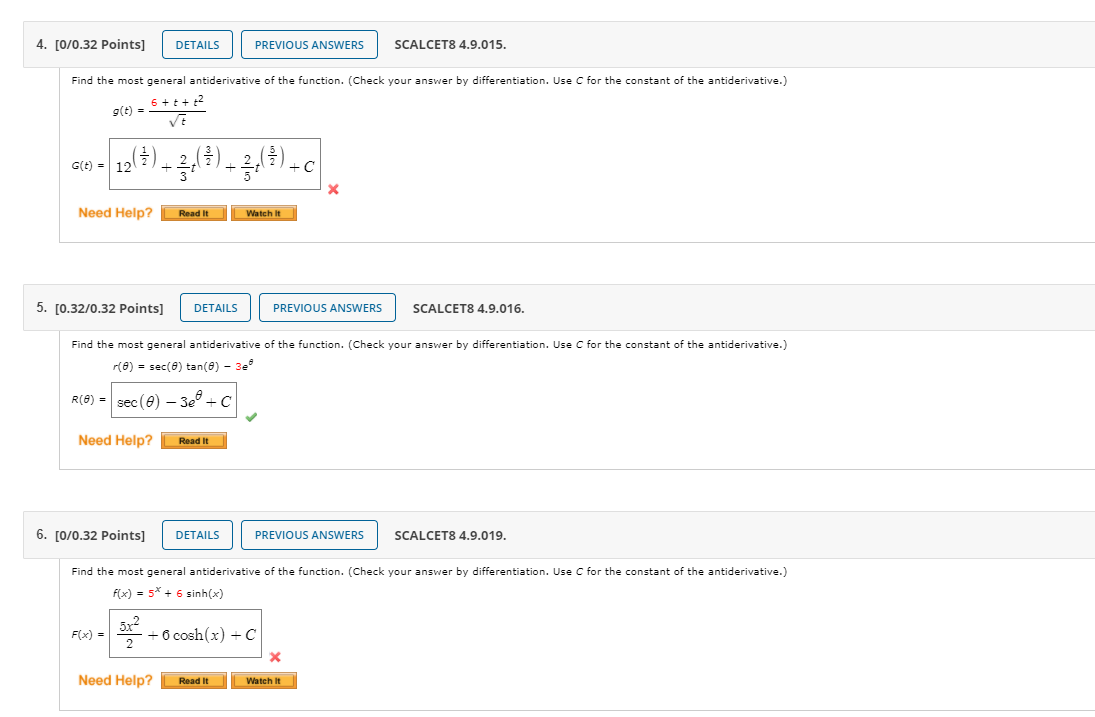
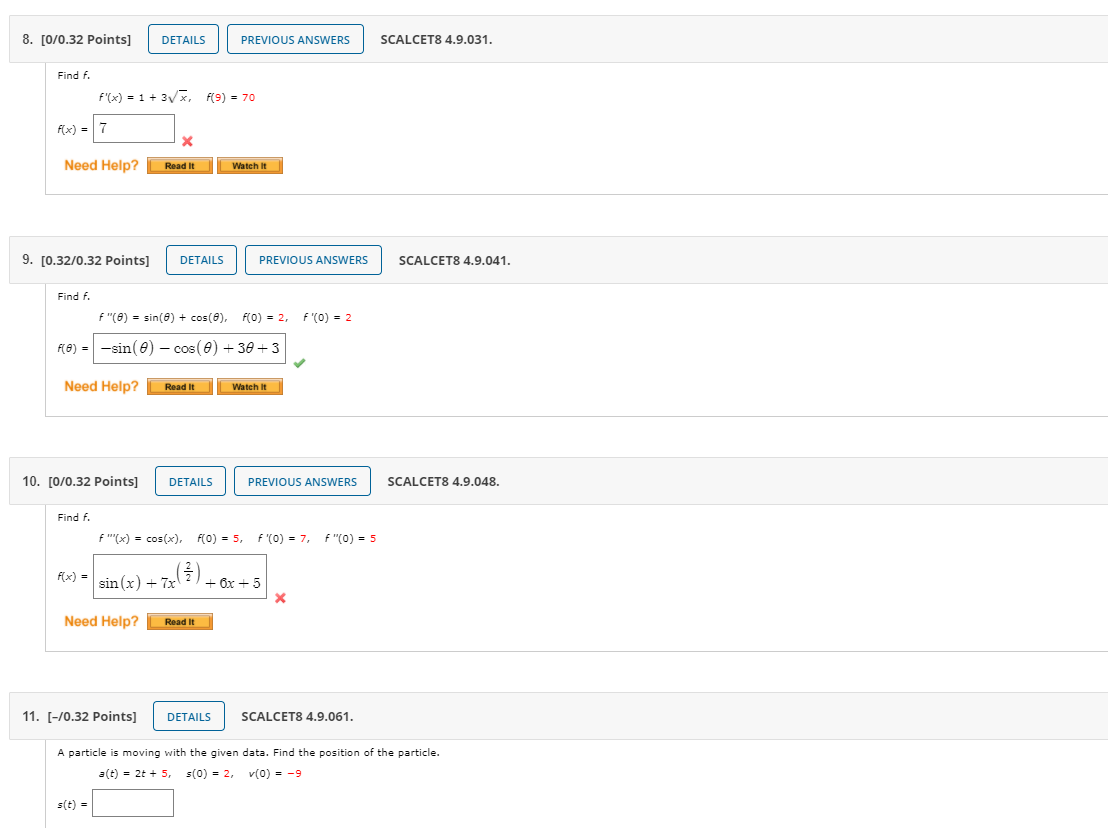
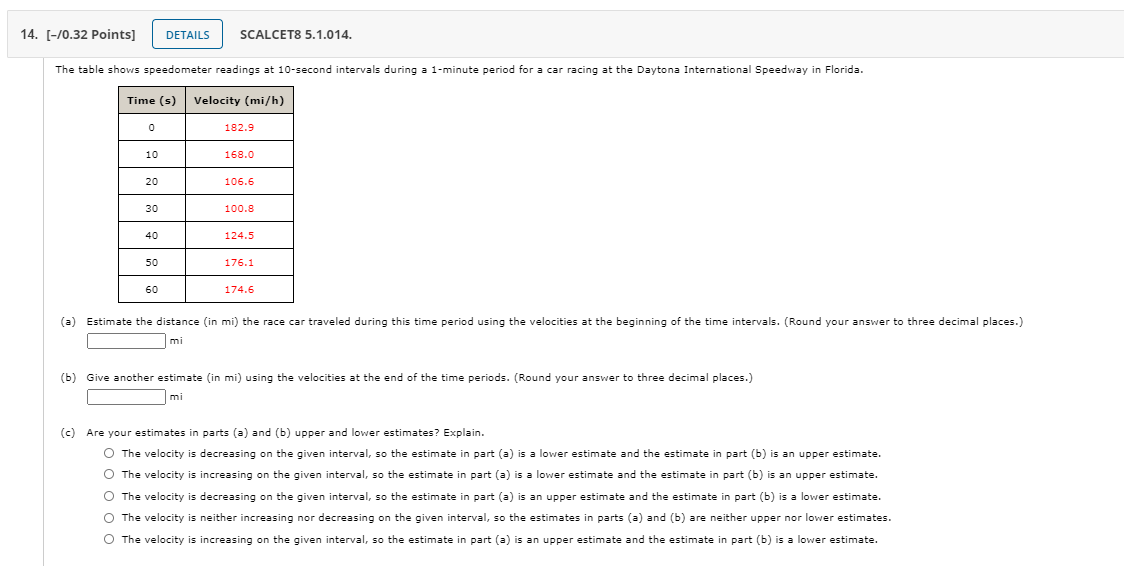
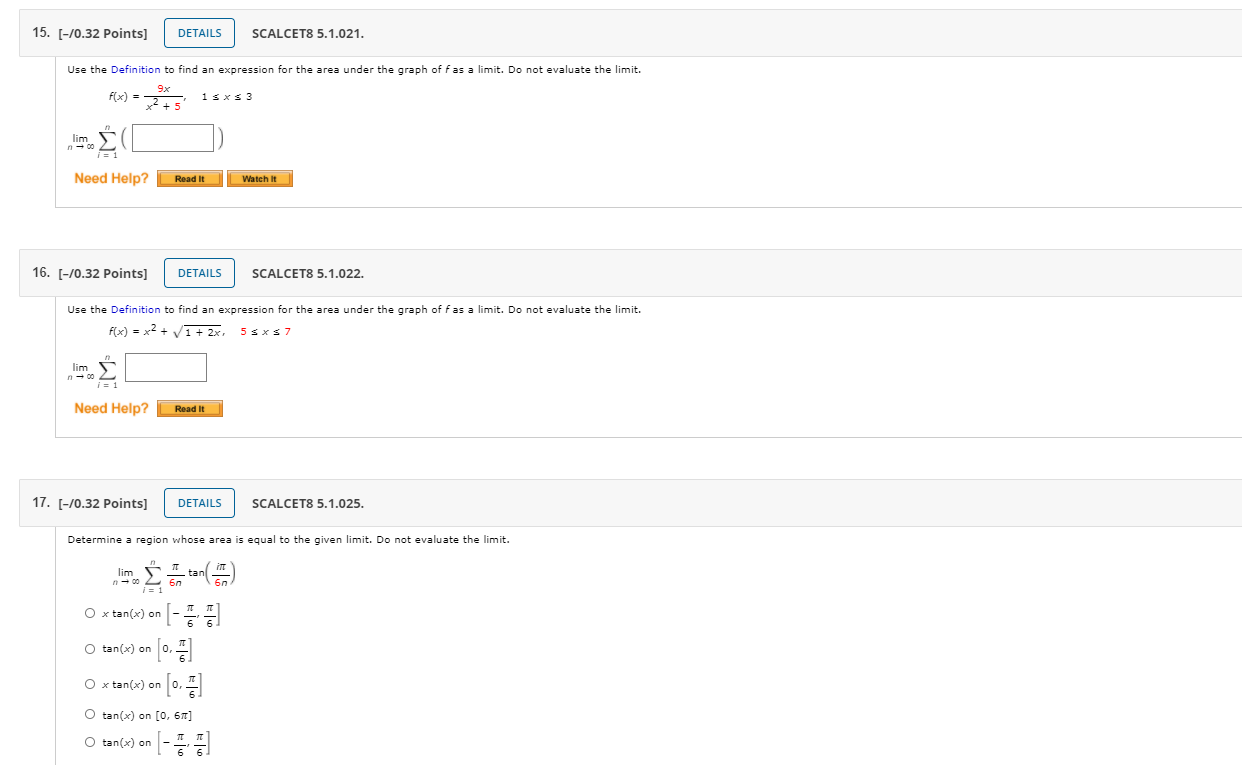
4. [0/0.32 Points] DETAILS PREVIOUS ANSWERS SCALCET8 4.9.015. Find the most general antiderivative of the function. (Check your answer by differentiation. Use C for the constant of the antiderivative.) g(t) = _ 6+t+ +2 Vt G(t) = 12 2, 2 5 C Need Help? Read It Watch It 5. [0.32/0.32 Points] DETAILS PREVIOUS ANSWERS SCALCET8 4.9.016. Find the most general antiderivative of the function. (Check your answer by differentiation. Use C for the constant of the antiderivative.) r(8) = sec(0) tan(0) - 3e R(8) = sec (0) - 30" + C Need Help? Read It 6. [0/0.32 Points] DETAILS PREVIOUS ANSWERS SCALCET8 4.9.019. Find the most general antiderivative of the function. (Check your answer by differentiation. Use C for the constant of the antiderivative.) f(x) = 5* + 6 sinh(x) F(x) = 5x2 2 + 6 cosh (x) + C X Need Help? Read It Watch It8. [0/0.32 Points] DETAILS PREVIOUS ANSWERS SCALCET8 4.9.031. Find f. F'(x) = 1 + 3Vx, f(9) = 70 F( x ) = 7 Need Help? Read It Watch It 9. [0.32/0.32 Points] DETAILS PREVIOUS ANSWERS SCALCET8 4.9.041. Find f. f "(8) = sin(8) + cos(0), f(0) = 2, F'(0) = 2 F(8) = -sin(@) - cos(8) + 30+ 3 Need Help? Read It Watch it 10. [0/0.32 Points] DETAILS PREVIOUS ANSWERS SCALCET8 4.9.048. Find f. F "(x) = cos(x), f(0) = 5, F '(0) = 7, F"(0) = 5 f( x ) = sin (x) + 7x N/N + 6x + 5 Need Help? Read It 11. [-/0.32 Points] DETAILS SCALCET8 4.9.061. A particle is moving with the given data. Find the position of the particle. a(t) = 2t + 5, s(0) = 2, v(0) = -9 s (t) =14. [-/0.32 Points] DETAILS SCALCET8 5.1.014. The table shows speedometer readings at 10-second intervals during a 1-minute period for a car racing at the Daytona International Speedway in Florida. Time (s) Velocity (mi/h) 182.9 10 168.0 20 106.6 30 100.8 40 124.5 50 176.1 60 174.6 (a) Estimate the distance (in mi) the race car traveled during this time period using the velocities at the beginning of the time intervals. (Round your answer to three decimal places.) mi (b) Give another estimate (in mi) using the velocities at the end of the time periods. (Round your answer to three decimal places.) mi (c) Are your estimates in parts (a) and (b) upper and lower estimates? Explain. The velocity is decreasing on the given interval, so the estimate in part (a) is a lower estimate and the estimate in part (b) is an upper estimate. O The velocity is increasing on the given interval, so the estimate in part (2) is a lower estimate and the estimate in part (b) is an upper estimate. The velocity is decreasing on the given interval, so the estimate in part (a) is an upper estimate and the estimate in part (b) is a lower estimate. O The velocity is neither increasing nor decreasing on the given interval, so the estimates in parts (@) and (b) are neither upper nor lower estimates. O The velocity is increasing on the given interval, so the estimate in part (3) is an upper estimate and the estimate in part (b) is a lower estimate.15. [-/0.32 Points] DETAILS SCALCET8 5.1.021. Use the Definition to find an expression for the area under the graph of fas a limit. Do not evaluate the limit. f( x ) = - 9x isx= 3 -+ 00 Need Help? Read It Watch It 16. [-/0.32 Points] DETAILS SCALCET8 5.1.022. Use the Definition to find an expression for the area under the graph of fas a limit. Do not evaluate the limit. F ( x ) = x2 + \\1+ 2x, 55x$7 Need Help? Read It 17. [-/0.32 Points] DETAILS SCALCET8 5.1.025. Determine a region whose area is equal to the given limit. Do not evaluate the limit. lim 1 tan It 6n O x tan(x) on - - O tan(x) on 0,- O x tan(x) on 0, O tan(x) on [0, 6n] O tan(x) on - 1,18. [-/0.32 Points] DETAILS SCALCET8 5.1.505.XP. The area A of the region S that lies under the graph of the continuous function is the limit of the sum of the areas of approximationg rectangles. A = lim R, = lim [f(x, )Ax + f(x2)4x + . . . + f(x,)Ax] Use this definition to find an expression for the area under the graph of fas a limit. Do not evaluate the limit. f(x) = Vx, is x= 13 A = lim Need Help? Read It Watch it 19. [-/0.32 Points] DETAILS SCALCET8 5.1.506.XP. The area A of the region S that lies under the graph of the continuous function is the limit of the sum of the areas of approximationg rectangles. A = lim R, = lim [f(x,)Ax + f(x2)4x + . . . + f(x,)4x] Use this definition to find an expression for the area under the graph of fas a limit. Do not evaluate the limit. f(x) = In(x) 7 5 x = 10 A = lime Need Help? Read It 20. [-/0.32 Points] DETAILS SCALCET8 5.2.002. If F(x) = 2 cos(x) 0 s x = 37/4 evaluate the Riemann sum with n = 6, taking the sample points to be left endpoints. (Round your answer to six decimal places.)24. [-/0.32 Points] DETAILS SCALCET8 5.2.022. Use the form of the definition of the integral given in the theorem to evaluate the integral. 1 ( x2 - 4x + 5 ) dx Need Help? Read It 25. [-/0.32 Points] DETAILS SCALCET8 5.2.023. Use the form of the definition of the integral given in the theorem to evaluate the integral. 12 (7 * 2 + 7 * ) dx Need Help? Read It Watch it 26. [-/0.32 Points] DETAILS SCALCET8 5.2.029. Express the integral as a limit of Riemann sums. Do not evaluate the limit. Vat x 2 dx Need Help? Read It Watch it 27. [-/0.32 Points] DETAILS SCALCET8 5.2.047.MI. Write as a single integral in the form / F(x) dx. ['Axax + Axax Ax) dx F( x ) dx28. [-/0.32 Points] DETAILS SCALCET8 5.2.049. f f(x ) dx = 33 and /9(x ) dx = 12 , find [ 4f ( x ) + 6g ( *)] dix . Need Help? Read It Watch it 29. [-/0.32 Points] DETAILS SCALCET8 5.2.053. Each of the regions A, B, and C bounded by the graph of f and the x-axis has area 3. Find the value of [, [ A( x ) + 2x + 6 ] dx. A -2 0 C Need Help? Read It Watch it 30. [-/0.32 Points] DETAILS SCALCET8 5.2.073. Express the limit as a definite integral. [Hint: Consider A(x) = x2.] lim Si- dx Need Help? Read it Watch it Submit Answer 31. [-/0.4 Points] DETAILS SCALCET8 5.2.502.XP.MI. If f(x) = 5x" - 4x, 0 s x