Answer b, c, d

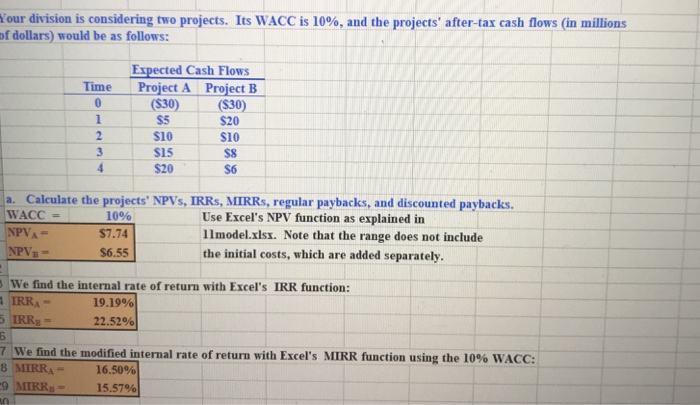
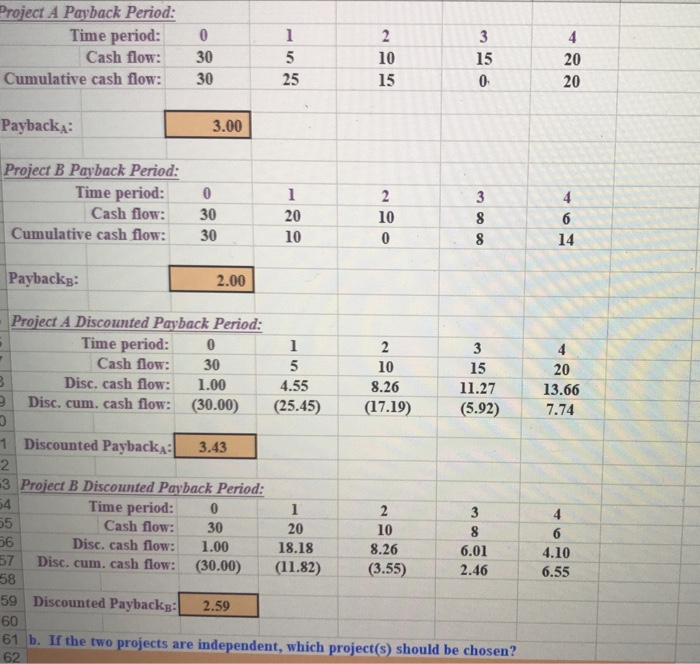
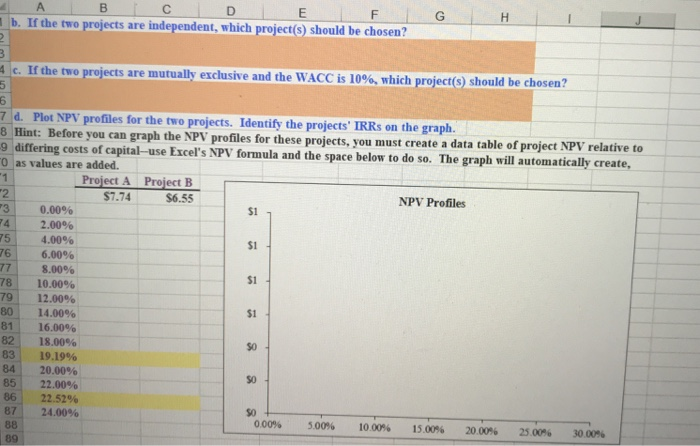
our division is considering two projects. Its WACC is 10%, and the projects after-tax cash flows (in millions of dollars) would be as follows: Expected Cash Flows Time Project A Project B 0 (S30) (S30) $20 S10 S8 S6 S5 $10 S15 $20 Calculate the projects' NPV, IRRs, MIRRs, regular paybacks, and discounted paybacks. a. Use Excel's NPV function as explained in llmodel.xlsx. Note that the range does not include the initial costs, which are added separately. 10% $7.74 $6.55 :we find the internal rate of return with Excel's IRR function: IRRA 19.19% 22.52% 7 we find the modified internal rate of return with Excel's MIRR function using the 10% WACC: 8 MIRRA- 16.50% 15.57% Project A Payback Period: Time period: Cash flow: Cumulative cash flow: 0 30 30 10 15 15 20 20 25 Paybacka: 3.00 Project B Payback Period: Time period: 0 Cash flow: 30 20 10 10 Cumulative cash flow: 30 Paybackg: Project A Discounted Payback Period: 14 2.00 Time period: 0 Cash flow: 30 1.00 10 8.26 15 11.27 20 13.66 Disc. cash flow: 4.55 9 Disc. cum. cash flow: (30.00 (25.45) (17.19) (5.92) 7.74 Discounted Paybackil 3.43 3 Project B Discounted Payback Period Time period: 0 Cash flow: 30 1.00 20 18.18 10 8.26 6 Disc. cash flow: 6.01 4.10 6.55 c. cum. cash flow: (30.00) (11.82) (3.55) 59 Discounted Payback:2.59 60 61 b. If the two projects are independent, which project(s) should be chosen? e two projects are independent, which project(S) should be chosen? c. If the two projects are mutually exclusive and the WACC is 10%, which project(s) should be chosen? 7 d. Plot NPV profiles for the two projects. Identify the projects' IRRs on the graph. 8 Hint: Before you can graph the NPV profiles for these projects, you must create a data table of project NPV relative to 9 differing costs of capital-use Excel's NPV formula and the space below to do so. The graph will automatically create, 0 as values are added. Project A Project B 7.74$6.55 NPV Profiles $1 31 0.00% Al 2.00% 75 4.00% 761 6.00% $1 8.00% $1 781 10.00% 79: 12.00% 801 14.00% 81 16.00% 821 18.00% 83 | 19.19% 841 20.00% 851 22.00% 86: 22.52% 87 | 24.00% 00096 50096 10.00% 15.0096 2000% 25 00% 3000%