Answer the following:

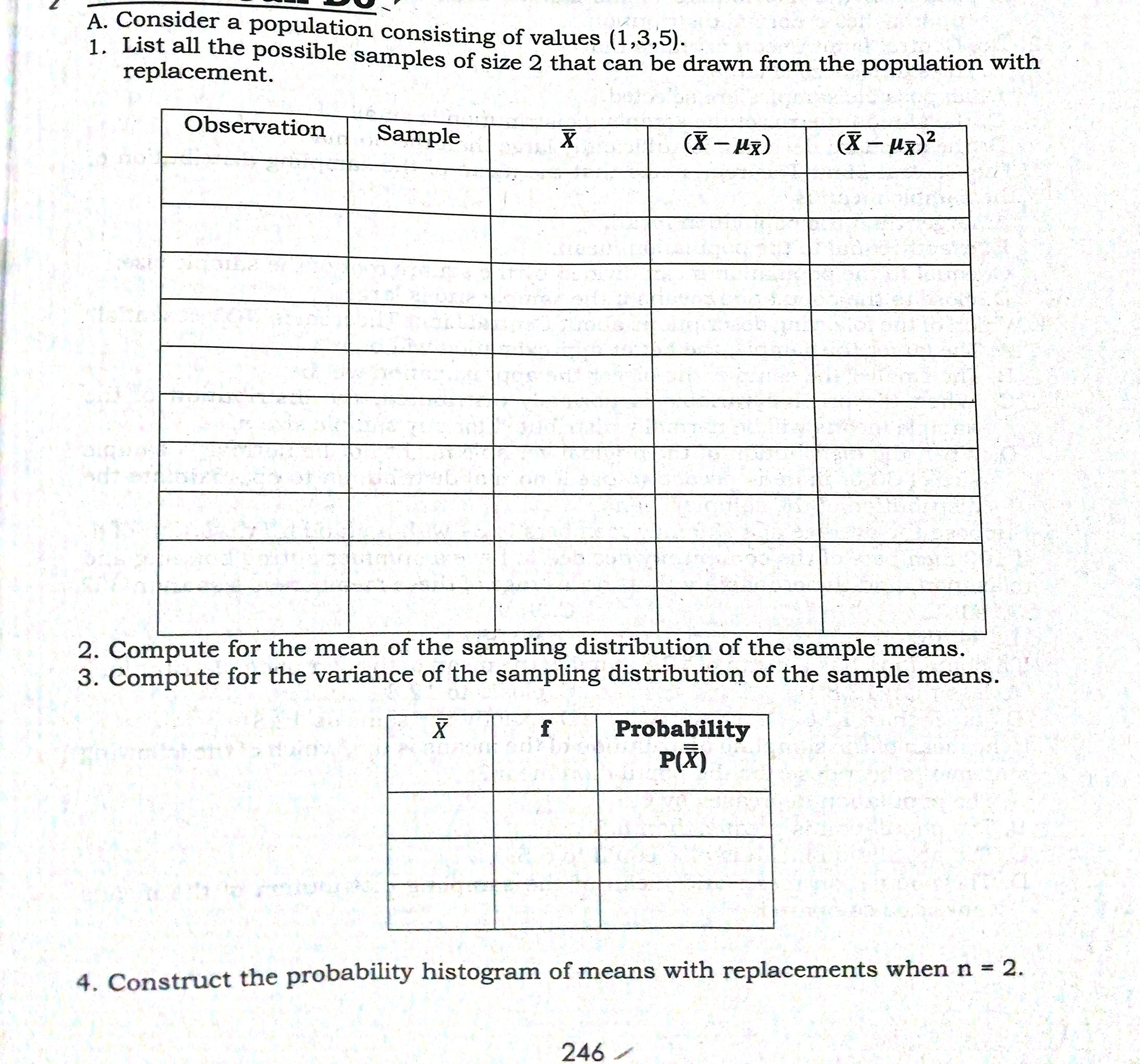
1. If the mean of the sampling distribution of the mean is 6.5, which of the following statements best describe the population mean? A. The population decreases by 6.5. B. The population is greater than 6.5. C. The population mean is also equal to 6.5. D. The population mean and mean of the sampling distribution of the means cannot be compared. 2. What does the Central Limit Theorem state? A. the sample size is large B. all possible samples are selected C. the standard error of the sampling distribution is small D. the standard deviation is sufficiently large than the normal 3. The Central Limit Theorem states that the mean of the sampling distribution of the sample mean is A. larger than the population mean. B. exactly equal to the population mean. C. close to the population means if the sample size is large. D. equal to the population mean divided by the square root of the sample size. 4. The states: "As the sample size n increases, the shape of the distribution of the sample means taken without replacement from a population with mean u and the standard deviation o will approach a normal distribution." A. Z distribution B. Central limit theorem C. chi distribution D. Pythagorean theorem 5. If a population has a mean of 12.8, what is the mean of the sampling distribution? A. less than 12.8 B. larger than 12.8 C. closer to 12.8 D. exactly the same as 12.86. As the sample size n increases, the standard deviation of the sampling distribution -. (2 points) A. Increases B. decreases C. stays the same D. not enough information is given 7. Which of the following descriptions does NOT illustrate the Central Limit Theorem? (2 points) A. The Central Limit Theorem is used to approximate the distribution of the sample mean over the distribution of the population mean. B. If the sample size n, where n is sufficiently large is drawn from any population with mean u and a standard deviation o, then the sampling distribution of sample means approximates a normal distribution. C. Whenever the population is not normally distributed, or if we do not know distribution, the Central Limit Theorem allows us to conclude that the distribution of sample means will be normal if the sample size is sufficiently large. D. Given a random variable X with mean u and variance o2, then regardless of whether the population distribution of X is normally distributed or not, the shape of the distribution of the sample means taken from the population approaches a normal distribution. 8. Which of the following statements is NOT true about Central Limit Theorem? (2 points) A. The population mean and the mean of the sampling distribution of the mean are equal. B. The variance of the sampling distribution of the mean and the population variance is exactly the same. C. The central limit theorem tells us exactly what the shape of the distribution of the mean will be when we draw repeated samples from a given population. D. If you take repeatedly independent random samples of size n from any population, then when n is large, the distribution of the sample mean will approach a normal distribution. 9. Consider the population consisting of values (2, 4, 6}. List all the possible samples of size 2 which can be drawn with replacement. (2 points) A. {2,4, 6} B. {(4, 2), (4, 4), (4, 6), (6,2), (6,4), (6,6) C. {(2,2), (2, 4), (2, 6) , (4, 2), (4, 4), (4, 6)} D. {(2,2), (2,4), (2, 6), (4,2), (4, 4), (4, 6), (6,2), (6,4), (6,6)} 10. If the population has a variance of 4.6, what is the variance of the sampling distribution of its means if the sampling distribution was derived with sample size n = 2 and all possible samples are drawn with replacements? (2 points) A. 21.16 B. 19.22 C. 10.58 D. 2.3The Central Limit Theorem implies the following important ideas in statistics: 1. When the sample size tends to infinity (a very large sample) the distribution of the sample mean X will be distributed. 2. If the sample size tends to infinity, the sample mean ux the population mean j. 3. When the original variable is normally distributed, the distribution of the sample means will be _ distributed, for any sample size n. 4. When the distribution of the original variable might not be normal, a sample size of or more is needed to use a normal distribution to approximate the distribution of the sample means. The larger the sample, the better the approximation will be.".\\ \\ A. ConSIder a population c 1, L1st all the possible s replacement. onsisting of values (1,3,5). _ , samples of size 2 that can be drawn from the population with 2. Compute for the mean of the sampling distribution of the sample means. 3. Compute for the variance of the sampling distribution of the sample means. 4 ConstruCt the probability histogram of means with replacements when n = 2. 246 /