
Answer the following questions
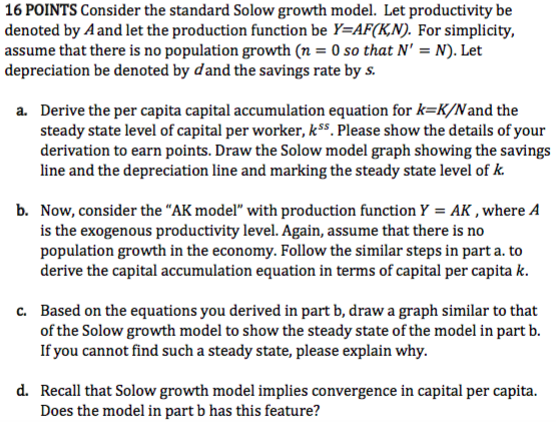
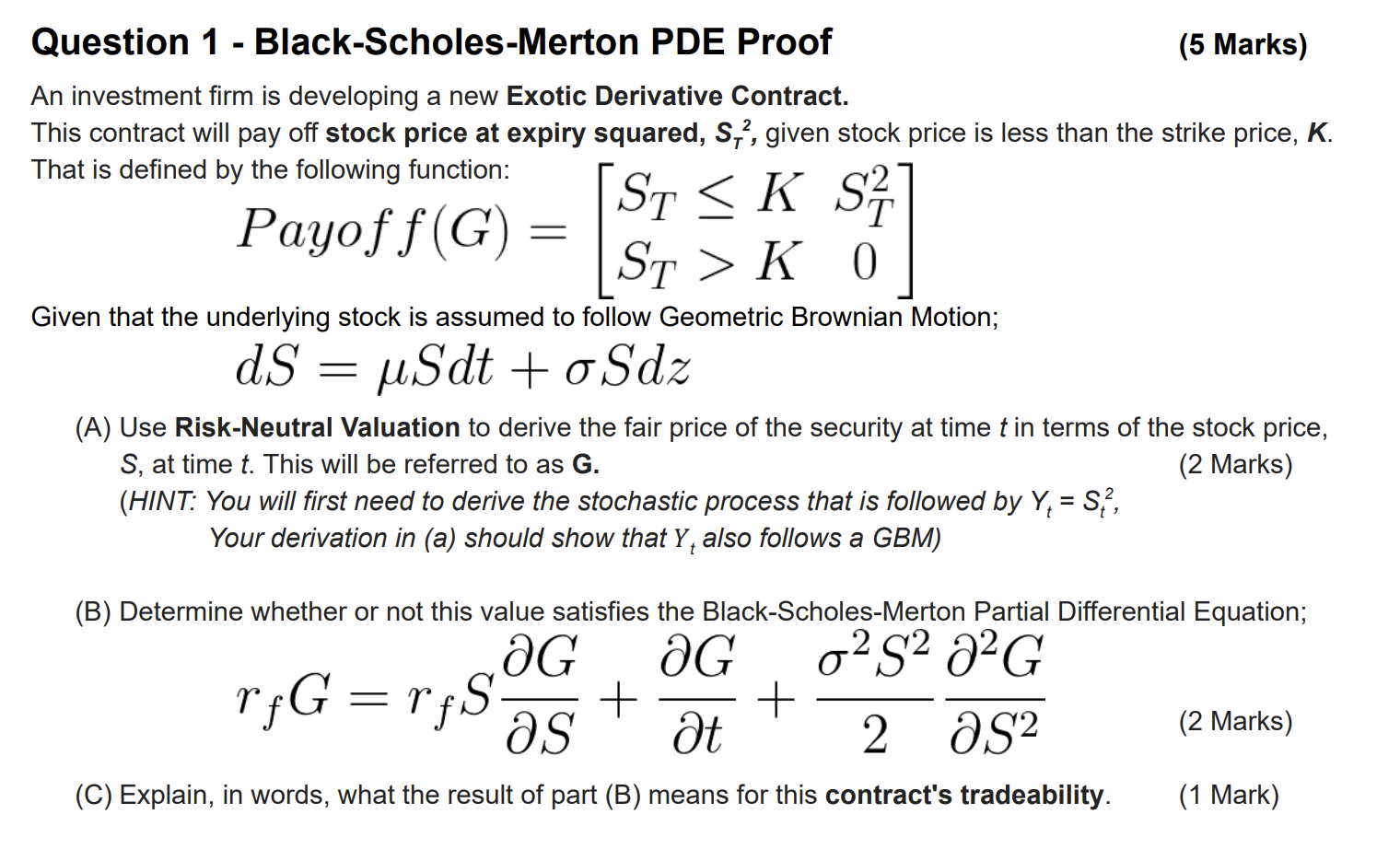
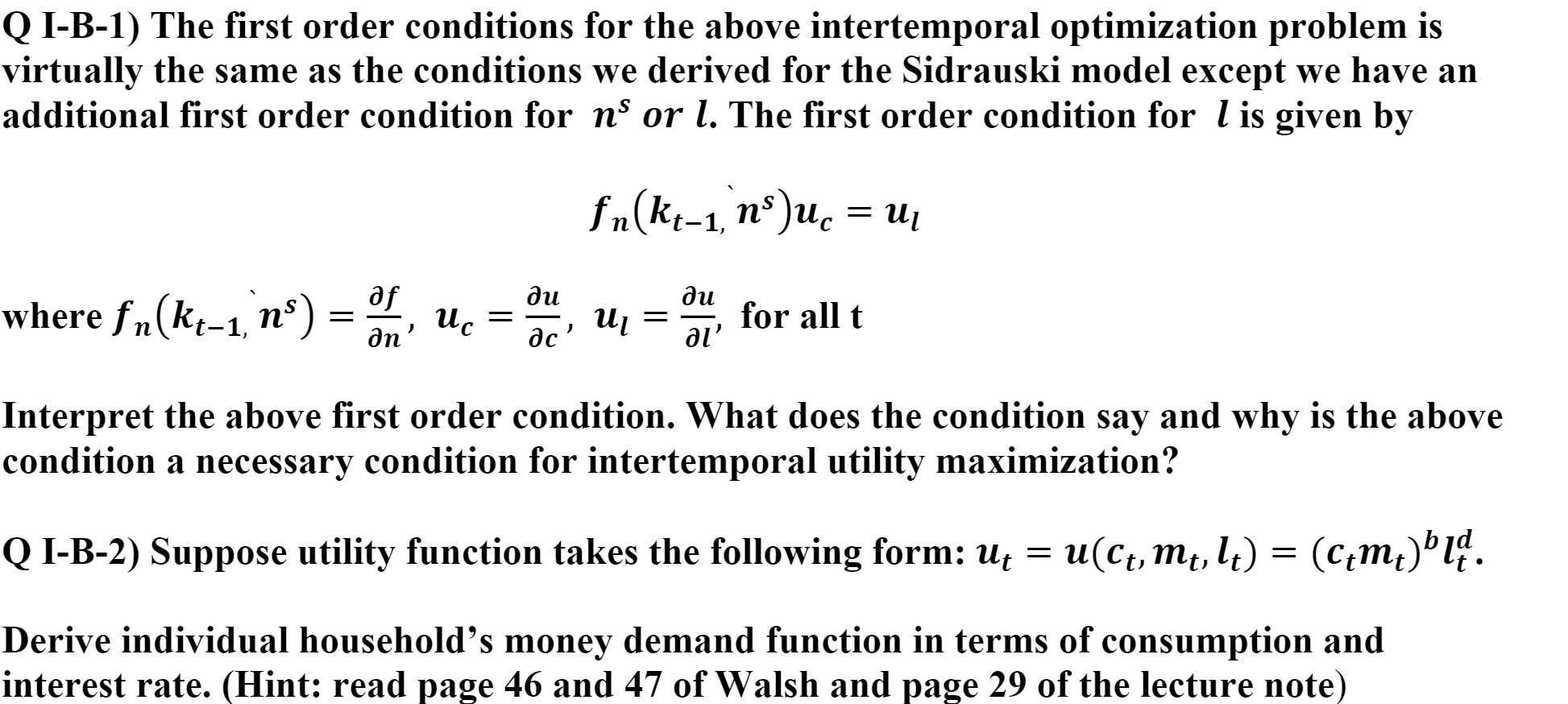
1. Suppose again that cereal is made from food storage items and labor, as in the previous problem. However, now Alex is only endowed with 120 hours of labor (no storage cans), and has utility Ua(ca,,,) = 25635. Bob is endowed only with E storage cans (but no labor call him a \"capitalist\" ), so his utility is Ub(cb) = cg'5. Bob also owns the cereal rm, and thus receives all of its prots. (a) (10 pts) Set up each household problem for Alex and Bob. (b) {5 pts) Production of cereal is given by F0(L, S) = LO'5SD'5. Set up the rm's problem. 0 If you fully solved the warmup, you may restate the answer to (b), (e), and (f) without showing the work again here. (c) (10 pts} Dene a. competitive equilibrium for this economy. Be sure to specify the market clearing condition for each market. ((1) (15 pts) Solve for Alex's demand for leisure, and for both Alex's and Bob's demand for cereal (in terms of input and output prices). a Note that Bob's problem is has no trade o's he will spend his whole budget on (33,. (e) {10 pts) Solve the rm's problem to obtain an expression that pins down the relative prices. (f) (5 pts) The previous question gives a prot-maximizing relationship between S and L. Use this to substitute for L the production function, and simplify to get the supply of C as a function input 8. Also, compute the prot earned, given S. (g) ( 5 pts) Solve for the equilibrium prices. 16 POINTS Consider the standard Solow growth model. Let productivity be denoted by A and let the production function be Y=AF(KN). For simplicity, assume that there is no population growth (n = 0 so that N' = N). Let depreciation be denoted by dand the savings rate by s. a. Derive the per capita capital accumulation equation for =K/Nand the steady state level of capital per worker, $s. Please show the details of your derivation to earn points. Draw the Solow model graph showing the savings line and the depreciation line and marking the steady state level of k. b. Now, consider the "AK model" with production function Y = AK , where A is the exogenous productivity level. Again, assume that there is no population growth in the economy. Follow the similar steps in part a. to derive the capital accumulation equation in terms of capital per capita k. c. Based on the equations you derived in part b, draw a graph similar to that of the Solow growth model to show the steady state of the model in part b. If you cannot find such a steady state, please explain why. d. Recall that Solow growth model implies convergence in capital per capita. Does the model in part b has this feature?Question 1 - Black-Scholes-Merton PDE Proof (5 Marks) An investment firm is developing a new Exotic Derivative Contract. This contract will pay off stock price at expiry squared, $72, given stock price is less than the strike price, K. That is defined by the following function: 2 $KS PayofG) 2 ST > K 0T Given that the underlying stock is assumed to follow Geometric Brownian Motion; d8 = uSdt | OSdZ (A) Use Risk-Neutral Valuation to derive the fair price of the security at time tin terms of the stock price, 8, at time t. This will be referred to as G. (2 Marks) (HINT: You will rst need to derive the stochastic process that is followed by Y, = Sf, Your derivation in (a) should show that Y, also follows a GBM) (B) Determine whether or not this value satisfies the Black-Scholes-Merton Partial Differential Equation; G 8 8G 80 02 82 (PG Tf _ Tf as + at 2 882 (2 Marks) (0) Explain, in words, what the result of part (B) means for this contract's tradeability. (1 Mark) Q I-B-1) The first order conditions for the above intertemporal optimization problem is virtually the same as the conditions we derived for the Sidrauski model except we have an additional first order condition for n's or l. The first order condition for I is given by fn(kt-1, ns )uc = ul where fn(kins) = ", up = du al for all t an ac W = Interpret the above first order condition. What does the condition say and why is the above condition a necessary condition for intertemporal utility maximization? Q I-B-2) Suppose utility function takes the following form: ut = u(Ct, mt, It) = (comt)bid. Derive individual household's money demand function in terms of consumption and interest rate. (Hint: read page 46 and 47 of Walsh and page 29 of the lecture note)














