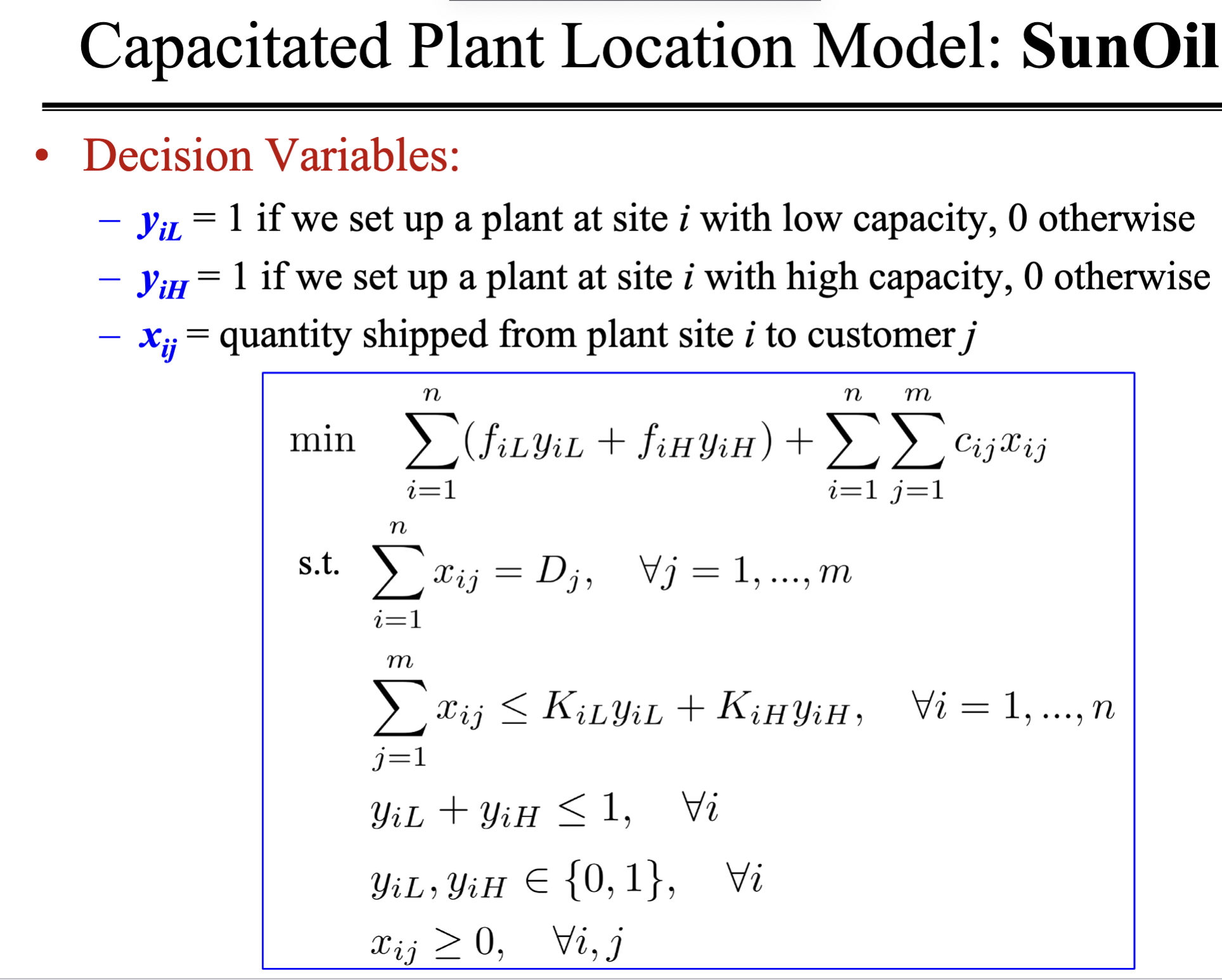
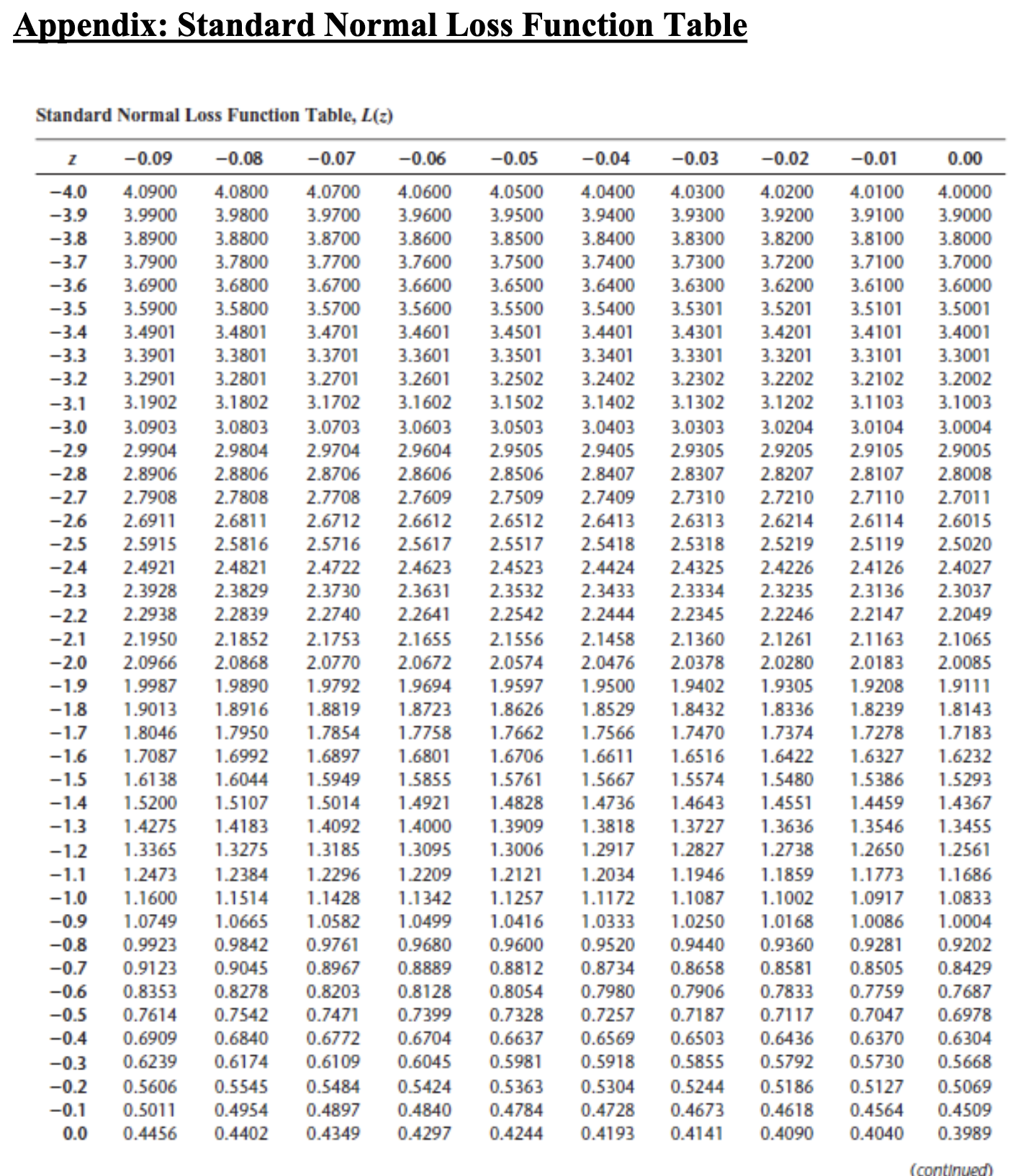
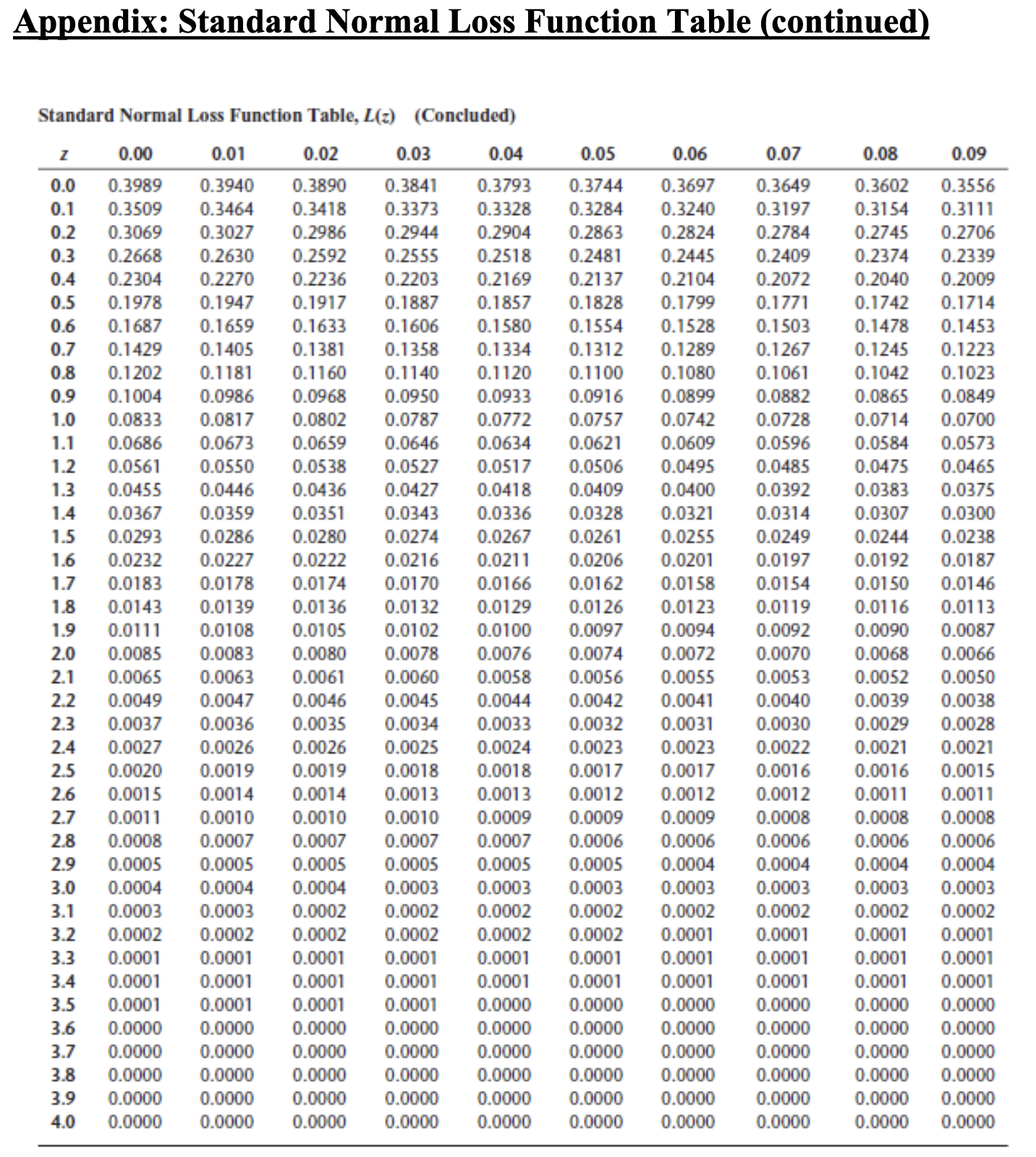
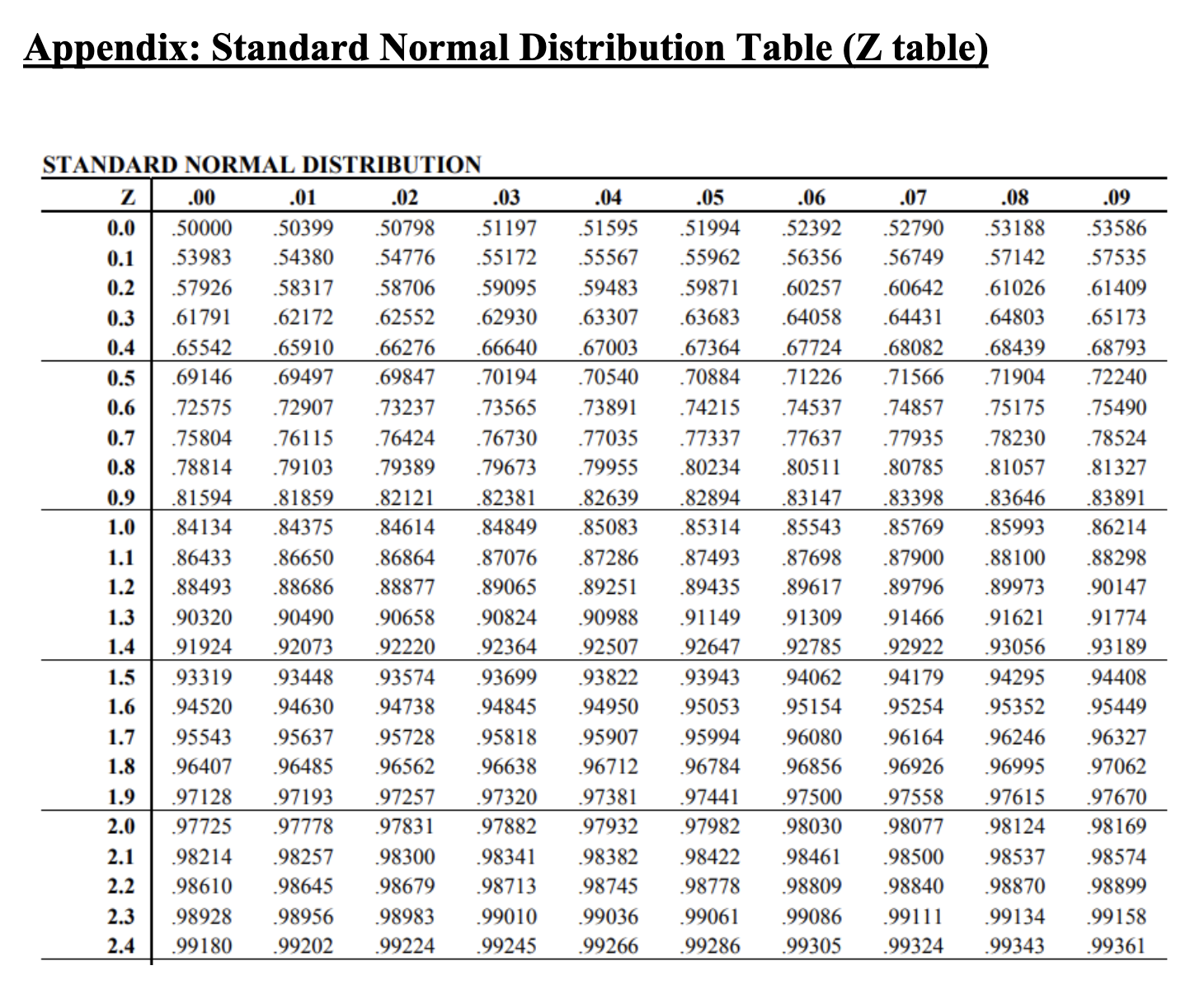
Appendix: Standard Normal Loss Function Table Appendix: Standard Normal Loss Function Table (continued) Standard Normal Loss Function Table, L(z) (Concluded) \begin{tabular}{|c|c|c|c|c|c|c|c|c|c|c|} \hlinez & 0.00 & 0.01 & 0.02 & 0.03 & 0.04 & 0.05 & 0.06 & 0.07 & 0.08 & 0.09 \\ \hline 0.0 & 0.3989 & 0.3940 & 0.3890 & 0.3841 & 0.3793 & 0.3744 & 0.3697 & 0.3649 & 0.3602 & 0.3556 \\ \hline 0.1 & 0.3509 & 0.3464 & 0.3418 & 0.3373 & 0.3328 & 0.3284 & 0.3240 & 0.3197 & 0.3154 & 0.3111 \\ \hline 0.2 & 0.3069 & 0.3027 & 0.2986 & 0.2944 & 0.2904 & 0.2863 & 0.2824 & 0.2784 & 0.2745 & 0.2706 \\ \hline 0.3 & 0.2668 & 0.2630 & 0.2592 & 0.2555 & 0.2518 & 0.2481 & 0.2445 & 0.2409 & 0.2374 & 0.2339 \\ \hline 0.4 & 0.2304 & 0.2270 & 0.2236 & 0.2203 & 0.2169 & 0.2137 & 0.2104 & 0.2072 & 0.2040 & 0.2009 \\ \hline 0.5 & 0.1978 & 0.1947 & 0.1917 & 0.1887 & 0.1857 & 0.1828 & 0.1799 & 0.1771 & 0.1742 & 0.1714 \\ \hline 0.6 & 0.1687 & 0.1659 & 0.1633 & 0.1606 & 0.1580 & 0.1554 & 0.1528 & 0.1503 & 0.1478 & 0.1453 \\ \hline 0.7 & 0.1429 & 0.1405 & 0.1381 & 0.1358 & 0.1334 & 0.1312 & 0.1289 & 0.1267 & 0.1245 & 0.1223 \\ \hline 0.8 & 0.1202 & 0.1181 & 0.1160 & 0.1140 & 0.1120 & 0.1100 & 0.1080 & 0.1061 & 0.1042 & 0.1023 \\ \hline 0.9 & 0.1004 & 0.0986 & 0.0968 & 0.0950 & 0.0933 & 0.0916 & 0.0899 & 0.0882 & 0.0865 & 0.0849 \\ \hline 1.0 & 0.0833 & 0.0817 & 0.0802 & 0.0787 & 0.0772 & 0.0757 & 0.0742 & 0.0728 & 0.0714 & 0.0700 \\ \hline 1.1 & 0.0686 & 0.0673 & 0.0659 & 0.0646 & 0.0634 & 0.0621 & 0.0609 & 0.0596 & 0.0584 & 0.0573 \\ \hline 1.2 & 0.0561 & 0.0550 & 0.0538 & 0.0527 & 0.0517 & 0.0506 & 0.0495 & 0.0485 & 0.0475 & 0.0465 \\ \hline 1.3 & 0.0455 & 0.0446 & 0.0436 & 0.0427 & 0.0418 & 0.0409 & 0.0400 & 0.0392 & 0.0383 & 0.0375 \\ \hline 1.4 & 0.0367 & 0.0359 & 0.0351 & 0.0343 & 0.0336 & 0.0328 & 0.0321 & 0.0314 & 0.0307 & 0.0300 \\ \hline 1.5 & 0.0293 & 0.0286 & 0.0280 & 0.0274 & 0.0267 & 0.0261 & 0.0255 & 0.0249 & 0.0244 & 0.0238 \\ \hline 1.6 & 0.0232 & 0.0227 & 0.0222 & 0.0216 & 0.0211 & 0.0206 & 0.0201 & 0.0197 & 0.0192 & 0.0187 \\ \hline 1.7 & 0.0183 & 0.0178 & 0.0174 & 0.0170 & 0.0166 & 0.0162 & 0.0158 & 0.0154 & 0.0150 & 0.0146 \\ \hline 1.8 & 0.0143 & 0.0139 & 0.0136 & 0.0132 & 0.0129 & 0.0126 & 0.0123 & 0.0119 & 0.0116 & 0.0113 \\ \hline 1.9 & 0.0111 & 0.0108 & 0.0105 & 0.0102 & 0.0100 & 0.0097 & 0.0094 & 0.0092 & 0.0090 & 0.0087 \\ \hline 2.0 & 0.0085 & 0.0083 & 0.0080 & 0.0078 & 0.0076 & 0.0074 & 0.0072 & 0.0070 & 0.0068 & 0.0066 \\ \hline 2.1 & 0.0065 & 0.0063 & 0.0061 & 0.0060 & 0.0058 & 0.0056 & 0.0055 & 0.0053 & 0.0052 & 0.0050 \\ \hline 2.2 & 0.0049 & 0.0047 & 0.0046 & 0.0045 & 0.0044 & 0.0042 & 0.0041 & 0.0040 & 0.0039 & 0.0038 \\ \hline 2.3 & 0.0037 & 0.0036 & 0.0035 & 0.0034 & 0.0033 & 0.0032 & 0.0031 & 0.0030 & 0.0029 & 0.0028 \\ \hline 2.4 & 0.0027 & 0.0026 & 0.0026 & 0.0025 & 0.0024 & 0.0023 & 0.0023 & 0.0022 & 0.0021 & 0.0021 \\ \hline 2.5 & 0.0020 & 0.0019 & 0.0019 & 0.0018 & 0.0018 & 0.0017 & 0.0017 & 0.0016 & 0.0016 & 0.0015 \\ \hline 2.6 & 0.0015 & 0.0014 & 0.0014 & 0.0013 & 0.0013 & 0.0012 & 0.0012 & 0.0012 & 0.0011 & 0.0011 \\ \hline 2.7 & 0.0011 & 0.0010 & 0.0010 & 0.0010 & 0.0009 & 0.0009 & 0.0009 & 0.0008 & 0.0008 & 0.0008 \\ \hline 2.8 & 0.0008 & 0.0007 & 0.0007 & 0.0007 & 0.0007 & 0.0006 & 0.0006 & 0.0006 & 0.0006 & 0.0006 \\ \hline 2.9 & 0.0005 & 0.0005 & 0.0005 & 0.0005 & 0.0005 & 0.0005 & 0.0004 & 0.0004 & 0.0004 & 0.0004 \\ \hline 3.0 & 0.0004 & 0.0004 & 0.0004 & 0.0003 & 0.0003 & 0.0003 & 0.0003 & 0.0003 & 0.0003 & 0.0003 \\ \hline 3.1 & 0.0003 & 0.0003 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0002 \\ \hline 3.2 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0002 & 0.0001 & 0.0001 & 0.0001 & 0.0001 \\ \hline 3.3 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 \\ \hline 3.4 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0001 \\ \hline 3.5 & 0.0001 & 0.0001 & 0.0001 & 0.0001 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 \\ \hline 3.6 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 \\ \hline 3.7 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 \\ \hline 3.8 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 \\ \hline 3.9 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 \\ \hline 4.0 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 & 0.0000 \\ \hline \end{tabular} Capacitated Plant Location Model: SunOil Decision Variables: yiL=1 if we set up a plant at site i with low capacity, 0 otherwise yiH=1 if we set up a plant at site i with high capacity, 0 otherwise xij= quantity shipped from plant site i to customer j mini=1n(fiLyiL+fiHyiH)+i=1nj=1mcijxijs.t.i=1nxij=Dj,j=1,,mj=1mxijKiLyiL+KiHyiH,i=1,,nyiL+yiH1,iyiL,yiH{0,1},ixij0,i,j Question 2 (Network design optimization). [18 points] Refer to the complete SunOil Model (with decision variables xij,yiL, and yiH ) in Lecture 5 Slide (full version) slide 14. Now suppose we have an additional single sourcing requirement. That is, each consumer market can only be served by a single plant site. For example, if we decide that the European market demand will be fulfilled by the plant in Asia, then all the 14 million units demand in Europe must all come from the Asian plant and Europe cannot receive shipments from any other plant. Revise the SunOil model we discussed in class to reflect the above single sourcing constraint. Please make sure your model has linear objective function and linear constraints. Feel free to define new decision variables however you see fit. For notation consistency, use index i,j=1,2,3,4,5 to respectively represent supply sites (i) and demand regions (j) in N. America, S. America, Europe, Asia, and Africa. Appendix: Standard Normal Distribution Table ( Z table)











