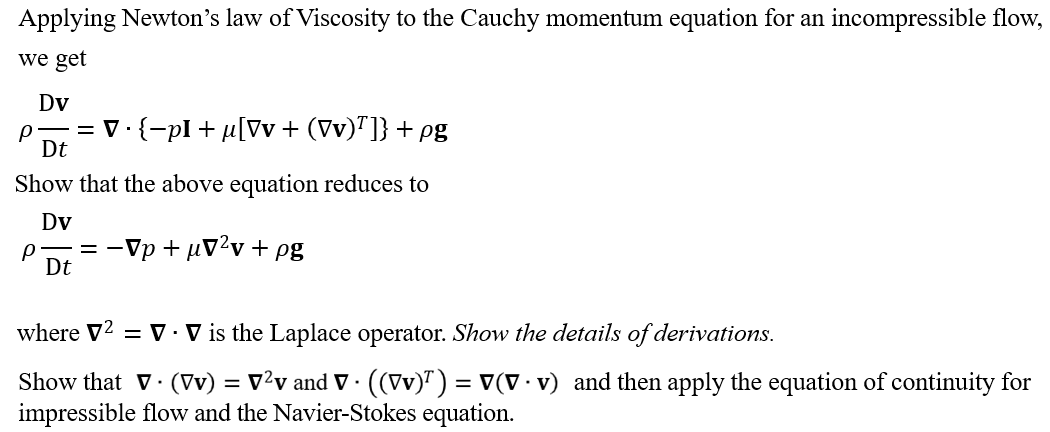
Applying Newton's law of Viscosity to the Cauchy momentum equation for an incompressible flow, we get Dv p== Dt {pI + [Vv + (Vv)]} + pg Show that the above equation reduces to Dv -p+Vv+pg Dt where V2 = V.V is the Laplace operator. Show the details of derivations. Show that V. (VV) = v and ((Vv)) = V(V v) and then apply the equation of continuity for impressible flow and the Navier-Stokes equation.