Answered step by step
Verified Expert Solution
Question
1 Approved Answer
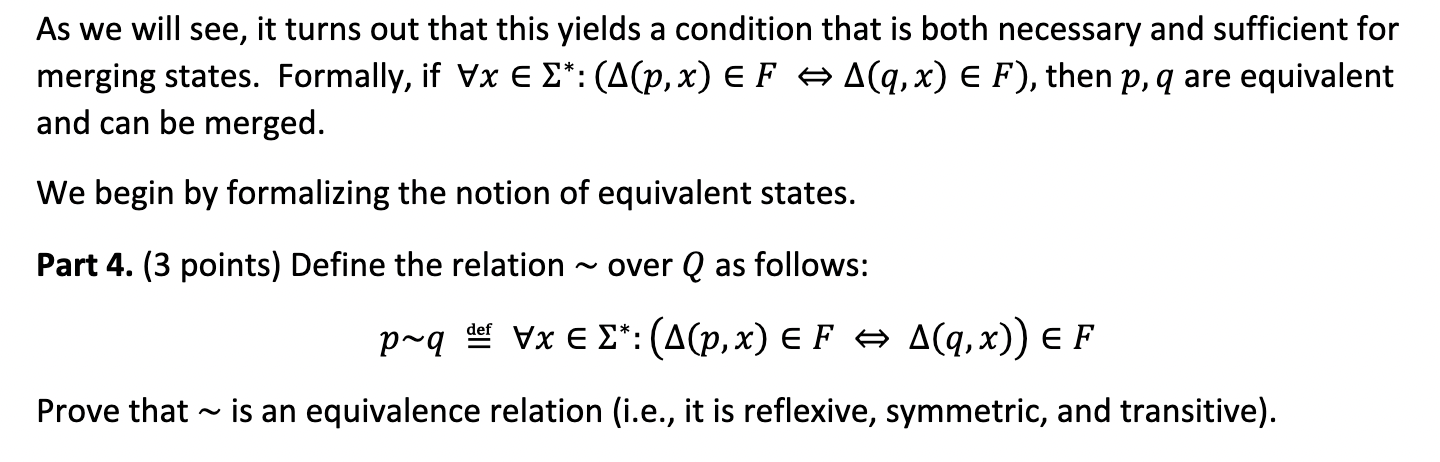
As we will see, it turns out that this yields a condition that is both necessary and sufficient for merging states. Formally, if :((,) (,)
As we will see, it turns out that this yields a condition that is both necessary and sufficient for merging states. Formally, if :((,) (,) ), then , are equivalent and can be merged. We begin by formalizing the notion of equivalent states. Define the relation ~ over as follows: ~ :A(,) (,)B Prove that ~ is an equivalence relation (i.e., it is reflexive, symmetric, and transitive).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


