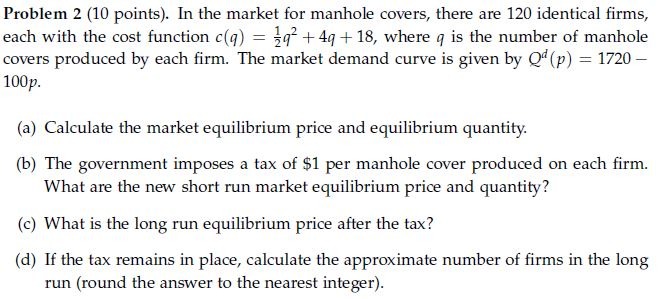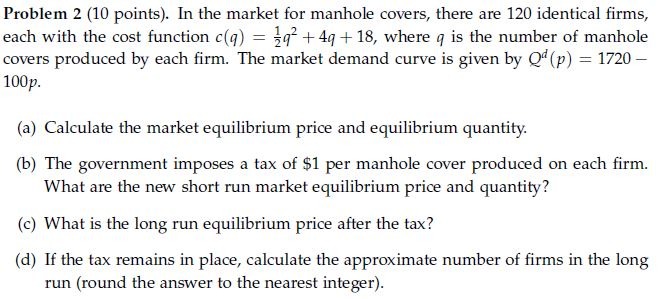
Assist please and give clear solutions that I can easily follow for easier understanding thank you. In the last question the table given is to help all the others are complete

Problem 4 Suppose all firms in a perfectly competitive market have identical long-run cost function given by: C(q) = q - 10q' + 36q where q is the output of the firm. Assume this is a constant cost industry. (a) What will be the long-run equilibrium price in this industry? What does the long-run market supply look like? (b) Suppose the market demand is given by QD = 111 - p where Qp is the market quantity demanded and p is the price. De- termine the long-run equilibrium market quantity. How many firms will there be in the long run?Problem 2 (10 points). In the market for manhole covers, there are 120 identical firms, each with the cost function c(q) = =q- + 4q + 18, where q is the number of manhole covers produced by each firm. The market demand curve is given by Q(p) = 1720 - 100p. (a) Calculate the market equilibrium price and equilibrium quantity. (b) The government imposes a tax of $1 per manhole cover produced on each firm. What are the new short run market equilibrium price and quantity? (c) What is the long run equilibrium price after the tax? (d) If the tax remains in place, calculate the approximate number of firms in the long run (round the answer to the nearest integer).Problem 3: Long-Run Equilibrium Suppose all firms in a perfectly competitive market have identical long-run cost function given by: C(q) = q3 - 10q2 + 36q where q is the output of the firm. Assume this is a constant cost industry. (a) What will be the long-run equilibrium price in this industry? What does the long-run market supply look like? (b) Suppose the market demand is given by QD = 111 -p where Qp is the market quantity demanded and p is the price. De- termine the long-run equilibrium market quantity. How many firms will there be in the long run?call there is one purestrategy Nash equilibrium, [3, In), despite Pareto improving outcomes if neither 3 nor m are played. Player 2 k 1 n1 w mmm Played 1? means I mmm (a) Explain why there are no mixed strategy equilibria where one player selects a pure strategy while the other mixes between two or three strategies. (b) Is there a mixed strategy equilibrium where Player 1 mixes between w and y and Player 2 mixes between k and l? (o) Is there a mixed strategy equilibrium where Player 1 only mixes between w and 2? Is there a mixed strategy equilibrium where Player 1 only mixes between y and s? (d) Is there a mixed strategy equilibrium where both players mix between all three strategies? (e) For any mixed strategy equilibrium found: is the equilibrium better or worse for the players than the pure strategy equilibrium of (am)? Explain why or why not