Attempt ONLY d AND e

Feedback: . In part (d), each moment should have included all the particles (both the 50-100 m range and the 100-500 m range). In part (e), the most common mistake was to assume all particles had the same size which is not appropriate for this question as you have the differential frequency distribution. With knowledge of the differential frequency distribution, the integral expression for total surface area should be used for a given mass.

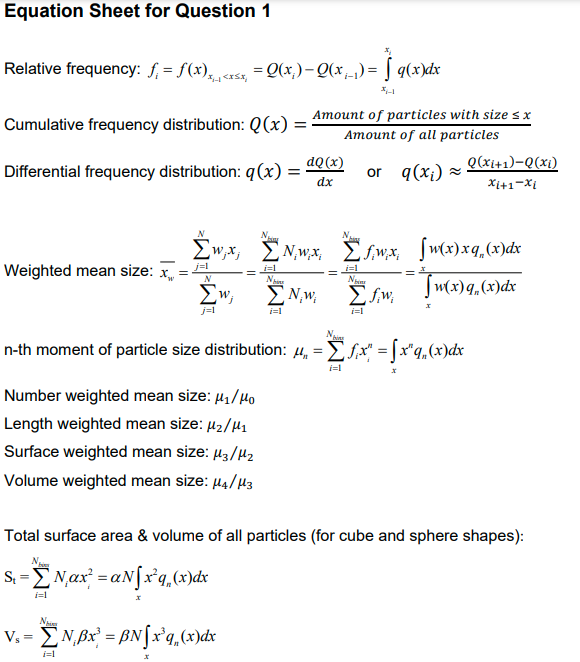
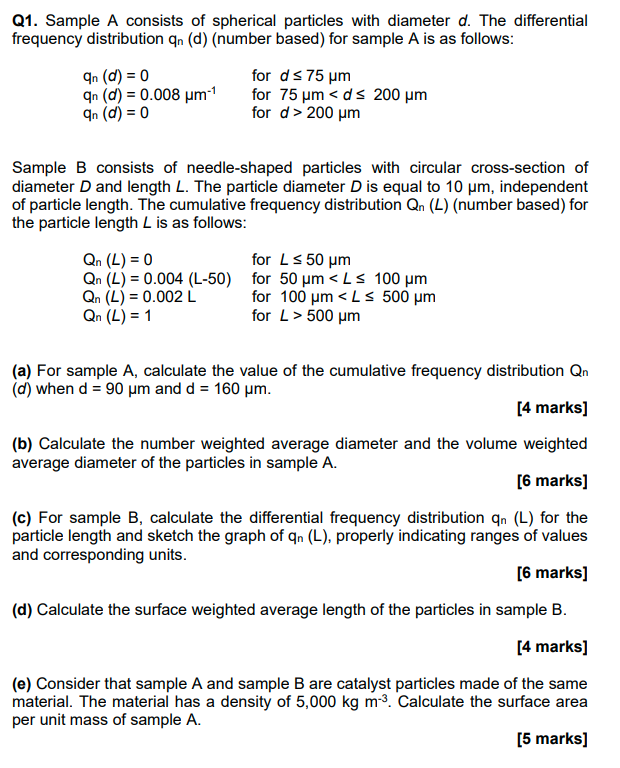
Equation Sheet for Question 1 Relative frequency: f;= f(x) y s, = Q(x,)- Q(x,-1) = 5 g(x)dx Cumulative frequency distribution: Q(x) Amount of particles with size s x Amount of all particles dQ(x) Differential frequency distribution: 7(x) = or 9(xi) Q(xi+1)-Q(xi) dx Xi+1-Xi N w.x, Nwx, Weighted mean size: x j=! = N i=1 N Ef.w.x w(x)xqy(x)dx fw, u(x)q,()dx Now , , Nos = i=1 n-th moment of particle size distribution: 4. = {1*= [x"q(v)dx Number weighted mean size: M1/Mo Length weighted mean size: Mz/u1 Surface weighted mean size: uz/uz Volume weighted mean size: M4/M3 Total surface area & volume of all particles (for cube and sphere shapes): S = 3 Nax = aNx*q,(x)dx N i=1 N. Vs = EN,Bx? = BNxq_(x)dx = i=1 Q1. Sample A consists of spherical particles with diameter d. The differential frequency distribution qn (d) (number based) for sample A is as follows: 9n (d) = 0 for ds 75 um qn (d) = 0.008 um? for 75 um 200 pm Sample B consists of needle-shaped particles with circular cross-section of diameter D and length L. The particle diameter D is equal to 10 um, independent of particle length. The cumulative frequency distribution Qn (L) (number based) for the particle length L is as follows: Qn (L) = 0 for Ls 50 um Qn (L) = 0.004 (L-50) for 50 um 500 pm Qn (L) = 1 for L > 500 pm (a) For sample A, calculate the value of the cumulative frequency distribution Qn (d) when d = 90 pm and d = 160 um. [4 marks] (b) Calculate the number weighted average diameter and the volume weighted average diameter of the particles in sample A. [6 marks] (c) For sample B, calculate the differential frequency distribution qn (L) for the particle length and sketch the graph of an (L), properly indicating ranges of values and corresponding units. [6 marks] (d) Calculate the surface weighted average length of the particles in sample B. [4 marks] (e) Consider that sample A and sample B are catalyst particles made of the same material. The material has a density of 5,000 kg m3. Calculate the surface area per unit mass of sample A. [5 marks]