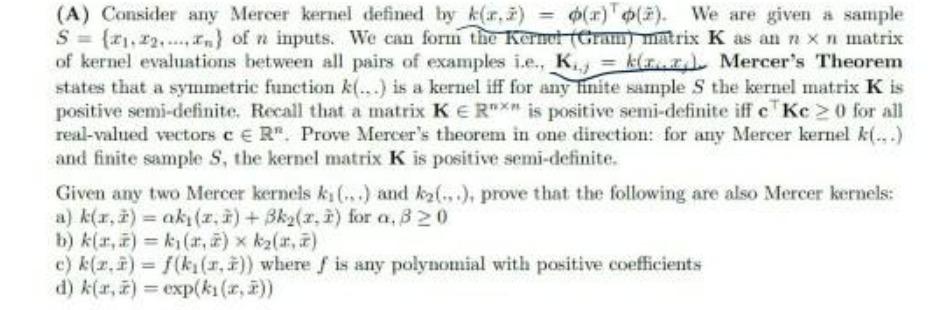
(A) Consider any Mercer kernel defined by k(r.) = o(r) (2). o(r) (2). We are given a sample S = (21.22.) of n inputs. We can form the Kernel (Gram) matrix K as an n x n matrix of kernel evaluations between all pairs of examples i.e., K., k. Mercer's Theorem states that a symmetric function k(...) is a kernel iff for any finite sample S the kernel matrix K is positive semi-definite. Recall that a matrix K E Rx" is positive semi-definite iff e Kc 20 for all real-valued vectors c E R. Prove Mercer's theorem in one direction: for any Mercer kernel k(...) and finite sample S, the kernel matrix K is positive semi-definite. Given any two Mercer kernels ki (...) and k(...), prove that the following are also Mercer kernels: a) k(r,i) = ak (z.)+3k(z.) for a, 320 b) k(,) = k(x, ) k(x,x) c) k(z.) = f(k (r. 2)) where f is any polynomial with positive coefficients d) k(x, z)= exp(ki(x,x))