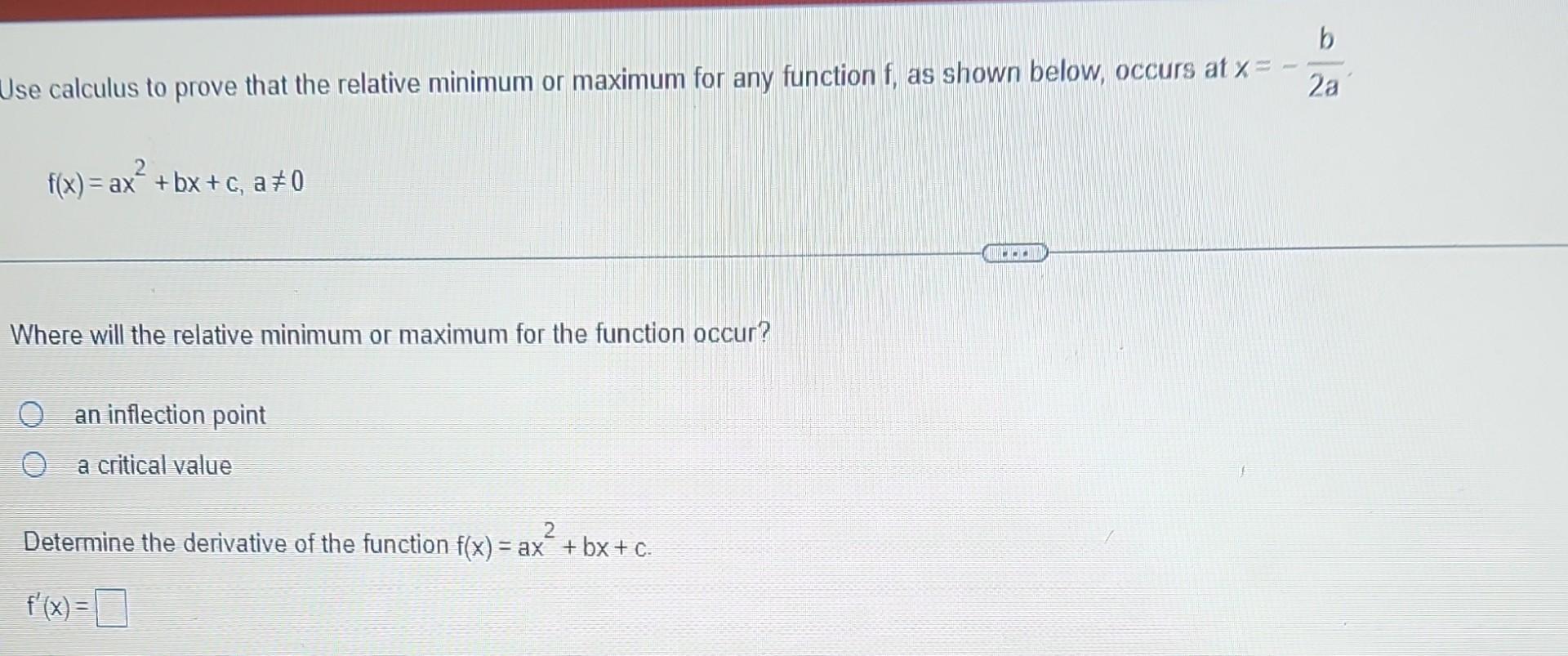
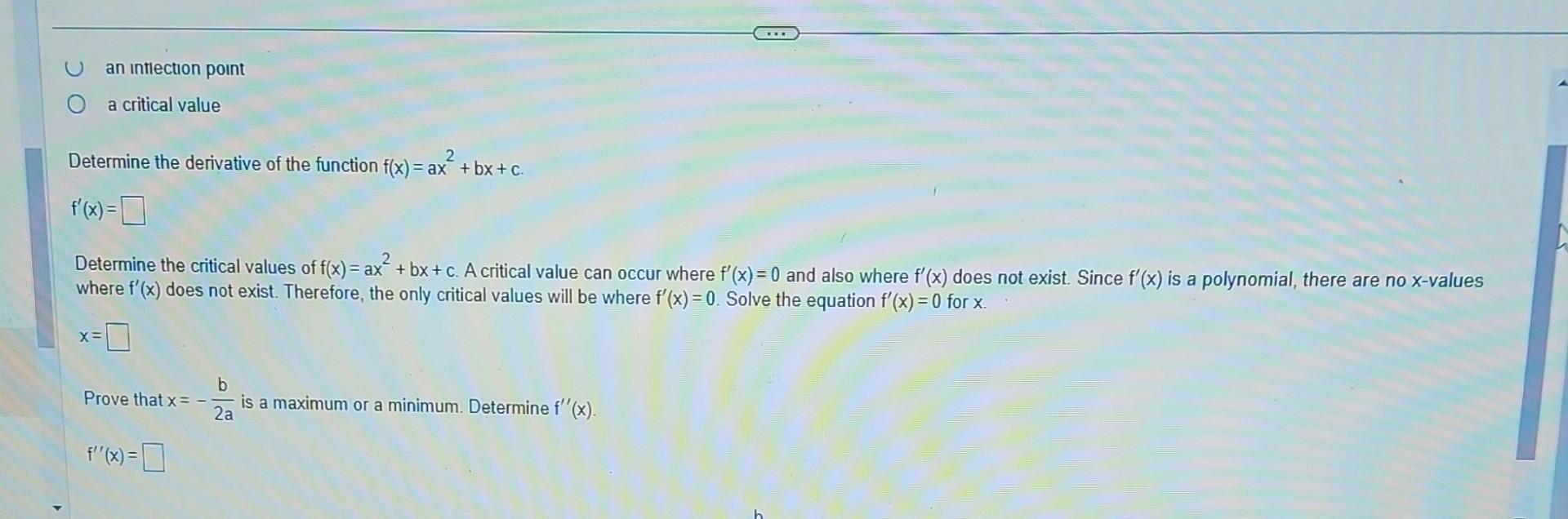
b Use calculus to prove that the relative minimum or maximum for any function f, as shown below, occurs at x = 2a 2 f(x) = ax +bx+c, a0 Where will the relative minimum or maximum for the function occur? an inflection point a critical value 2 Determine the derivative of the function f(x) = ax + bx+c. f'(x) = ... Determine the derivative of the function f(x) = ax + bx+c. f'(x) = Determine the critical values of f(x) = ax +bx+c. A critical value can occur where f'(x) = 0 and also where f'(x) does not exist. Since f'(x) is a polynomial, there are no x-values where f'(x) does not exist. Therefore, the only critical values will be where f'(x)=0. Solve the equation f'(x)=0 for x X= Prove that x= is a maximum or a minimum. Determine f''(x). 2a f''(x) = Since f''(x) = 2a is a constant, if a 0 and f is concave up for all x and x = - 2a 2a is a an inflection point a critical value Determine the derivative of the function f(x) = ax +bx+c. f'(x) = 0 Determine the critical values of f(x) = ax +bx+c. A critical value can occur where f'(x) = 0 and also where f'(x) does not exist. Since f'(x) is a polynomial, there are no x-values where f'(x) does not exist. Therefore, the only critical values will be where f'(x) = 0. Solve the equation f'(x) = 0 for x. X= b Prove that x = is a maximum or a minimum. Determine f''(x). 2a f''(x) =