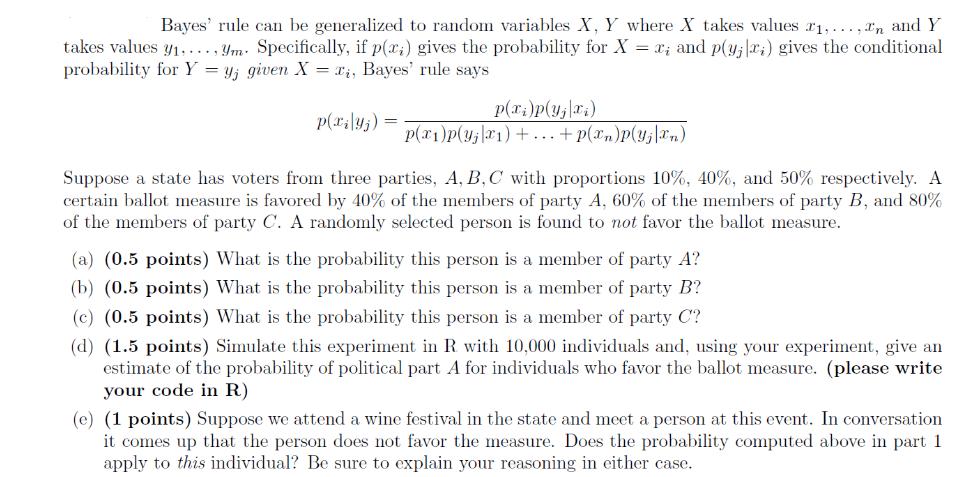
Bayes' rule can be generalized to random variables X, Y where X takes values 1,..., n and Y takes values y.... Ym. Specifically, if p(x,) gives the probability for X = x; and p(y;x;) gives the conditional probability for Y = y; given X = x, Bayes' rule says p(xi|yj) p(x)p(y;|xi) p(x1)p(y;1)+...+p(en)p(yjxn) Suppose a state has voters from three parties, A, B, C with proportions 10%, 40%, and 50% respectively. A certain ballot measure is favored by 40% of the members of party A, 60% of the members of party B, and 80% of the members of party C. A randomly selected person is found to not favor the ballot measure. (a) (0.5 points) What is the probability this person is a member of party A? (b) (0.5 points) What is the probability this person is a member of party B? (c) (0.5 points) What is the probability this person is a member of party C? (d) (1.5 points) Simulate this experiment in R. with 10,000 individuals and, using your experiment, give an estimate of the probability of political part A for individuals who favor the ballot measure. (please write your code in R) (e) (1 points) Suppose we attend a wine festival in the state and meet a person at this event. In conversation it comes up that the person does not favor the measure. Does the probability computed above in part 1 apply to this individual? Be sure to explain your reasoning in either case.