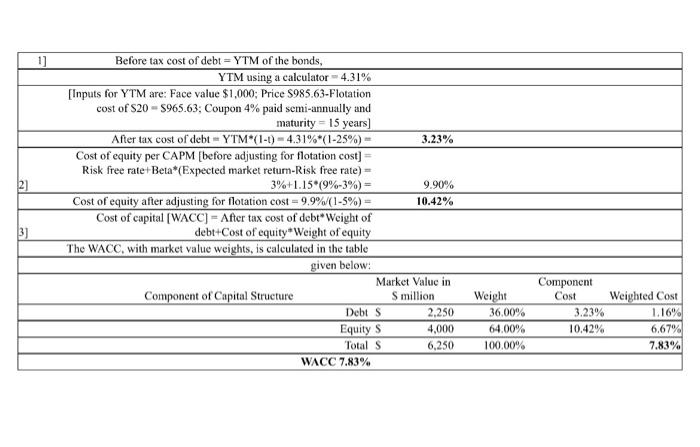
Behnke Golf has decided to sell a new line of golf clubs. The clubs will sell for $825 per set and have a variable cost of 77% of revenues per set. The company has spent $250,000 for a marketing study that determined the company will sell 90,000 sets per year for seven years. The company also plans to offer a line of golf balls, which are expected to sell for $45/dozen and have a variable cost of $15/dozen. The fixed costs each year will be $15,200,000. The company has also spent $1,000,000 on research and development for the new clubs. The plant and equipment required will cost $25,500,000 and will be depreciated using the MACRS seven- year schedule. The equipment will be sold for 150% of its book value in year 7. The new clubs will also require an increase in net working capital of $1,800,000 that will be returned at the end of the project. The tax rate is 25 percent. Information for computing the cost of capital is given in the previous problems. year schedule. The equipment will be sold for 150% of its book value in year 7. The new clubs will also require an increase in net working capital of $1,800,000 that will be returned at the end of the project. The tax rate is 25 percent. Information for computing the cost of capital is given in the previous problems. 4. Construct the proforma income statement for this project. 5. Calculate the NPV of the project. 6. Compute the IRR of the project. 7. Compute the profitability of the project. 1) Before tax cost of debt = YTM of the bonds, YTM using a calculator 4.31% [Inputs for YTM are: Face value $1,000; Price $985.63-Flotation cost of $20 - 5965.63; Coupon 4% paid semi-annually and maturity = 15 years) After tax cost of debt - YTM*(1-1) - 4.31%*(1-25%) - 3.23% Cost of equity per CAPM [before adjusting for flotation cost] Risk free rate+Beta*(Expected market return-Risk free rate) 3%+1.15*(9%-3%) = 9.90% Cost of equity after adjusting for flotation cost = 9.9%/(1-5%) = 10.42% Cost of capital (WACC) - After tax cost of debt*Weight of debt+Cost of equity*Weight of equity The WACC with market value weights, is calculated in the table given below: Market Value in Component of Capital Structure S million Debts 2.250 Equity s 4,000 Total s 6,250 WACC 7.83% 3) Weight 36,00% 64.00% 100.00% Component Cost Weighted Cost 3.23% 1.16% 10.42% 6,67% 7.83% Behnke Golf has decided to sell a new line of golf clubs. The clubs will sell for $825 per set and have a variable cost of 77% of revenues per set. The company has spent $250,000 for a marketing study that determined the company will sell 90,000 sets per year for seven years. The company also plans to offer a line of golf balls, which are expected to sell for $45/dozen and have a variable cost of $15/dozen. The fixed costs each year will be $15,200,000. The company has also spent $1,000,000 on research and development for the new clubs. The plant and equipment required will cost $25,500,000 and will be depreciated using the MACRS seven- year schedule. The equipment will be sold for 150% of its book value in year 7. The new clubs will also require an increase in net working capital of $1,800,000 that will be returned at the end of the project. The tax rate is 25 percent. Information for computing the cost of capital is given in the previous problems. year schedule. The equipment will be sold for 150% of its book value in year 7. The new clubs will also require an increase in net working capital of $1,800,000 that will be returned at the end of the project. The tax rate is 25 percent. Information for computing the cost of capital is given in the previous problems. 4. Construct the proforma income statement for this project. 5. Calculate the NPV of the project. 6. Compute the IRR of the project. 7. Compute the profitability of the project. 1) Before tax cost of debt = YTM of the bonds, YTM using a calculator 4.31% [Inputs for YTM are: Face value $1,000; Price $985.63-Flotation cost of $20 - 5965.63; Coupon 4% paid semi-annually and maturity = 15 years) After tax cost of debt - YTM*(1-1) - 4.31%*(1-25%) - 3.23% Cost of equity per CAPM [before adjusting for flotation cost] Risk free rate+Beta*(Expected market return-Risk free rate) 3%+1.15*(9%-3%) = 9.90% Cost of equity after adjusting for flotation cost = 9.9%/(1-5%) = 10.42% Cost of capital (WACC) - After tax cost of debt*Weight of debt+Cost of equity*Weight of equity The WACC with market value weights, is calculated in the table given below: Market Value in Component of Capital Structure S million Debts 2.250 Equity s 4,000 Total s 6,250 WACC 7.83% 3) Weight 36,00% 64.00% 100.00% Component Cost Weighted Cost 3.23% 1.16% 10.42% 6,67% 7.83%