Below are information about tensors.

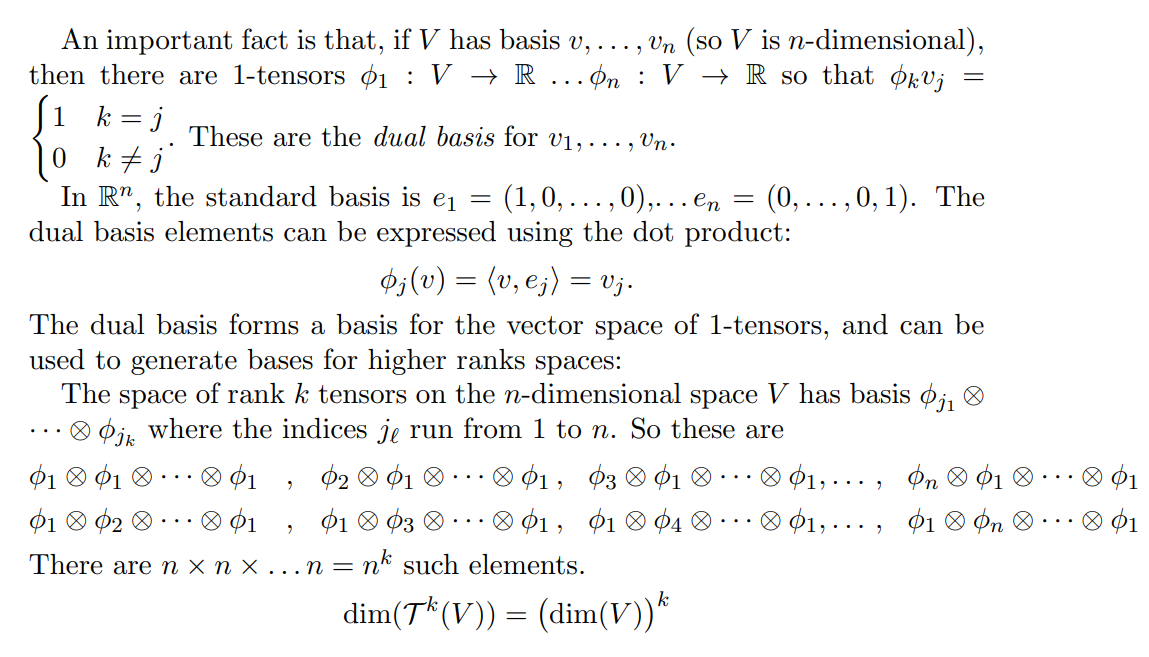
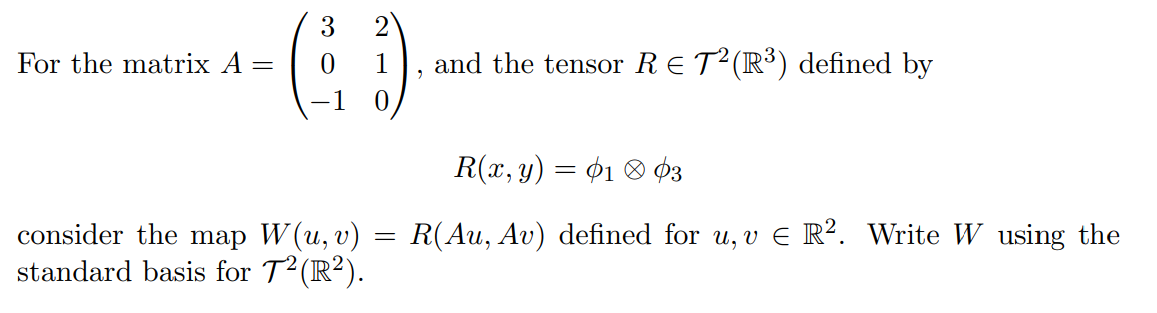
3 For the matrix A= 0 1 and the tensor RET?(R3) defined by 2 0 R(x, y) = 01 03 consider the map W(u, v) = R(Au, Av) defined for u, v E RP. Write W using the standard basis for T(R2). TENSORS For a vector space! V over the scalar field R, a k-tensor (or tensor of rank or order k) is a map T:Vk + R which is linear in each of its k entries. Examples: (1) any linear map L: V + R is a tensor of rank 1. These are sometimes called functionals. (2) an inner product (,): V x V + R is bilinear, so it is a rank 2 tensor. In particular, the usual "dot product on R is a 2-tensor on V =Rn. (3) if f : RM + R is k times differentiable, then, as we discussed last time, f(k)(x) is a k-tensor on V =RM. (4) the map DET which takes m vectors v1, ..., Vm in Rm (write them as column vectors) to the number U1 U2 DET(01,...,Um) = det : Um is an m-tensor. Here are a couple of elementary facts about k-tensors: if T, S: Vk + R are two k tensors, then the linear combination a +b : (aT + b)(v1, ..., UK) = aT(v1, ..., Uk) +bS(v1,..., UK) is also a k tensor. The set of k tensors over V forms a vector space. It is denoted Tk(V). If T: Vk R and S: Vm R are two tensors (possibly having different rank) then TS is a k + m tensor defined as TS(v1, ..., Vk, U1, ... , Um) = T(v1, ..., Uk). S(ui, ..., Um) Be careful! In general, TS is not the same as ST. Example Consider the tensor T : R3 x R3 R defined by T(u, v) = U3v1 + 20342, and the tensor S: R3 x R3 defined by S(u, v) = -U102 + V1U2. Then TOS: (R2)4 +R is ( TS)(u, v, w, z) = (u3v1 + 2v3u2)(-w122 + z1w2) and S T :(R2)4 R is (ST)(u, v, w, 2) = (-U102 + v1U2)(W321 +223w2) In particular, (TS) (C2, C3, C1, C2) is (2).(-1) = -2, while (ST)(C2,C3, C1, C1) is (0) (0) = 0. = O = An important fact is that, if V has basis v, ..., Vn (so V is n-dimensional), then there are 1-tensors 01 : V + R ... On : V + R so that OkV; 1 k=j These are the dual basis for vi,...,Un. k #j In R, the standard basis is ei = (1,0, ,0),... en (0,...,0,1). The dual basis elements can be expressed using the dot product: 0;(v) = (v, ej) = Vj. The dual basis forms a basis for the vector space of 1-tensors, and can be used to generate bases for higher ranks spaces: The space of rank k tensors on the n-dimensional space V has basis ji Ojk where the indices je run from 1 to n. So these are 01 01 & 8 01 02 01 ... 001, 03 01 001,..., On 01 01 01 02 0 001 01 03 01, 010 04 01, ..., 01 on ... 01 There are n xn x...n=nk such elements. dim(T*(V)) = (dim(V)) > k 3 For the matrix A= 0 1 and the tensor RET?(R3) defined by 2 0 R(x, y) = 01 03 consider the map W(u, v) = R(Au, Av) defined for u, v E RP. Write W using the standard basis for T(R2). TENSORS For a vector space! V over the scalar field R, a k-tensor (or tensor of rank or order k) is a map T:Vk + R which is linear in each of its k entries. Examples: (1) any linear map L: V + R is a tensor of rank 1. These are sometimes called functionals. (2) an inner product (,): V x V + R is bilinear, so it is a rank 2 tensor. In particular, the usual "dot product on R is a 2-tensor on V =Rn. (3) if f : RM + R is k times differentiable, then, as we discussed last time, f(k)(x) is a k-tensor on V =RM. (4) the map DET which takes m vectors v1, ..., Vm in Rm (write them as column vectors) to the number U1 U2 DET(01,...,Um) = det : Um is an m-tensor. Here are a couple of elementary facts about k-tensors: if T, S: Vk + R are two k tensors, then the linear combination a +b : (aT + b)(v1, ..., UK) = aT(v1, ..., Uk) +bS(v1,..., UK) is also a k tensor. The set of k tensors over V forms a vector space. It is denoted Tk(V). If T: Vk R and S: Vm R are two tensors (possibly having different rank) then TS is a k + m tensor defined as TS(v1, ..., Vk, U1, ... , Um) = T(v1, ..., Uk). S(ui, ..., Um) Be careful! In general, TS is not the same as ST. Example Consider the tensor T : R3 x R3 R defined by T(u, v) = U3v1 + 20342, and the tensor S: R3 x R3 defined by S(u, v) = -U102 + V1U2. Then TOS: (R2)4 +R is ( TS)(u, v, w, z) = (u3v1 + 2v3u2)(-w122 + z1w2) and S T :(R2)4 R is (ST)(u, v, w, 2) = (-U102 + v1U2)(W321 +223w2) In particular, (TS) (C2, C3, C1, C2) is (2).(-1) = -2, while (ST)(C2,C3, C1, C1) is (0) (0) = 0. = O = An important fact is that, if V has basis v, ..., Vn (so V is n-dimensional), then there are 1-tensors 01 : V + R ... On : V + R so that OkV; 1 k=j These are the dual basis for vi,...,Un. k #j In R, the standard basis is ei = (1,0, ,0),... en (0,...,0,1). The dual basis elements can be expressed using the dot product: 0;(v) = (v, ej) = Vj. The dual basis forms a basis for the vector space of 1-tensors, and can be used to generate bases for higher ranks spaces: The space of rank k tensors on the n-dimensional space V has basis ji Ojk where the indices je run from 1 to n. So these are 01 01 & 8 01 02 01 ... 001, 03 01 001,..., On 01 01 01 02 0 001 01 03 01, 010 04 01, ..., 01 on ... 01 There are n xn x...n=nk such elements. dim(T*(V)) = (dim(V)) > k









