Bob runs a snow cone stand, and customers go in and out by an M/M/1 queuing system model. Bob's average service time is 0.5 minutes for one customer. (You don't need the arrival rate to answer this question.)

Alice gets in line and is the 4th customer in line.

Due to the ongoing the friendly rivalry between Alice and Bob, Alice challenges Bob's service speed with the following wager:

If after 1 minutes, Alice is still in the system, then Alice wins.

But if Alice gets out before spending 1 minutes total in the system, then Bob wins.

What is the chance that Bob will win the wager? Give the probability in decimal form, rounded to 3 places.

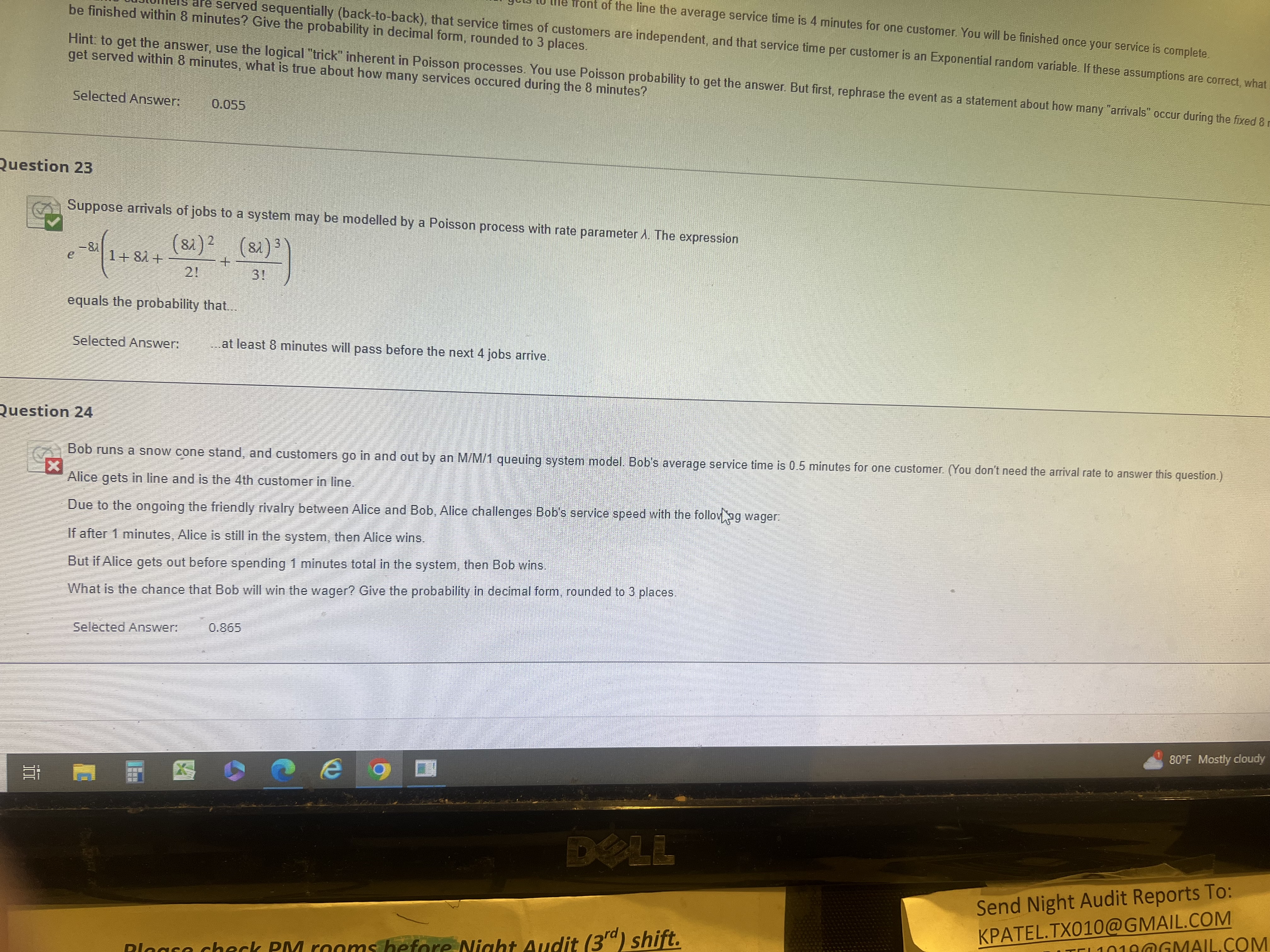int of the line the average service time is 4 minutes for one customer. You will be finished once your service is complete. are served sequentially (back-to-back), that service times of customers are independent, and that service time per customer is an Exponential random variable. If these assumptions are correct, what be finished within 8 minutes? Give the probability in decimal form, rounded to 3 places. Hint: to get the answer, use the logical "trick" inherent in Poisson processes. You use Poisson probability to get the answer. But first, rephrase the event as a statement about how many "arrivals" occur during the fixed & get served within 8 minutes, what is true about how many services occured during the 8 minutes? Selected Answer: 0.055 Question 23 Suppose arrivals of jobs to a system may be modelled by a Poisson process with rate parameter A. The expression - 81 1 + 81+ (81) 2 (81) 3 2! 3 ! equals the probability that... Selected Answer: at least 8 minutes will pass before the next 4 jobs arrive. Question 24 Bob runs a snow cone stand, and customers go in and out by an M/M/1 queuing system model. Bob's average service time is 0.5 minutes for one customer. (You don't need the arrival rate to answer this question.) X Alice gets in line and is the 4th customer in line. Due to the ongoing the friendly rivalry between Alice and Bob, Alice challenges Bob's service speed with the followag wager: If after 1 minutes, Alice is still in the system, then Alice wins. But if Alice gets out before spending 1 minutes total in the system, then Bob wins What is the chance that Bob will win the wager? Give the probability in decimal form, rounded to 3 places. Selected Answer: 0.865 80 F Mostly cloudy DELL Send Night Audit Reports To: KPATEL. TX010@GMAIL.COM ALL.COM udit (3rd) shift