Box 15.2:
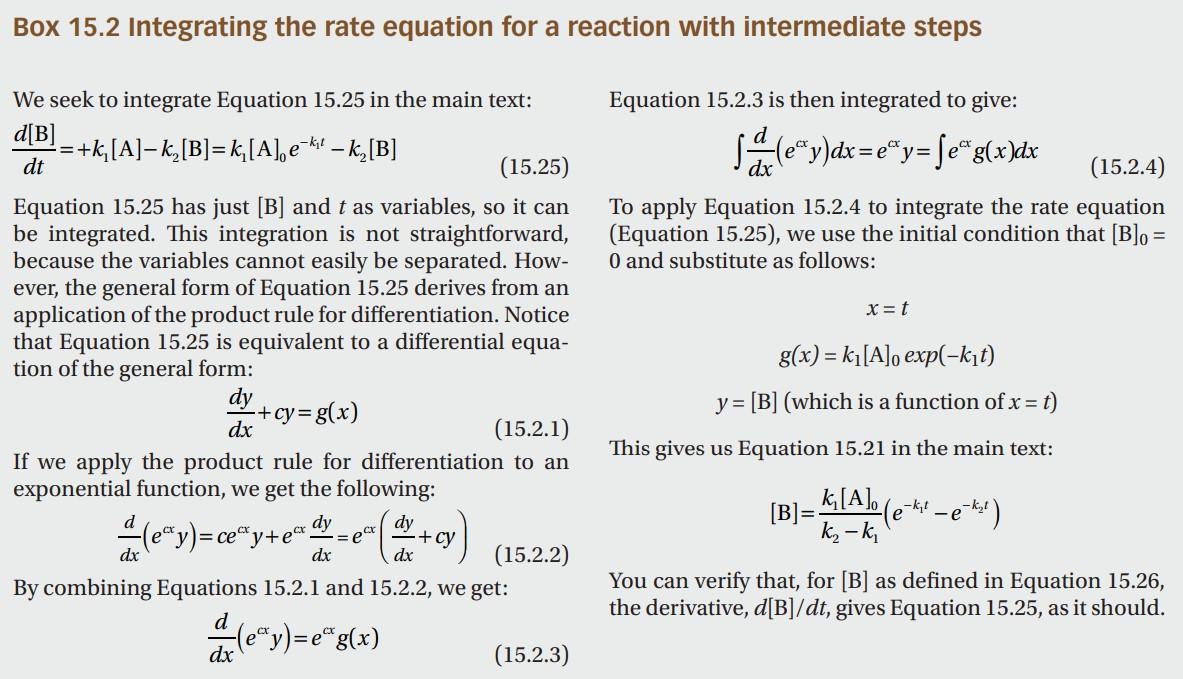
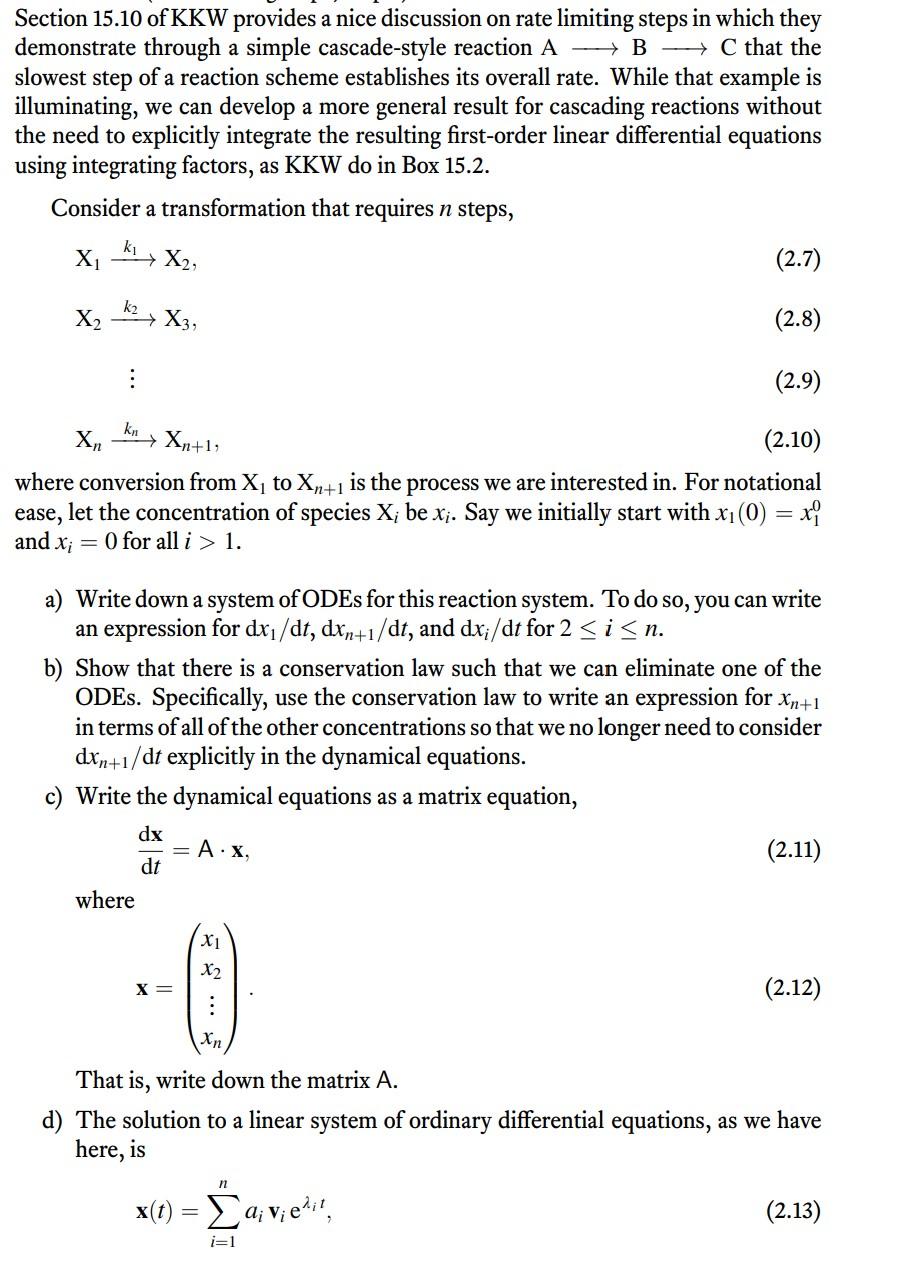
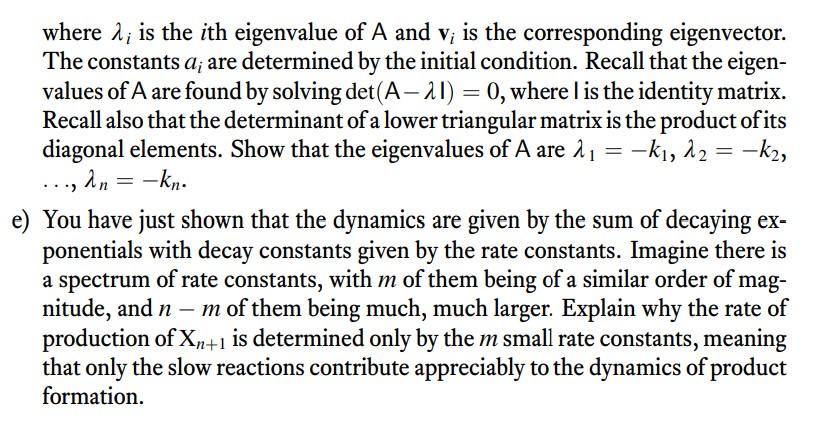
Box 15.2 Integrating the rate equation for a reaction with intermediate steps We seek to integrate Equation 15.25 in the main text: Equation 15.2.3 is then integrated to give: dtd[B]=+k1[A]k2[B]=k1[A]0ek1tk2[B] Equation 15.25 has just [B] and t as variables, so it can To apply Equation 15.2.4 to integrate the rate equation be integrated. This integration is not straightforward, (Equation 15.25), we use the initial condition that [B]0= because the variables cannot easily be separated. How- 0 and substitute as follows: ever, the general form of Equation 15.25 derives from an application of the product rule for differentiation. Notice that Equation 15.25 is equivalent to a differential equation of the general form: dxdy+cy=g(x)y=[B](whichisafunctionofx=t) If we apply the product rule for differentiation to an This gives us Equation 15.21 in the main text: exponential function, we get the following: dxd(ecxy)=cecxy+ecxdxdy=ecx(dxdy+cy)[B]=k2k1k1[A]0(ek1tek2t) By combining Equations 15.2.1 and 15.2.2, we get: You can verify that, for [B] as defined in Equation 15.26, dxd(ecxy)=ecxg(x) the derivative, d[B]/dt, gives Equation 15.25, as it should. Section 15.10 of KKW provides a nice discussion on rate limiting steps in which they demonstrate through a simple cascade-style reaction ABC that the slowest step of a reaction scheme establishes its overall rate. While that example is illuminating, we can develop a more general result for cascading reactions without the need to explicitly integrate the resulting first-order linear differential equations using integrating factors, as KKW do in Box 15.2. Consider a transformation that requires n steps, X1k1X2,X2k2X3,XnknXn+1, where conversion from X1 to Xn+1 is the process we are interested in. For notational ease, let the concentration of species Xi be xi. Say we initially start with x1(0)=x10 and xi=0 for all i>1. a) Write down a system of ODEs for this reaction system. To do so, you can write an expression for dx1/dt,dxn+1/dt, and dxi/dt for 2in. b) Show that there is a conservation law such that we can eliminate one of the ODEs. Specifically, use the conservation law to write an expression for xn+1 in terms of all of the other concentrations so that we no longer need to consider dxn+1/dt explicitly in the dynamical equations. c) Write the dynamical equations as a matrix equation, dtdx=Ax, where x=x1x2xn That is, write down the matrix A. d) The solution to a linear system of ordinary differential equations, as we have here, is x(t)=i=1naivieit where i is the i th eigenvalue of A and vi is the corresponding eigenvector. The constants ai are determined by the initial condition. Recall that the eigenvalues of A are found by solving det(AI)=0, where l is the identity matrix. Recall also that the determinant of a lower triangular matrix is the product of its diagonal elements. Show that the eigenvalues of A are 1=k1,2=k2, ,n=kn. e) You have just shown that the dynamics are given by the sum of decaying exponentials with decay constants given by the rate constants. Imagine there is a spectrum of rate constants, with m of them being of a similar order of magnitude, and nm of them being much, much larger. Explain why the rate of production of Xn+1 is determined only by the m small rate constants, meaning that only the slow reactions contribute appreciably to the dynamics of product formation









