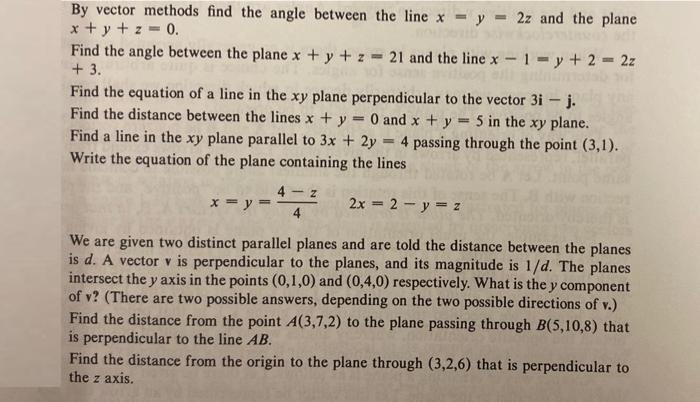
By vector methods find the angle between the line x=y= 2z and the plane x+y+z=0. Find the angle between the plane x + y + z = 21 and the line x 1 = y + 2 = 2z +3. Find the equation of a line in the xy plane perpendicular to the vector 3i - j. Find the distance between the lines x + y 0 and x + y = 5 in the xy plane. = Find a line in the xy plane parallel to 3x + 2y = 4 passing through the point (3,1). Write the equation of the plane containing the lines x = y = 4 4 Z 2x = 2-y=z We are given two distinct parallel planes and are told the distance between the planes is d. A vector v is perpendicular to the planes, and its magnitude is 1/d. The planes intersect the y axis in the points (0,1,0) and (0,4,0) respectively. What is the y component of v? (There are two possible answers, depending on the two possible directions of v.) Find the distance from the point A(3,7,2) to the plane passing through B(5,10,8) that is perpendicular to the line AB. Find the distance from the origin to the plane through (3,2,6) that is perpendicular to the z axis.