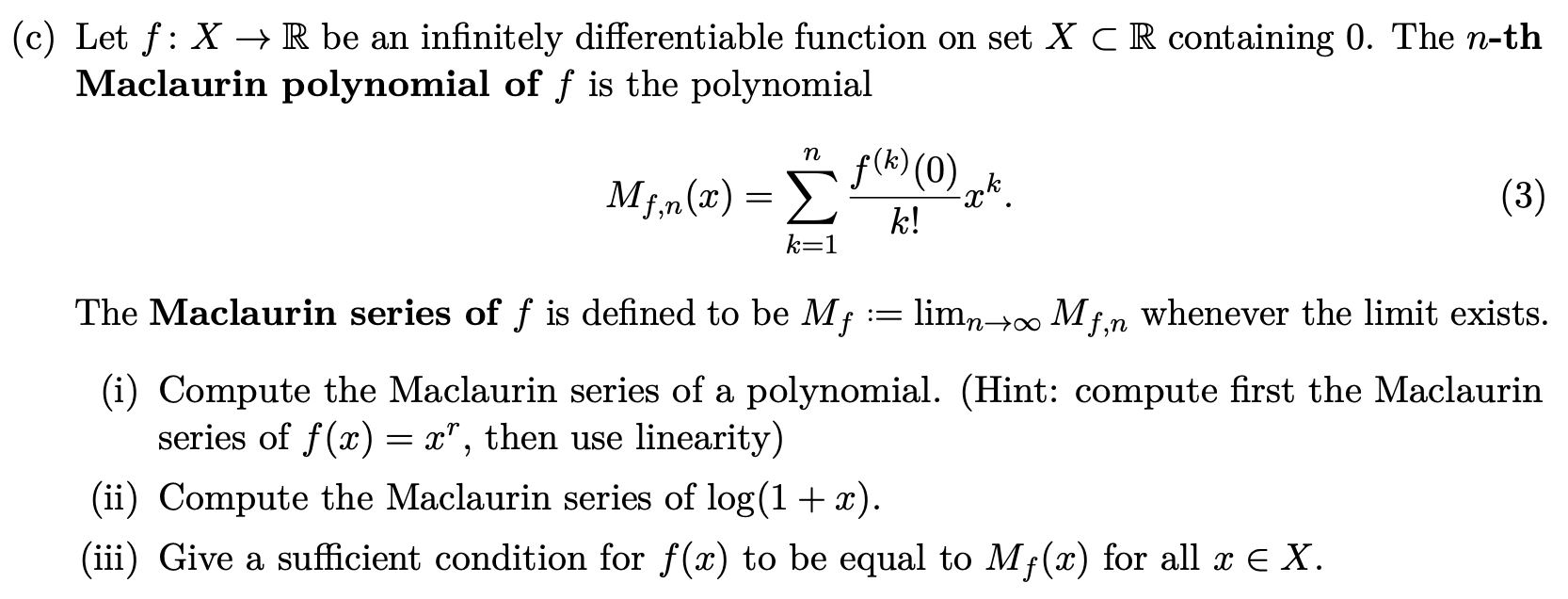
(c) Let f: X R be an infinitely differentiable function on set X CR containing 0. The n-th Maclaurin polynomial of f is the polynomial Mf,n(x) = f(h)(0) prk. = k! k=1 n The Maclaurin series of is defined to be M limn Mf,n whenever the limit exists. (i) Compute the Maclaurin series of a polynomial. (Hint: compute first the Maclaurin series of f(x) = x, then use linearity) (ii) Compute the Maclaurin series of log(1 + x). (iii) Give a sufficient condition for f(x) to be equal to M(x) for all x X. (3) :=