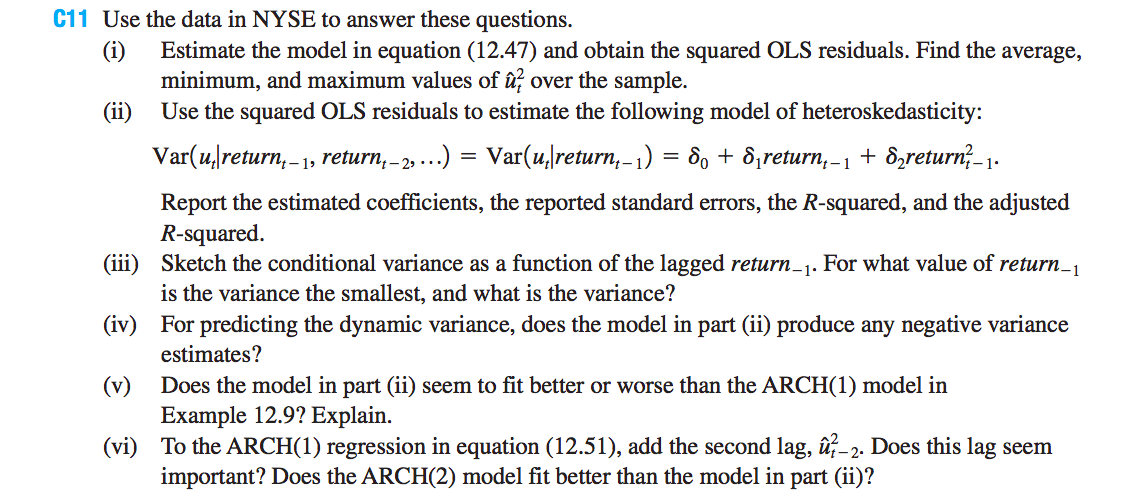
C11 Use the data in NYSE to answer these questions. (i) Estimate the model in equation (12.47) and obtain the squared OLS residuals. Find the average, minimum, and maximum values of z over the sample. (ii) Use the squared OLS residuals to estimate the following model of heteroskedasticity: Var(u.[return,-1, return,-2, ...) = Var(u)return - 1) = 80 + dj return, -1 + dreturn?-1. Report the estimated coefficients, the reported standard errors, the R-squared, and the adjusted R-squared (iii) Sketch the conditional variance as a function of the lagged return_1. For what value of return_1 is the variance the smallest, and what is the variance? (iv) For predicting the dynamic variance, does the model in part (ii) produce any negative variance estimates? Does the model in part (ii) seem to fit better or worse than the ARCH(1) model in Example 12.9? Explain. (vi) To the ARCH(1) regression in equation (12.51), add the second lag, z-2. Does this lag seem important? Does the ARCH(2) model fit better than the model in part (ii)? (v) Does C11 Use the data in NYSE to answer these questions. (i) Estimate the model in equation (12.47) and obtain the squared OLS residuals. Find the average, minimum, and maximum values of z over the sample. (ii) Use the squared OLS residuals to estimate the following model of heteroskedasticity: Var(u.[return,-1, return,-2, ...) = Var(u)return - 1) = 80 + dj return, -1 + dreturn?-1. Report the estimated coefficients, the reported standard errors, the R-squared, and the adjusted R-squared (iii) Sketch the conditional variance as a function of the lagged return_1. For what value of return_1 is the variance the smallest, and what is the variance? (iv) For predicting the dynamic variance, does the model in part (ii) produce any negative variance estimates? Does the model in part (ii) seem to fit better or worse than the ARCH(1) model in Example 12.9? Explain. (vi) To the ARCH(1) regression in equation (12.51), add the second lag, z-2. Does this lag seem important? Does the ARCH(2) model fit better than the model in part (ii)? (v) Does