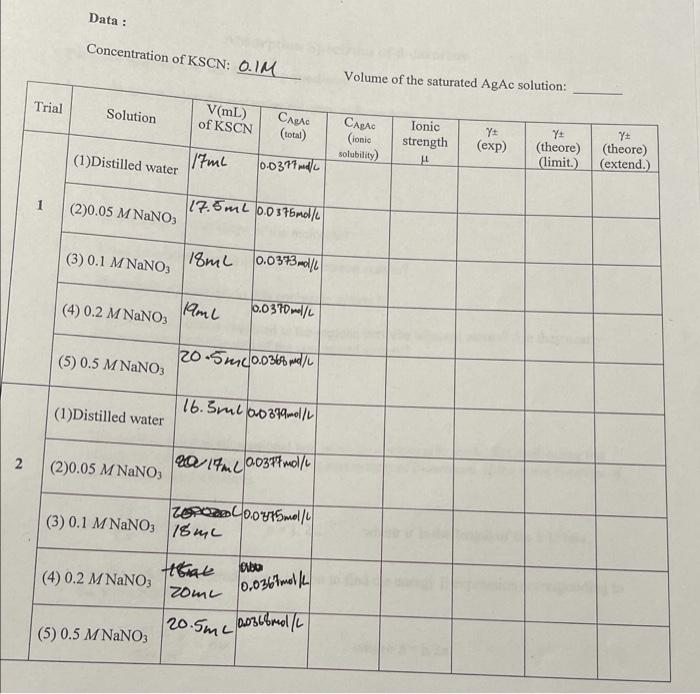
Calculation : (1) Total solubility of AgAc in the solution (M): (Hint: use the concentration and the volume of KSCN in the titration to find the total concentration of AgAc) CAEAd(toal)=17mLKSCN+85mLH20+1ral fomk indeator =103mL Mw AgAt =334.896mel 1.3/334.696=0.603816molAgAc M=0.00308/10310.3=0.0377mol/LAgAc (2) Concentration of undissociated AgAc in the solution (M): (Hint: use Eq. (3)) CAgAc(undisociated)= (3) Concentration of dissociated AgAc (ie: ionic solubility) in the solution (M): CAAst(ionicsolutility= (4) The ionic strength of each solution from molar concentration: (Hint: the summation in Eq. (7) is over all ionic species present) (5) Equilibrium constant Ksp : Plot log10c versus A/(1+B), where A is taken as 0.509,B as 1.25. Ksp for AgAc will be determined from the intercept of your graph of the data (see Eq.11). Since there will only be ten titrations, it is probably best to plot all ten data points separately rather than averaging for each solution. If a single point is obviously out of line, you can ignore it when you draw your best-fit line. Place about 1.3g of AgAc in each of five clean, dry sample flasks. The AgAc should be weighed out roughly. Each flask should be indelibly numbered or tagged to provide identification. Fill each flask with 85mL of one of the following solutions: 1. Distilled water 2. 0.05MNaNO3 3. 0.1MNaNO3 4. 0.2MNaNO3 5. 0.5MNaNO3 Set the sample flasks in a large beaker of hot water until the solutions have warmed up to about 55C. Then stopper the flasks tightly and shake vigorously for 2min. Place the flasks in a 25C thermostat bath, shake them by hand about once every 5min, and allow 1hr for equilibrium. At the end of the hour, leave the flasks in the bath for 5 or 10min to allow the solid to settle. Without removing the flasks from the bath, withdraw two 25mL sample from each flask; and use a pipette fitted at the tip with a filter consisting of a short (1-in.) piece of rubber tubing packed with cotton. Remove the filter before adjusting the level of the liquid in the pipette to the mark. Use a clean, dry filter for each sample, and rinse out the pipette with a few We are concerned here with the following equilibrium, 1 which occurs in the presence of excess solid silver acetate: AgAc(s)=Ag+(insoln.)+Ac(insoln.)(25C,1atm) However, it has been shown 2 that silver acetate exists in solution not only as Ag+and Ac, but also as undissociated silver acetate AgAc, and as complex ions AgAc2,Ag2Ac+and possibly other complex ionic forms. For our purposes complex ions may be neglected, and silver acetate will be assumed to be in solution as the undissociated neutral molecules AgAc and as the free ions Ag+and Ac. The determination of the equilibrium constant K for the change in state AgAc(s)=KAgAc(undiss.,insoln.) will not be attempted as a part of this experiment. From extensive electrochemical measurements 2 the value K=1.05102molliter1 has been obtained at 25C and 1atm. The concentration of undissociated AgAc may then be subtracted from the total concentration of silver found by titration to give the concentration c of Ag+ion. For the equilibrium given in Eq. (1) the product of the activities of Ag+and Acions at the given temperature and pressure is a constant, regardless of the concentrations of these ions or of the presence of other ionic or molecular species in solution: aAB+aAC=KSP The experimentally measurable concentrations are related to the activities by the activity coefficient : Thus, aAg+=Ag+cAg+aAc=AccAc.KSP=Ag+AccAg+cAc=2cAg+cAC where , the mean activity coefficient for AgAc in the solution concerned, is the geometric mean of the two ionic activity coefficients. If there is no common ion present from another solute, and if there is no reagent present which reduces the concentration of either ion by chemical reaction, the concentrations of Ag+and Acwill be equal to each other and to the ionic solubility c, so that we can write s2c2=Ksp Measurements of the ionic solubility c can therefore be used to determine the mean activity coefficient for AgAc in solutions containing other ions in various concentrations, provided the constant K can be determined. Debye-Hckel theory 3 In the limit of zero ionic strength the activities of ions are equal to their concentrations, the activity coefficients thus becoming unity in the limit. The ionic strength is defined by the expression =21cizj2 where ci is the concentration of the i th ionic species, zi is the charge of the ion in units of the electronic charge, and the sum is taken over all ionic species present. The simple Debye-Hckel "limiting law" logJ=Az,2 predicts the activity coefficient of ion j at low ionic strengths but becomes seriously inexact at even moderate ionic strengths. A more complete form of the Debye- Hckel theory, which holds well for ionic strengths, is represented by the equation 3 logj=zj21+BjA where A and Bj are constants. The value of A can be calculated theoretically; it depends on characteristics of the solvent and on the temperature. If logarithms to the base 10 are used, it diameter of of about 0.509 for aqueous solutions at 25C.Bj depends in addition on the in the solution ion and also on the diameters of other ions of opposite sign that may be prest given electrolyte is a good approximation, it can be shown that the mean activity coefficient of a log=z+z1+BA where B is the mean of the Bj values of the two ions; the limiting law is, of course, log=z+zA When is calculated using concentrations in moles per liter of solvent (or, in the case of dilute aqueous solutions, the molality which is mol/1000 g solvent), the constant B is found to be close to unity for many salts at 25C4 For of AgAc in the system to be studied, B has been found empirically 5 to be about 1.25 at 25C when is expressed in moles per liter of solution. Determination of KsP If we combine Eq. (9) with Eq. (6) we obtain for the case where the z 's are unity logc=21logKsp+1+BA If logc is plotted against , a smooth curve should be obtained, and when this curve is extrapolated to =0, the intercept will give logK. A more nearly linear curve can be obtained by plotting logc versus A(1+B); this facilitates extrapolation to zero concentration from data at moderate ionic strengths. By means of this extrapolation the activity product K can be determined, and by application of Eq. (6) the activity coefficient for AgAc can be calculated for each solution studied. Place about 1.3g of AgAc in each of five clean, dry sample flasks. The AgAc should be weighed out roughly. Each flask should be indelibly numbered or tagged to provide identification. Fill each flask with 85mL of one of the following solutions: 1. Distilled water 2. 0.05MNaNO3 3. 0.1MNaNO3 4. 0.2MNaNO3 5. 0.5MNaNO3 Set the sample flasks in a large beaker of hot water until the solutions have warmed up to about 55C. Then stopper the flasks tightly and shake vigorously for 2min. Place the flasks in a 25C thermostat bath, shake them by hand about once every 5min, and allow 1hr for equilibrium. At the end of the hour, leave the flasks in the bath for 5 or 10min to allow the solid to settle. Without removing the flasks from the bath, withdraw two 25mL sample from each flask; and use a pipette fitted at the tip with a filter consisting of a short (1-in.) piece of rubber tubing packed with cotton. Remove the filter before adjusting the level of the liquid in the pipette to the mark. Use a clean, dry filter for each sample, and rinse out the pipette with a few milliliters of filtered solution before taking each sample. Discharge each sample into a 200-mL Erlenmeyer flask containing 15mL of distilled H2O and 5mL of 6MHNO3. Determination of the concentration of Ag+in the saturated AgAc solution The amount of silver ion in each sample is determined by titration with 0.1NKSCN solution. Add about 1mL of 0.1M ferric alum solution to each flask as an indicator; titrate to the appearance of a faint reddish-brown color which does not disappear on shaking. Record the volume of KSCN solution used. Concentration of KSCN: 0.1M Volume of the saturated AgAc solution: Calculation : (1) Total solubility of AgAc in the solution (M): (Hint: use the concentration and the volume of KSCN in the titration to find the total concentration of AgAc) CAEAd(toal)=17mLKSCN+85mLH20+1ral fomk indeator =103mL Mw AgAt =334.896mel 1.3/334.696=0.603816molAgAc M=0.00308/10310.3=0.0377mol/LAgAc (2) Concentration of undissociated AgAc in the solution (M): (Hint: use Eq. (3)) CAgAc(undisociated)= (3) Concentration of dissociated AgAc (ie: ionic solubility) in the solution (M): CAAst(ionicsolutility= (4) The ionic strength of each solution from molar concentration: (Hint: the summation in Eq. (7) is over all ionic species present) (5) Equilibrium constant Ksp : Plot log10c versus A/(1+B), where A is taken as 0.509,B as 1.25. Ksp for AgAc will be determined from the intercept of your graph of the data (see Eq.11). Since there will only be ten titrations, it is probably best to plot all ten data points separately rather than averaging for each solution. If a single point is obviously out of line, you can ignore it when you draw your best-fit line. Place about 1.3g of AgAc in each of five clean, dry sample flasks. The AgAc should be weighed out roughly. Each flask should be indelibly numbered or tagged to provide identification. Fill each flask with 85mL of one of the following solutions: 1. Distilled water 2. 0.05MNaNO3 3. 0.1MNaNO3 4. 0.2MNaNO3 5. 0.5MNaNO3 Set the sample flasks in a large beaker of hot water until the solutions have warmed up to about 55C. Then stopper the flasks tightly and shake vigorously for 2min. Place the flasks in a 25C thermostat bath, shake them by hand about once every 5min, and allow 1hr for equilibrium. At the end of the hour, leave the flasks in the bath for 5 or 10min to allow the solid to settle. Without removing the flasks from the bath, withdraw two 25mL sample from each flask; and use a pipette fitted at the tip with a filter consisting of a short (1-in.) piece of rubber tubing packed with cotton. Remove the filter before adjusting the level of the liquid in the pipette to the mark. Use a clean, dry filter for each sample, and rinse out the pipette with a few We are concerned here with the following equilibrium, 1 which occurs in the presence of excess solid silver acetate: AgAc(s)=Ag+(insoln.)+Ac(insoln.)(25C,1atm) However, it has been shown 2 that silver acetate exists in solution not only as Ag+and Ac, but also as undissociated silver acetate AgAc, and as complex ions AgAc2,Ag2Ac+and possibly other complex ionic forms. For our purposes complex ions may be neglected, and silver acetate will be assumed to be in solution as the undissociated neutral molecules AgAc and as the free ions Ag+and Ac. The determination of the equilibrium constant K for the change in state AgAc(s)=KAgAc(undiss.,insoln.) will not be attempted as a part of this experiment. From extensive electrochemical measurements 2 the value K=1.05102molliter1 has been obtained at 25C and 1atm. The concentration of undissociated AgAc may then be subtracted from the total concentration of silver found by titration to give the concentration c of Ag+ion. For the equilibrium given in Eq. (1) the product of the activities of Ag+and Acions at the given temperature and pressure is a constant, regardless of the concentrations of these ions or of the presence of other ionic or molecular species in solution: aAB+aAC=KSP The experimentally measurable concentrations are related to the activities by the activity coefficient : Thus, aAg+=Ag+cAg+aAc=AccAc.KSP=Ag+AccAg+cAc=2cAg+cAC where , the mean activity coefficient for AgAc in the solution concerned, is the geometric mean of the two ionic activity coefficients. If there is no common ion present from another solute, and if there is no reagent present which reduces the concentration of either ion by chemical reaction, the concentrations of Ag+and Acwill be equal to each other and to the ionic solubility c, so that we can write s2c2=Ksp Measurements of the ionic solubility c can therefore be used to determine the mean activity coefficient for AgAc in solutions containing other ions in various concentrations, provided the constant K can be determined. Debye-Hckel theory 3 In the limit of zero ionic strength the activities of ions are equal to their concentrations, the activity coefficients thus becoming unity in the limit. The ionic strength is defined by the expression =21cizj2 where ci is the concentration of the i th ionic species, zi is the charge of the ion in units of the electronic charge, and the sum is taken over all ionic species present. The simple Debye-Hckel "limiting law" logJ=Az,2 predicts the activity coefficient of ion j at low ionic strengths but becomes seriously inexact at even moderate ionic strengths. A more complete form of the Debye- Hckel theory, which holds well for ionic strengths, is represented by the equation 3 logj=zj21+BjA where A and Bj are constants. The value of A can be calculated theoretically; it depends on characteristics of the solvent and on the temperature. If logarithms to the base 10 are used, it diameter of of about 0.509 for aqueous solutions at 25C.Bj depends in addition on the in the solution ion and also on the diameters of other ions of opposite sign that may be prest given electrolyte is a good approximation, it can be shown that the mean activity coefficient of a log=z+z1+BA where B is the mean of the Bj values of the two ions; the limiting law is, of course, log=z+zA When is calculated using concentrations in moles per liter of solvent (or, in the case of dilute aqueous solutions, the molality which is mol/1000 g solvent), the constant B is found to be close to unity for many salts at 25C4 For of AgAc in the system to be studied, B has been found empirically 5 to be about 1.25 at 25C when is expressed in moles per liter of solution. Determination of KsP If we combine Eq. (9) with Eq. (6) we obtain for the case where the z 's are unity logc=21logKsp+1+BA If logc is plotted against , a smooth curve should be obtained, and when this curve is extrapolated to =0, the intercept will give logK. A more nearly linear curve can be obtained by plotting logc versus A(1+B); this facilitates extrapolation to zero concentration from data at moderate ionic strengths. By means of this extrapolation the activity product K can be determined, and by application of Eq. (6) the activity coefficient for AgAc can be calculated for each solution studied. Place about 1.3g of AgAc in each of five clean, dry sample flasks. The AgAc should be weighed out roughly. Each flask should be indelibly numbered or tagged to provide identification. Fill each flask with 85mL of one of the following solutions: 1. Distilled water 2. 0.05MNaNO3 3. 0.1MNaNO3 4. 0.2MNaNO3 5. 0.5MNaNO3 Set the sample flasks in a large beaker of hot water until the solutions have warmed up to about 55C. Then stopper the flasks tightly and shake vigorously for 2min. Place the flasks in a 25C thermostat bath, shake them by hand about once every 5min, and allow 1hr for equilibrium. At the end of the hour, leave the flasks in the bath for 5 or 10min to allow the solid to settle. Without removing the flasks from the bath, withdraw two 25mL sample from each flask; and use a pipette fitted at the tip with a filter consisting of a short (1-in.) piece of rubber tubing packed with cotton. Remove the filter before adjusting the level of the liquid in the pipette to the mark. Use a clean, dry filter for each sample, and rinse out the pipette with a few milliliters of filtered solution before taking each sample. Discharge each sample into a 200-mL Erlenmeyer flask containing 15mL of distilled H2O and 5mL of 6MHNO3. Determination of the concentration of Ag+in the saturated AgAc solution The amount of silver ion in each sample is determined by titration with 0.1NKSCN solution. Add about 1mL of 0.1M ferric alum solution to each flask as an indicator; titrate to the appearance of a faint reddish-brown color which does not disappear on shaking. Record the volume of KSCN solution used. Concentration of KSCN: 0.1M Volume of the saturated AgAc solution