Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Calculus 3 Section 14.3 Reading Assignment: Partial Derivatives I am suck with this question. Question 1 Answer: Given a 3D surface defined by the multivariate

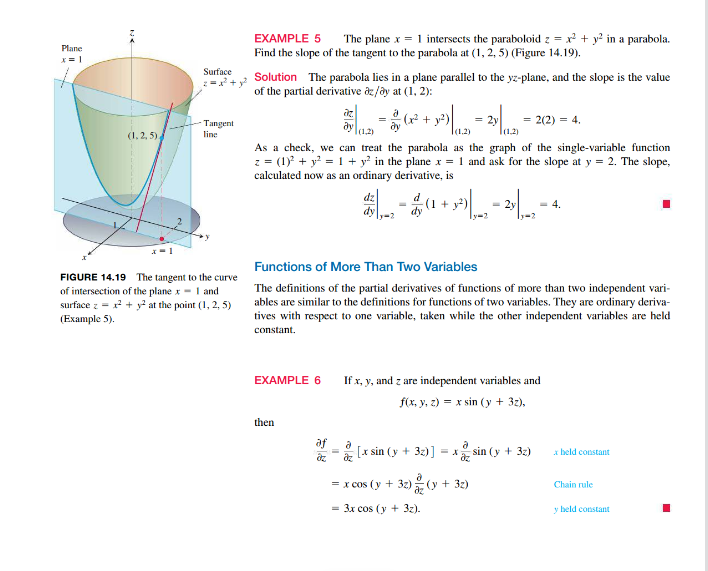
Calculus 3 Section 14.3 Reading Assignment: Partial Derivatives
I am suck with this question.
Question 1 Answer: Given a 3D surface defined by the multivariate function z=f(x,y), which is a 3D surface, where x and y are horizontal rectangular coordinates and z is the vertical coordinate:
- the partial derivative off(x,y) with respect to x, i.e. xf, at a given point(x0,y0) corresponds to the tangent line that changes along x and z, with y kept constant (y=y0). This tangent line lies within the planey=y0 which is parallel to the x-z plane.
- the partial derivative off(x,y) with respect to y, i.e. yf, at a given point(x0,y0) corresponds to the tangent line that changes along y and z, with x kept constant (x=x0). This tangent line lies within the planex=x0 which is parallel to the y-z plane.
Question 2. Read Example 5 (p. 827). Based on the question, how can you tell which partial derivative should be used for the solution? Explain how this connects with your answer from Question 1 above.
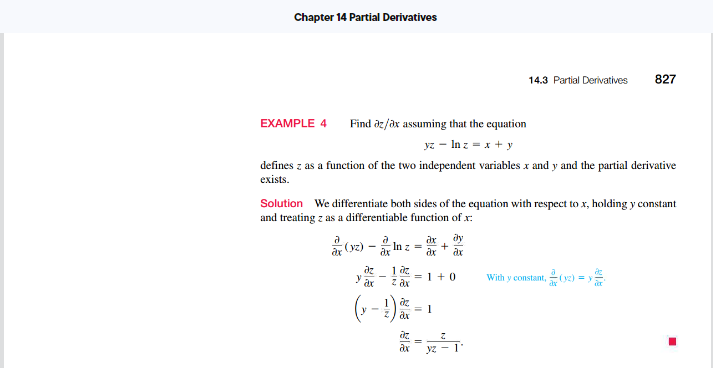
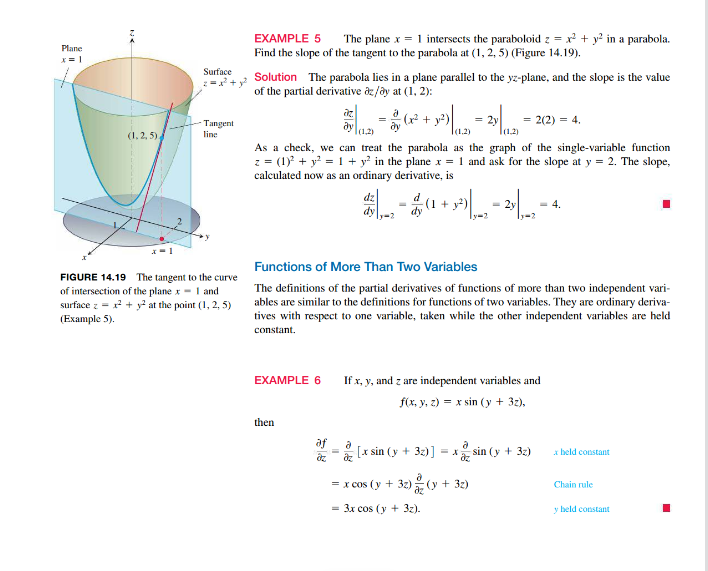
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


