Calculus problems.

In this project you will design an optimal soda can.

Problem

Cans of soft drinks are made from aluminum.Due to the soaring prices, the beverage company executives are looking for all possible cost-cutting measures.You are being asked to find a way to redesign the traditional can to decrease cost as much as possible.The manufacturer fills the containers with 355 cm³ of liquid and leaves 15 cm³ of open space, so the total volume of 370 cm³ must be maintained.

Part 1: Draw a Model of the Can

1.Sketch a right circular cylinder and label the radius, r, and height, h.Give the formulas for volume and surface area of a right circular cylinder.What quantity is known? Take a picture of your work/drawing and include it here.

2.The goal is to minimize the surface area to keep cost as low as possible but note that the formula has too many variables.

a. Use the information in (1) to eliminate the height from the surface area formula so that you have surface area as a function of radius only. Show your work.

b. What is the realistic domain of the surface area function you created in (a)?

c. Graph the surface area function in desmos.Label each axis by name and units. Include a picture or sketch of your graph.

3.We have been working on finding minimums and maximums of functions over intervals.

a. Refer the graph from 2(c) and use desmos to approximate the value of the radius for which the surface area is the least.What is that radius, to the nearest hundredth, and what is the corresponding surface area, to the nearest hundredth?Don't forget units.

• Radius =
• Surface Area =

b. Now use calculus. Find the derivative of the surface area function and determine the minimum surface area using the derivative.Show your support work.

c. Do you get the same radius value as in a and b?Should the values be the same?Explain.

4.For the radius which produces the minimum surface area, determine the corresponding dimensions.Round all to the nearest hundredth.Include your work.

• Radius =
• Diameter =
• Height =

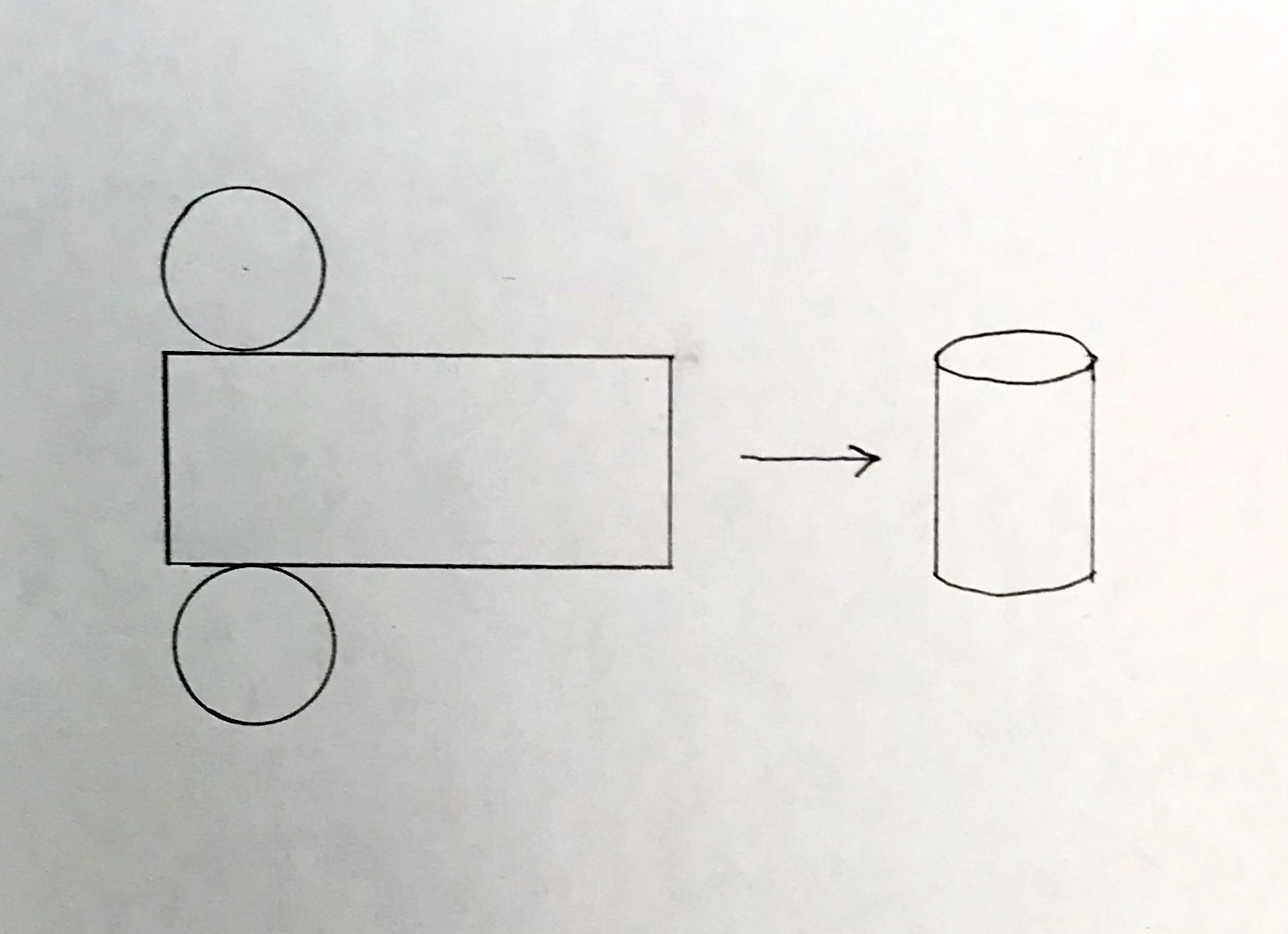
5.Use the dimensions you have determined in (4) to make a three-dimensional beverage can.You can use a rectangle and two circles as shown below.Draw them to scale using a straightedge and compass (if you have them).

\f