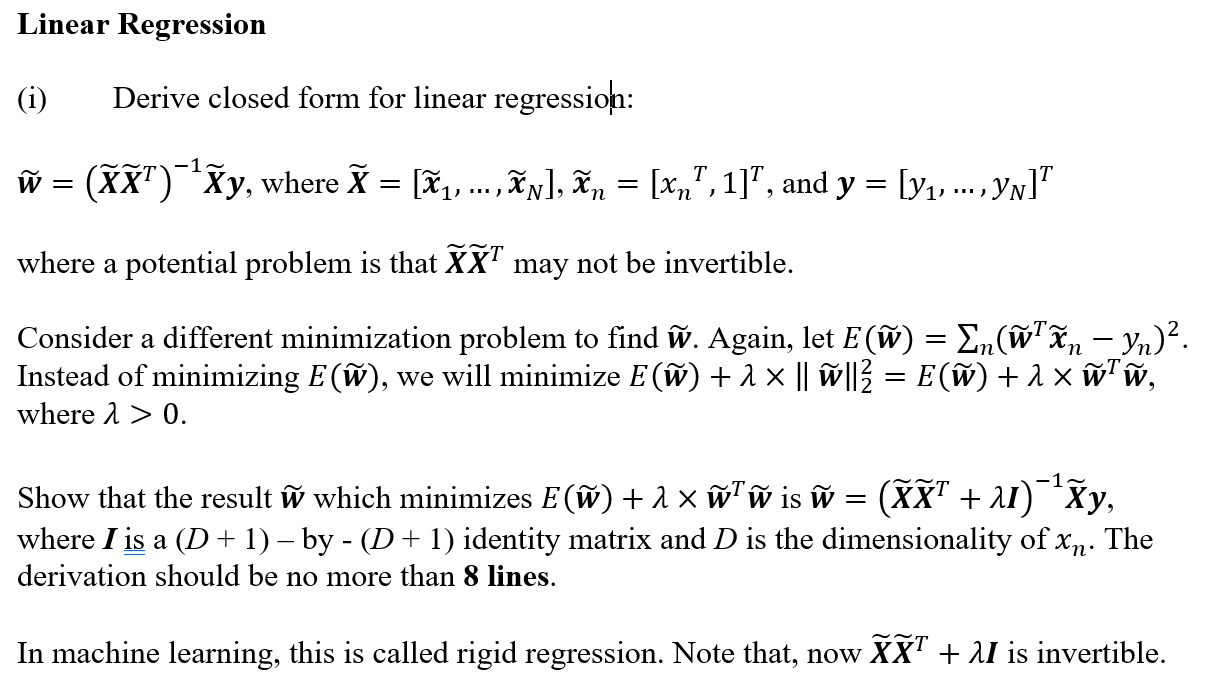
Can someone help me with these questions?

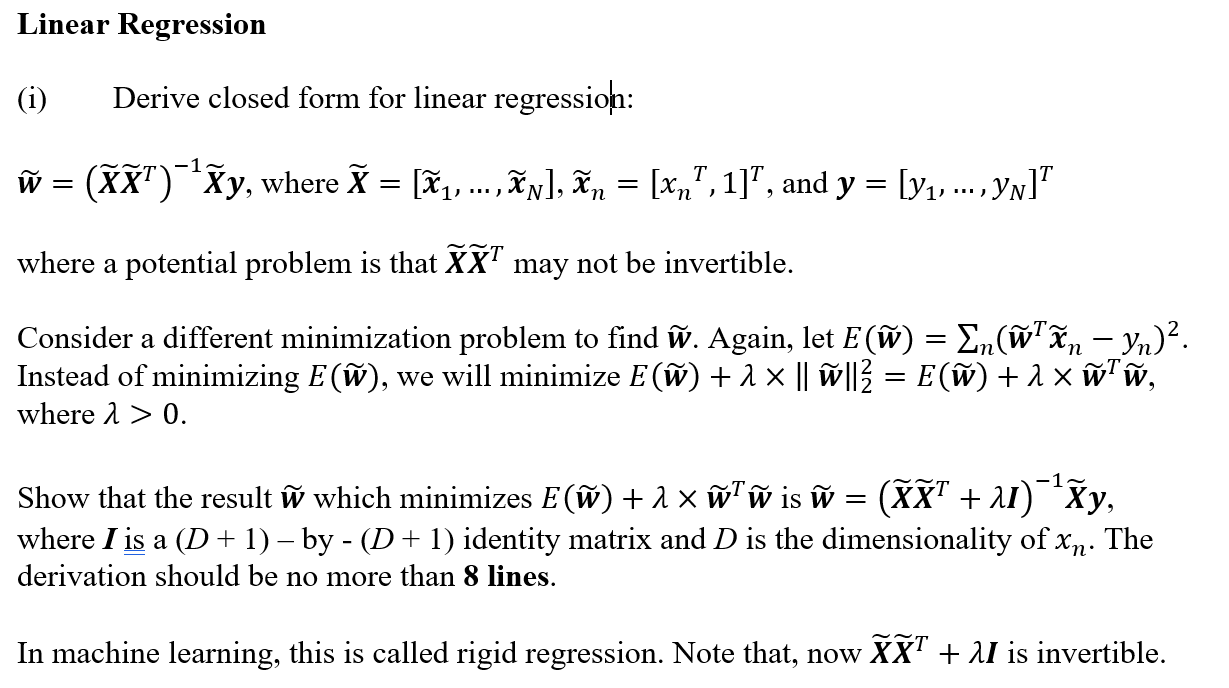
Linear Regression (i) Derive closed form for linear regressiob: = (NRT) - = [*1, ... , XN , in = [xn T, 1] T, and y where a potential problem is that XX T may not be invertible. 2 Consider a different minimization problem to find W. Again, let E (W) = En(W yn) Instead of minimizing E(W), we will minimize E@) + 1 > < II = + 1 X j' TW, where 1 > 0. Show that the result which minimizes E(W) + 1 X is = + *y, where I is a (D + l) by - (D + l) identity matrix and D is the dimensionality of xn. The derivation should be no more than 8 lines. In machine learning, this is called rigid regression. Note that, now XX T + 11 is invertible.