Can you give the answer for all of this questions and with explanation, Please?

Thank you so much!

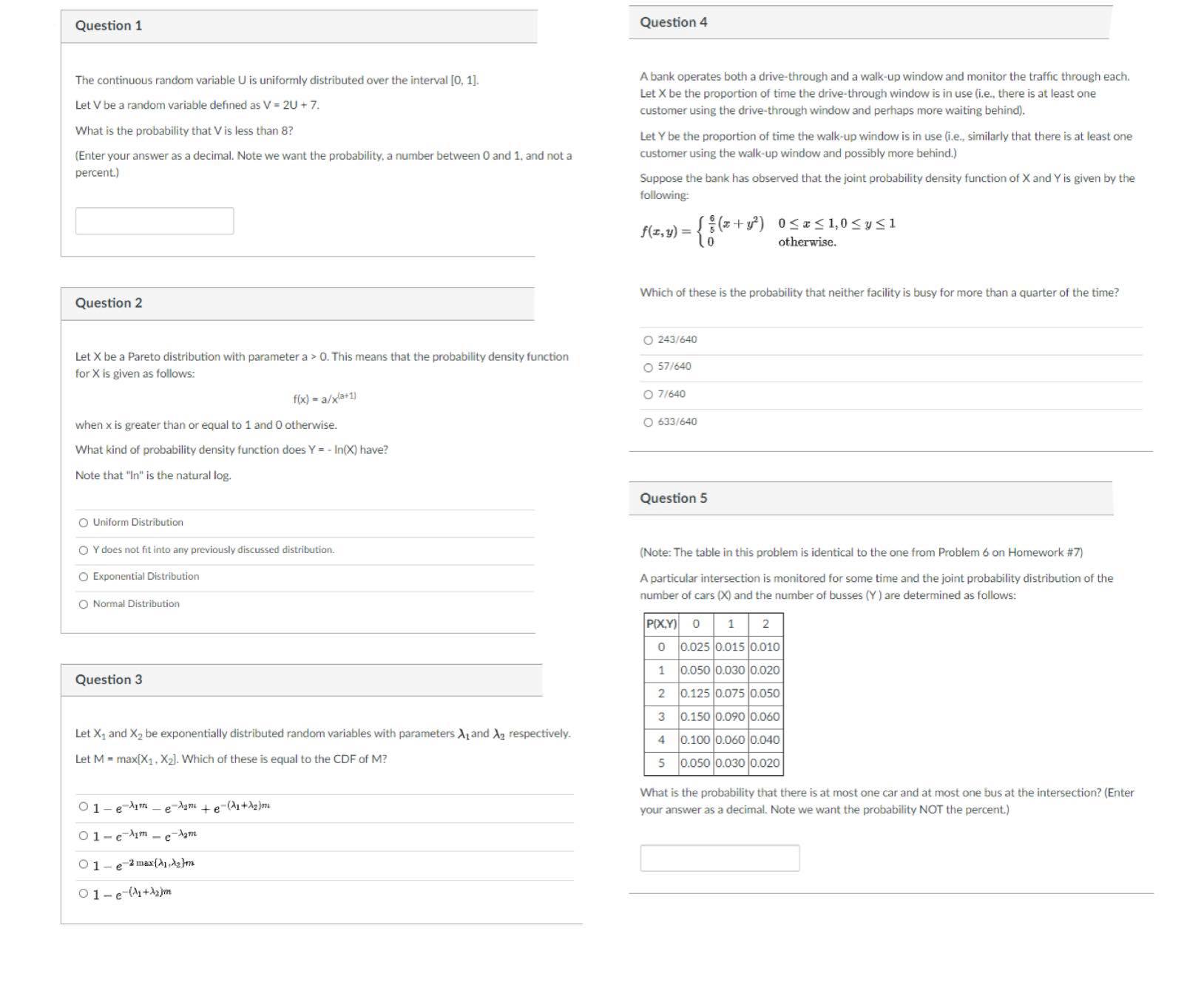
Question 1 Question 4 The continuous random variable U is uniformly distributed over the interval [0, 1]. A bank operates both a drive-through and a walk-up window and monitor the traffic through each. Let V be a random variable defined as V = 2U + 7. Let X be the proportion of time the drive-through window is in use (i.e., there is at least one customer using the drive-through window and perhaps more waiting behind). What is the probability that V is less than 8? Let Y be the proportion of time the walk-up window is in use (i.e., similarly that there is at least one (Enter your answer as a decimal. Note we want the probability, a number between 0 and 1, and not a customer using the walk-up window and possibly more behind.) percent.) Suppose the bank has observed that the joint probability density function of X and Y is given by the following: f(z, y) = (2+y') OS251,0Sys1 otherwise. Question 2 Which of these is the probability that neither facility is busy for more than a quarter of the time? O 243/640 Let X be a Pareto distribution with parameter a > 0. This means that the probability density function for X is given as follows: 57/640 f(x) = a/xla+1) O 7/640 when x is greater than or equal to 1 and 0 otherwise. O 633/640 What kind of probability density function does Y = - In(X) have? Note that "In" is the natural log. Question 5 O Uniform Distribution Y does not fit into any previously discussed distribution. (Note: The table in this problem is identical to the one from Problem 6 on Homework #7) O Exponential Distribution A particular intersection is monitored for some time and the joint probability distribution of the O Normal Distribution number of cars (X) and the number of busses (Y ) are determined as follows: P(X,Y) 0 1 2 0 0.025 0.015 0.010 Question 3 1 0.050 0.030 0.020 2 0.125 0.075 0.050 3 0.150 0.090 0.060 Let X, and X2 be exponentially distributed random variables with parameters >, and Ag respectively. 0.100 0.060 0.040 Let M = max[X1. X2]. Which of these is equal to the CDF of M? 5 0.050 0.030 0.020 O1-e dim _ e dam + e (1+Az)m What is the probability that there is at most one car and at most one bus at the intersection? (Enter your answer as a decimal. Note we want the probability NOT the percent.) 01-e lim - e- dam O1 -e 2 max(21,d2)m 01-e (lit))m