Can you please help me determine NPV and discount factors for all years?

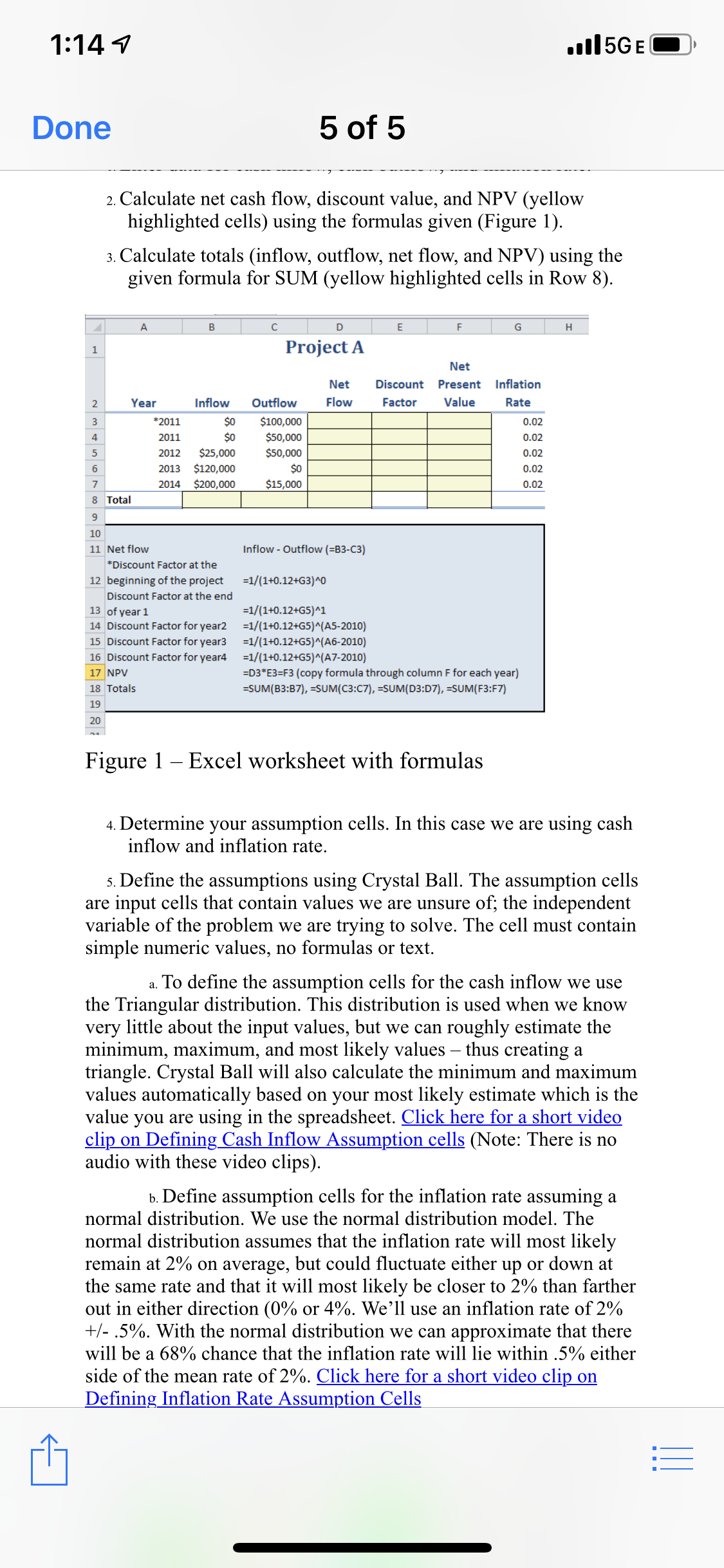
1:14 7 .11 5GE D Done 5 of 5 2. Calculate net cash flow, discount value, and NPV (yellow highlighted cells) using the formulas given (Figure 1). 3. Calculate totals (inflow, outflow, net flow, and NPV) using the given formula for SUM (yellow highlighted cells in Row 8). A B C E F G H 1 Project A Net Net Discount Present Inflation 2 Year Inflow Outflow Flow Factor Value Rate w *2011 SO $100,000 0.02 2011 $50,000 0.02 2012 $25,000 $50,000 0.02 2013 $120,000 SO 0.02 2014 $200,000 $15,000 0.02 8 9 10 11 Net flow Inflow - Outflow (=B3-C3) *Discount Factor at the 12 beginning of the project =1/(1+0.12+G3)^0 Discount Factor at the end 13 of year 1 =1/(1+0.12+G5)^1 14 Discount Factor for year2 =1/(1+0.12+G5)^(A5-2010) 15 Discount Factor for year3 =1/(1+0.12+G5)^(A6-2010) 16 Discount Factor for year4 =1/(1+0.12+G5)^(A7-2010) 17 NPV =D3*E3=F3 (copy formula through column F for each year) 18 Totals =SUM(B3:87), =SUM(C3:07), =SUM(D3:07), =SUM(F3:F7) 19 20 Figure 1 - Excel worksheet with formulas 4. Determine your assumption cells. In this case we are using cash inflow and inflation rate. 5. Define the assumptions using Crystal Ball. The assumption cells are input cells that contain values we are unsure of; the independent variable of the problem we are trying to solve. The cell must contain simple numeric values, no formulas or text. a. To define the assumption cells for the cash inflow we use the Triangular distribution. This distribution is used when we know very little about the input values, but we can roughly estimate the minimum, maximum, and most likely values - thus creating a triangle. Crystal Ball will also calculate the minimum and maximum values automatically based on your most likely estimate which is the value you are using in the spreadsheet. Click here for a short video clip on Defining Cash Inflow Assumption cells (Note: There is no audio with these video clips). b. Define assumption cells for the inflation rate assuming a normal distribution. We use the normal distribution model. The normal distribution assumes that the inflation rate will most likely remain at 2% on average, but could fluctuate either up or down at the same rate and that it will most likely be closer to 2% than farther out in either direction (0% or 4%. We'll use an inflation rate of 2% +/- .5%. With the normal distribution we can approximate that there will be a 68% chance that the inflation rate will lie within .5% either side of the mean rate of 2%. Click here for a short video clip on Defining Inflation Rate Assumption Cells