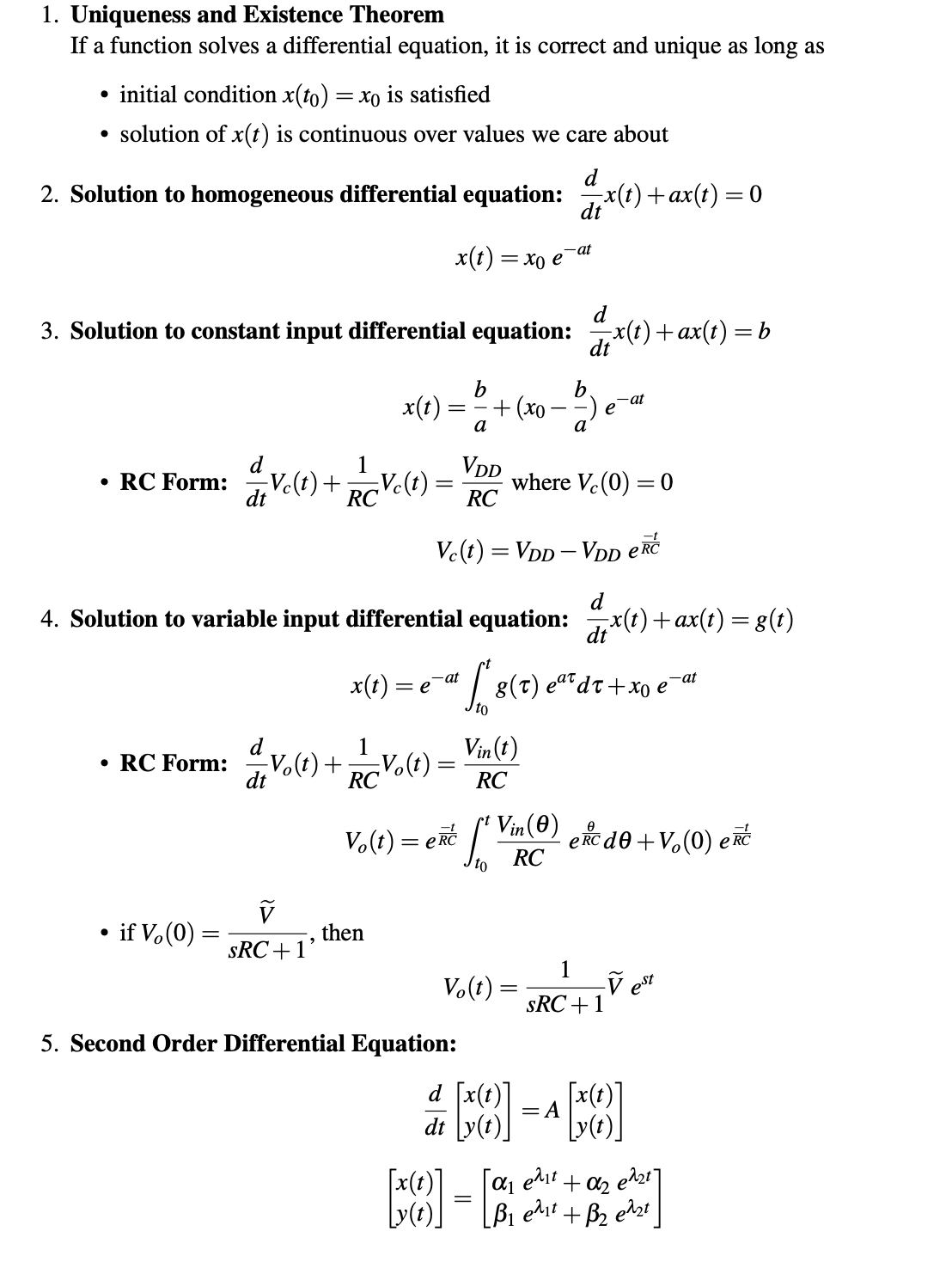
Cheat Sheet.
4 Predator-Prey Model Ellen is a keen ecologist studying life on a planet far, far away. She found two species on the planet, one predator and the other prey. You found her journal back on Earth and are trying to decipher life on this other planet. In Ellen's journal, she states the following observations about the predator and prey populations 0 The prey population grows at a rate proportional to the current prey population. 0 For a given prey population, the rate of increase of prey population decreases linearly with the predator population. As the predator population increases, it is harder for the prey population to grow. 0 The predator population also grows at a rate proportional to the current predator population. 0 For a xed predator population, predator population increases linearly with prey population. As the amount of prey increases, predators have more food and their population increases faster. Let's call these species 1: (prey) and y (predator) to keep things simple. A friend suggests using the following model for predator and prey populations: 31,960) = (a W (5) d We) = (ax - d)y (6) a) Comment on the validity of the model described in Equations 5 and 6. b) You find a data entry in Ellen's journal measuring the growth rates of predator and prey populations. At a predator population of 10 and prey population of 10, both populations increase at 10/5. With a single prey remaining, the predators starve and their population decreases at 8/ s at a population of 10 predators. At population levels of 1 predator and 10 prey, the prey population rapidly increases at 100/5. Using these observations, find the model parameters a, b, c and d. c) If we had 1000 predators on the planet and no prey. What will happen to the predator population? d) At what levels will the predator and prey populations not change any more. As we have seen in class, this set of population levels is called an equilibrium. e) We want to analyze the model at a set of population levels. We don't know how to solve the model described in Equations 5 and 6. We can, however, linearize the model around a set of population levels and analyze the population changes in that vicinity. If the predator population is 10 and the prey population is 1, linearize the system around these populations and express the new, linearized system in the form saws m 1. Uniqueness and Existence Theorem If a function solves a differential equation, it is correct and unique as long as . initial condition x(to) = xo is satisfied . solution of x(t) is continuous over values we care about 2. Solution to homogeneous differential equation: a x (t ) + ax(t ) = 0 dt x (t ) = xo e-at 3. Solution to constant input differential equation: -x (t ) + ax(t ) = b dt x (t ) = -+(xo - - ) e-at . RC Form: d RC VDD dt -Ve (t ) + Ve (t ) = RC where Vc (0 ) = 0 Vc(t) = VDD - VDD eRC 4. Solution to variable input differential equation: a, x (t) + ax(t) = 8(t) x (t ) = e at 8(t) eatdi txoe- at . RC Form: "vo (t ) + - Volt ) = Vin (1 ) dt RC Vo(t) = eRC [ VineRcde + V.(0) eRC to RC . if Vo (0) = V SRC + 1' 1, then Vo(t) = 1 SRC + 1 Vest 5. Second Order Differential Equation: x (t ) _[a ell + 02 el27 Ly(1)] LB1 elit + Breast