Question: 1. Civil engineers use the continuity equation for many applications. This simple ordinary differential equation states that the difference between (volumetric) rates of inflow

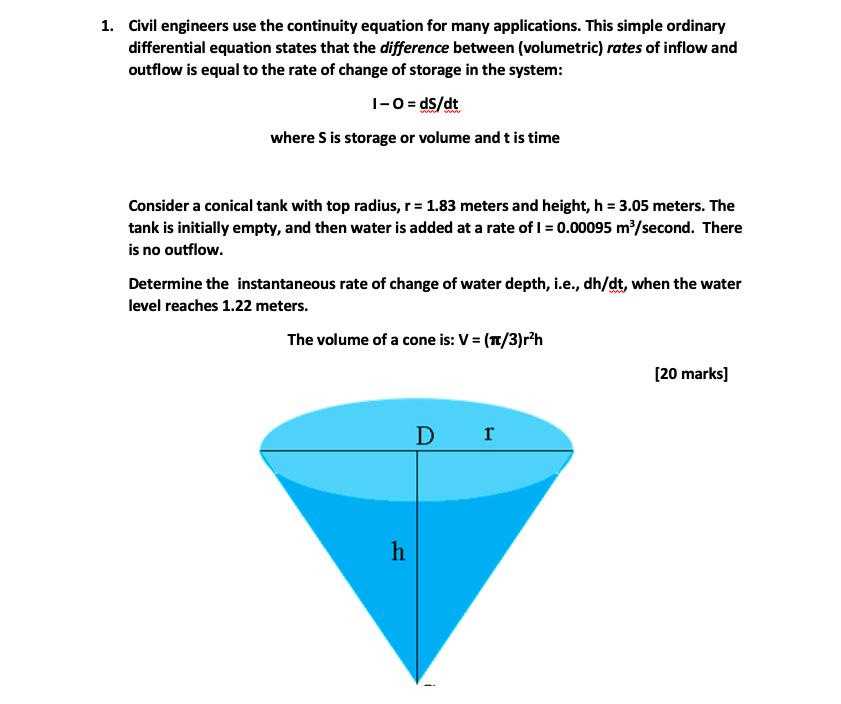
Civil engineers use the continuity equation for many applications. This simple ordinary differential equation states that the

1. Civil engineers use the continuity equation for many applications. This simple ordinary differential equation states that the difference between (volumetric) rates of inflow and outflow is equal to the rate of change of storage in the system: 1-0 = dS/dt where S is storage or volume and t is time Consider a conical tank with top radius, r = 1.83 meters and height, h = 3.05 meters. The tank is initially empty, and then water is added at a rate of I = 0.00095 m/second. There is no outflow. Determine the instantaneous rate of change of water depth, i.e., dh/dt, when the water level reaches 1.22 meters. The volume of a cone is: V = (T/3)rh [20 marks] D r h

Step by Step Solution

★★★★★

3.49 Rating (149 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Civil Engineering Questions!

The velocity is equal to the rate of change of distance x (m), (a) Substitute Eq. (1.10) and develop an analytical solution for distance as a function of time. Assume that x(0) = 0. (b) Use Euler's...

In polar coordinates, the continuity equation for steady in-compressible flow becomes Derive equations (10-10), using this relation. 1 dve = 0 r 0 : (rv,) + - r r 1 Ur V r 0 r

A conical tank with a base radius of 20 cm and height of 40 cm is initially filled with water Water drains through a small round hole at the bottom with a diameter of 1 cm. Find an expression for the...

As a practical application of Fig P1.14, often termed a sharp-crested weir, civil engineers use the following formula for flow rate: Q 3.3 BH3/2, with Q in ft3/s and B and H in feet. Is this formula...

Assuming that there is no transformation of mass to energy or vice versa, it is possible to write a continuity equation for mass. (a) If we use the continuity equation for charge as our model, what...

For the slipper-pad bearing of Prob. 1023, use the continuity equation, appropriate boundary conditions, and the one-dimensional Leibniz theorem (see Chap. 4) to show that Data from Problem 23 A...

The data set in DS 11.2.6 concerns an experiment to compare three different assembly methods for an electric motor. Use the Friedman test procedure to investigate whether there is evidence of any...

Use the financial information for Starlight from Problem 16.1. Assume now that equity accounts do not vary directly with sales but change when retained earning change or new equity is issued. The...

In Problem 67, Chapter 7 (Figure P7.31), the block diagram of a cascade scheme for the speed control of an HEV (Preitl, 2007) was represented as a unity feedback system. In that diagram the output of...

Precision Systems, Inc. (PSI)* Precision Systems, Inc. (PSI) has been in business for more than 40 years and has generally reported a positive net income. The company manufactures and sells...

Identify a company of your choice and discuss the following; 1. Using the laddering technique, and Porter's five forces discuss its competitive advantage 2. Using the value chain analysis, identify...

Carsen Company produces handcrafted pottery that uses two inputs: materials and labor. During the past quarter, 24,000 units were produced, requiring 96,000 pounds of materials and 48,000 hours of...

Differentiate the function. y = ( 3 x 4 ) 3 ( 5 x 3 ) 4 d y d x = example Get mora ho

Case Study 3 You are the director of human resources for the Midwest division Kelly Green. Two of your employees are accused of being racist towards customers. In your customer letter, she states:...

give an explanation of the difference between wifi and WiMAX from the protocol stack and give an explanation of how they work according to the stack use this for reference...

You have been appointed as a new product manager with Samsung and have been tasked to introduce the latest smartphone in a new country. Choose and research a country other than the United States, and...

Consider this reaction: 2NH3(g) N2(g) + 3H2(g) At a certain temperature it obeys this rate law rate = (7.59 10-4 s-1)[NH3 Suppose a vessel contains NH3 at a concentration of 1.01 M. Calculate the...

Shallow processing of a word is encouraged when attention is focused on: O the physical features of the word O the meaning of a word O the category of the word O the pleasantness of a word QUESTION 2...

What is the velocity(in ft/s) of a sandbag 0.25 s after it is released from a hot-air balloon that is stationary in the air?

1. Civil engineers use regression analysis to estimate repair and replacement costs of different types of piping. The interdependent variable in their analysis, X, is the diameter of the pipe( in...

Question 6 of 20 : Select the best answer for the question. 6. When a = .10, what is the rejection region that should be used to test Ho:02 =0? against Ho: 02 > 03? Assume v, = 10 and V2 = 12. O A. F...

Hello, The following questions seem a little confusing: I am trying to solve them but not getting the correct solution. I have followed the example and exercise that is given in the textbook which I...

how do civil engineers use the pythagorean theorem?

Refer to Multiple-Concept Example 4 for a review of the concepts that play roles in this problem. Civil engineers use a transit theodolite when surveying. One version of this device determines...

The data set in the spreadsheet consists of 599 records that you will be analyzing from the Bureau of Labor Statistics. The data set contains a listing of several jobs titles with yearly salaries...