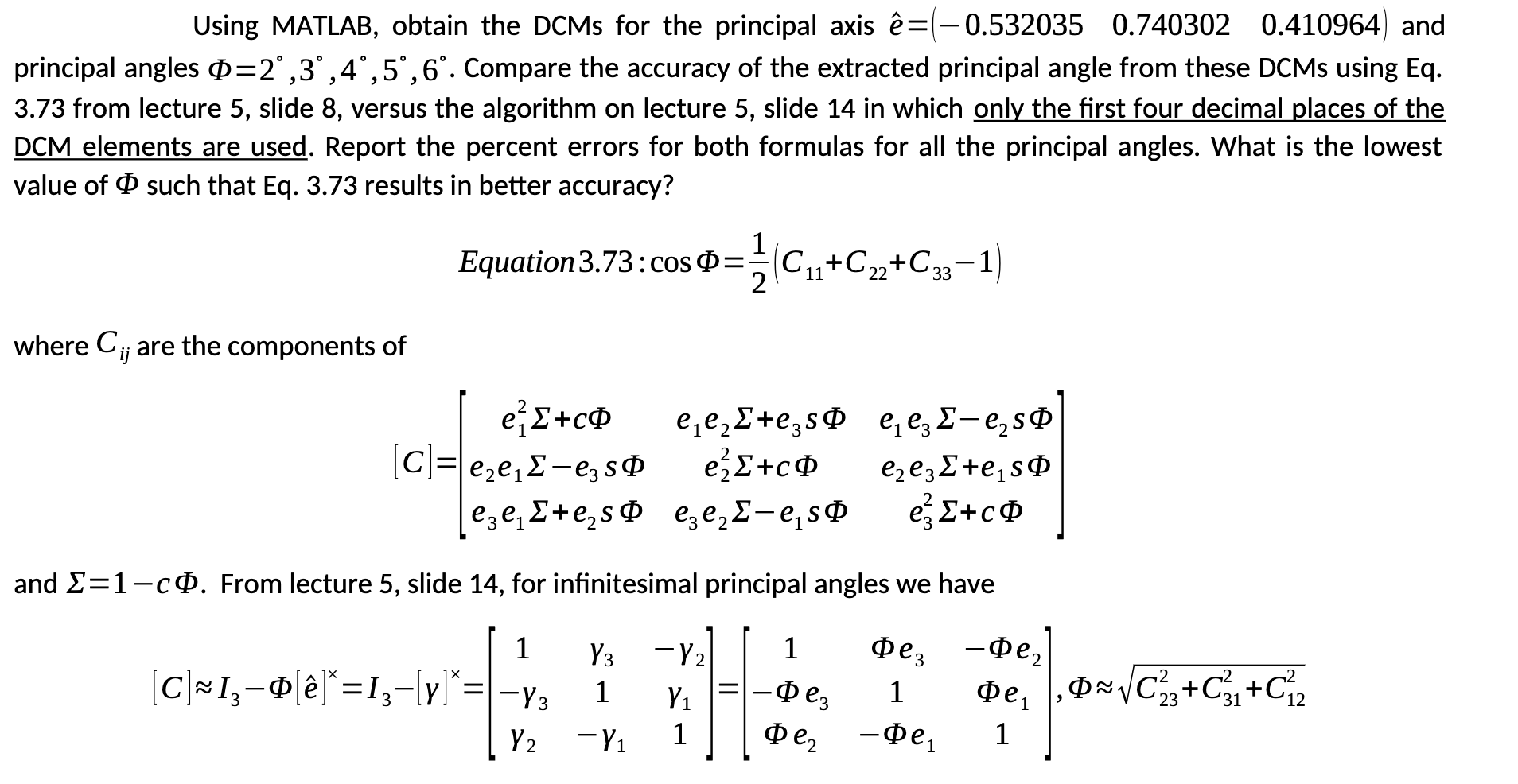
Compare the accuracy of the extracted principal angle from these DCMs using Eq. 3.73 from lecture 5, slide 8, versus the algorithm on lecture 5, slide 14 in which only the first four decimal places of the DCM elements are used. Report the percent errors for both formulas for all the principal angles. What is the lowest value of such that Eq. 3.73 results in better accuracy?

Using MATLAB, obtain the DCMs for the principal axis e^=(0.5320350.7403020.410964) and principal angles =2,3,4,5,6. Compare the accuracy of the extracted principal angle from these DCMs using Eq. 3.73 from lecture 5 , slide 8 , versus the algorithm on lecture 5 , slide 14 in which only the first four decimal places of the DCM elements are used. Report the percent errors for both formulas for all the principal angles. What is the lowest value of such that Eq. 3.73 results in better accuracy? Equation3.73:cos=21(C11+C22+C331) where Cij are the components of [C]=e12+ce2e1e3se3e1+e2se1e2+e3se22+ce3e2e1se1e3e2se2e3+e1se32+c and =1c. From lecture 5 , slide 14 , for infinitesimal principal angles we have [C]I3[e^]=I3[]=132311211=1e3e2e31e1e2e11,C232+C312+C122