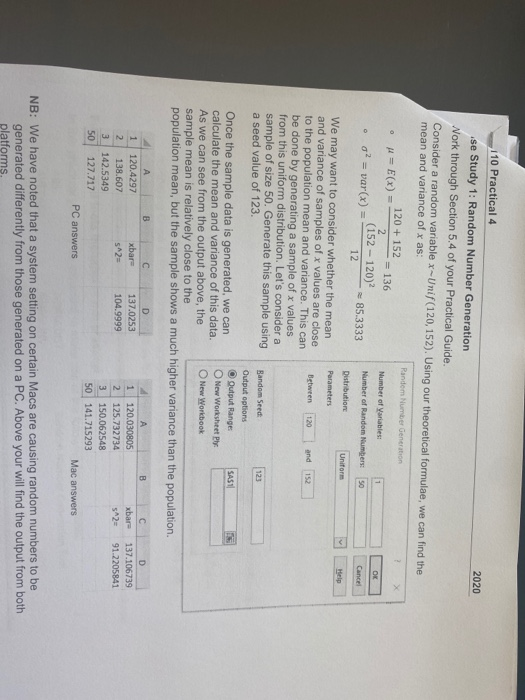
Compare the decision making process Scenario 1: You have to ensure that you are sustained and have sufficient food for the month. You have a fixed budget of R500. Outline how you will go about getting the groceries that you need. 110 Practical 4 2020 se Study 1: Random Number Generation Work through Section 5.4 of your Practical Guide. Consider a random variable x-Unif(120,152). Using our theoretical formulae, we can find the mean and variance of x as: Pandem Number Generation = E(x) = 120 + 152 = 136 Number of Variables = Var(x) = (152 - 1202 Number of Random Number: 50 - 85.3333 Distribution Uniform We may want to consider whether the mean Parameters and variance of samples of x values are close to the population mean and variance. This can Between 120 and 152 be done by generating a sample of x values from this uniform distribution. Let's consider a sample of size 50. Generate this sample using Bandom Seed: a seed value of 123. Output options Once the sample data is generated, we can Output Range: SASI calculate the mean and variance of this data. New Worksheet Phy As we can see from the output above, the O New Workbook sample mean is relatively close to the population mean, but the sample shows a much higher variance than the population. B 1 A 120.4297 138.607 142.5349 127.717 C xbar- S^2= D 137.0253 104.9999 C xbar 5^2= D 137.106739 91.2205841 3 50 1 120.030805 2 125.732734 3 150.062548 50 141.715293 PC answers Mac answers NB: We have noted that a system setting on certain Macs are causing random numbers to be generated differently from those generated on a PC. Above your will find the output from both platforms. Let x-N(50,16) = N(50,4). Generate a sample of size 100 from this distribution using a seed value of 1793 u= E(x) = [a] - 2 decimal places if rounding is required o = var(x) = [b] - 4 decimal places if rounding is required Compare the decision making process Scenario 1: You have to ensure that you are sustained and have sufficient food for the month. You have a fixed budget of R500. Outline how you will go about getting the groceries that you need. 110 Practical 4 2020 se Study 1: Random Number Generation Work through Section 5.4 of your Practical Guide. Consider a random variable x-Unif(120,152). Using our theoretical formulae, we can find the mean and variance of x as: Pandem Number Generation = E(x) = 120 + 152 = 136 Number of Variables = Var(x) = (152 - 1202 Number of Random Number: 50 - 85.3333 Distribution Uniform We may want to consider whether the mean Parameters and variance of samples of x values are close to the population mean and variance. This can Between 120 and 152 be done by generating a sample of x values from this uniform distribution. Let's consider a sample of size 50. Generate this sample using Bandom Seed: a seed value of 123. Output options Once the sample data is generated, we can Output Range: SASI calculate the mean and variance of this data. New Worksheet Phy As we can see from the output above, the O New Workbook sample mean is relatively close to the population mean, but the sample shows a much higher variance than the population. B 1 A 120.4297 138.607 142.5349 127.717 C xbar- S^2= D 137.0253 104.9999 C xbar 5^2= D 137.106739 91.2205841 3 50 1 120.030805 2 125.732734 3 150.062548 50 141.715293 PC answers Mac answers NB: We have noted that a system setting on certain Macs are causing random numbers to be generated differently from those generated on a PC. Above your will find the output from both platforms. Let x-N(50,16) = N(50,4). Generate a sample of size 100 from this distribution using a seed value of 1793 u= E(x) = [a] - 2 decimal places if rounding is required o = var(x) = [b] - 4 decimal places if rounding is required