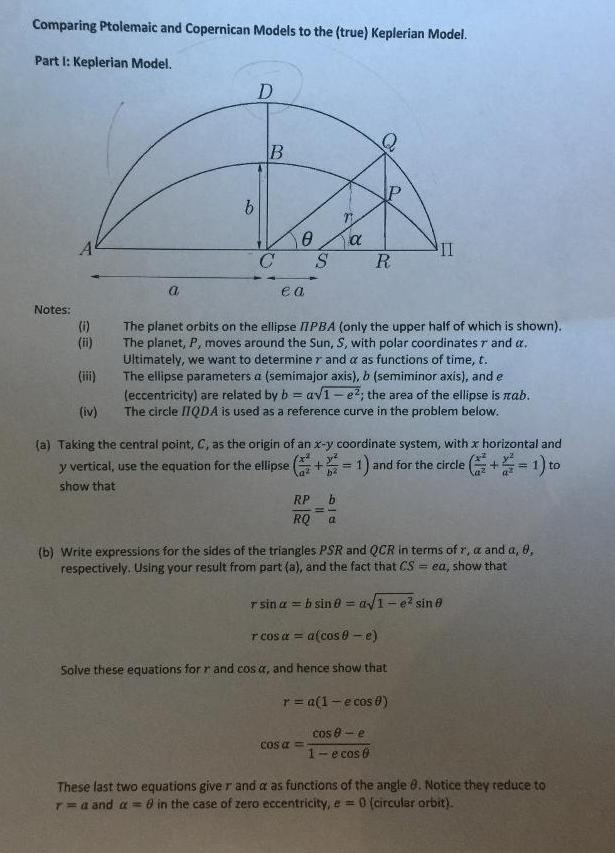
Comparing Ptolemaic and Copernican Models to the (true) Keplerian Model. Part I: Keplerian Model. D B a A II R e a Notes: (i) (fi) The planet orbits on the ellipse IPBA (only the upper half of which is shown). The planet, P, moves around the Sun, S, with polar coordinates r and a. Ultimately, we want to determine r and a as functions of time, t. The ellipse parameters a (semimajor axis), b (semiminor axis), and e (eccentricity) are related by b = av1- e2; the area of the ellipse is nab. The circle IIQDA is used as a reference curve in the problem below. (ii) (iv) (a) Taking the central point, C, as the origin of an x-y coordinate system, with x horizontal and y vertical, use the equation for the ellipse (+= 1) and for the circle to %3D %3D show that RP RQ (b) Write expressions for the sides of the triangles PSR and QCR in terms of r, a and a, 8, respectively, Using your result from part (a), and the fact that CS = ea, show that r sin a = b sin a = av1- e? sin e !! r cos a = a(cos 8 -e) Solve these equations for r and cos a, and hence show that r= a(1-e cos 0) Cos e-e 1-e cos 6 Cos a = These last two equations giver and a as functions of the angle 0. Notice they reduce to T=a and a=6 in the case of zero eccentricity, e= 0 (circular orbit). 10 (c) We now have equations for r and a in terms of 0, but what we want are equations for r and a in terms of the time, t. We will use Kepler's Area Law (equal areas swept out in equal times) to find a relationship between t and 0. First, convince yourself that, according to Kepler's Area Law, Area(IPS) nab where t is the time (starting at t = 0 when the planet is at the perihelion point, II), and T is the orbital period of the planet. Next, divide Area(IPS) into two pieces: Area(IIPS) = Area(SRP) + Area(IIPR) Express Area(SRP) in terms ofr and a, and then use your results from part (b) to show that Area(SRP) = 1. ab (cos 0- e) sin 6 Starting with your result from part (a), argue that Area(IPR) =Area(IIQR) =ab (8- cos e sin 6) a Briefly describe your reasoning for the first equality. For the second equality, you cannot assume IIQ is a straight line; instead, write Area(I1QR) = Area(I1QC)- Area(RQC), and use simple geometrical properties of the circle and the right triangle. Thus show that 2n - = 0 - e sin 0 Notice that 0 does not increase linearly with t, except when e = 0. In principle, we would like to solve this last equation for 0 as a function of t, and then substitute that into the last two equations in part (b) to get r and a as functions of t. In practice, this cannot be done in terms of elementary functions, so we will resort to approximations when e is small (e 1). You are not asked to do this; the answer, to second order in e, is: 1-e cos B + e? sin? B a a = + 2e sin +e2 sin 2B where B = 2nt/T increases linearly in time. The details of the calculation will be shown in the solutions for those who may be interested in trying to work it through themselves.