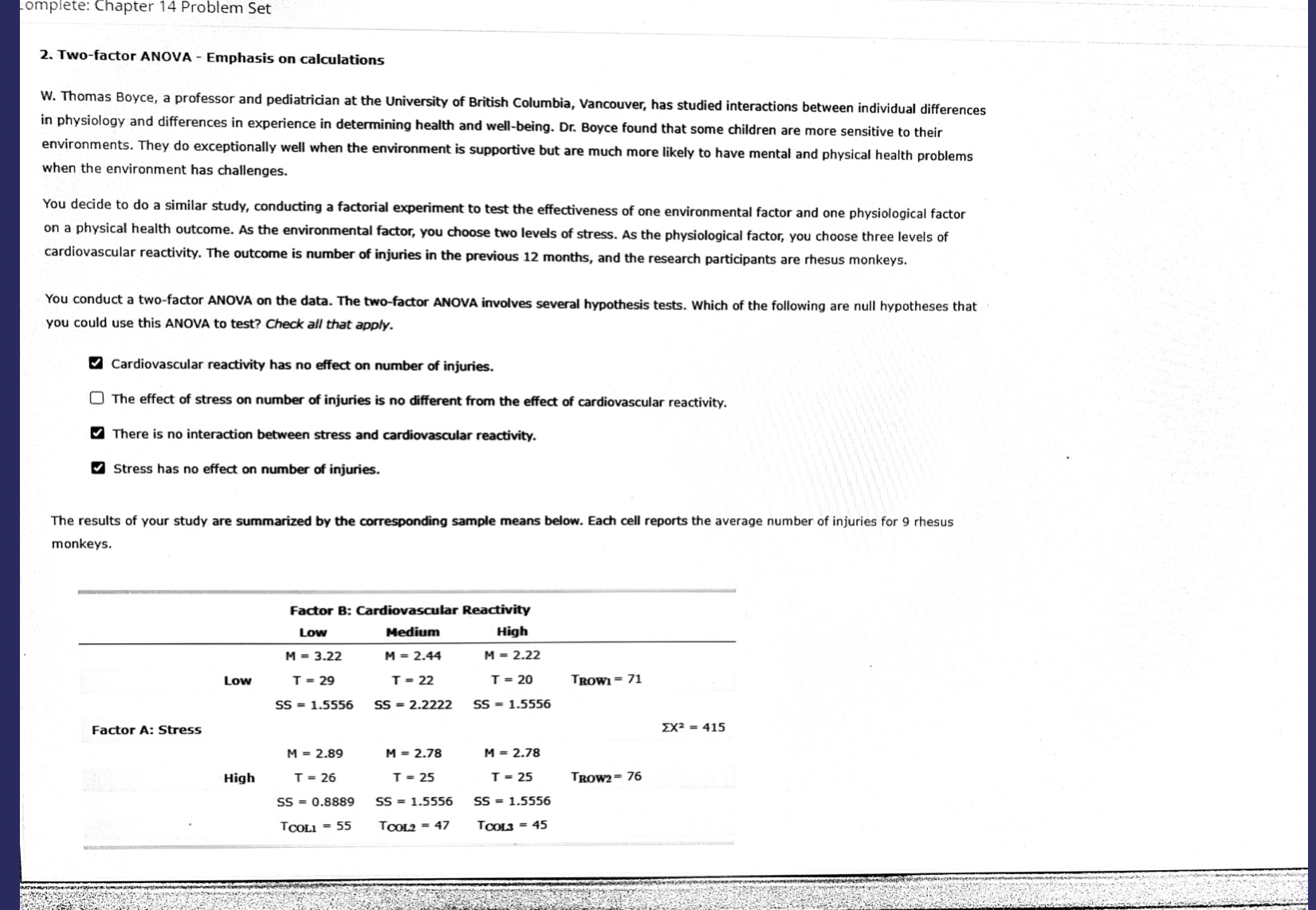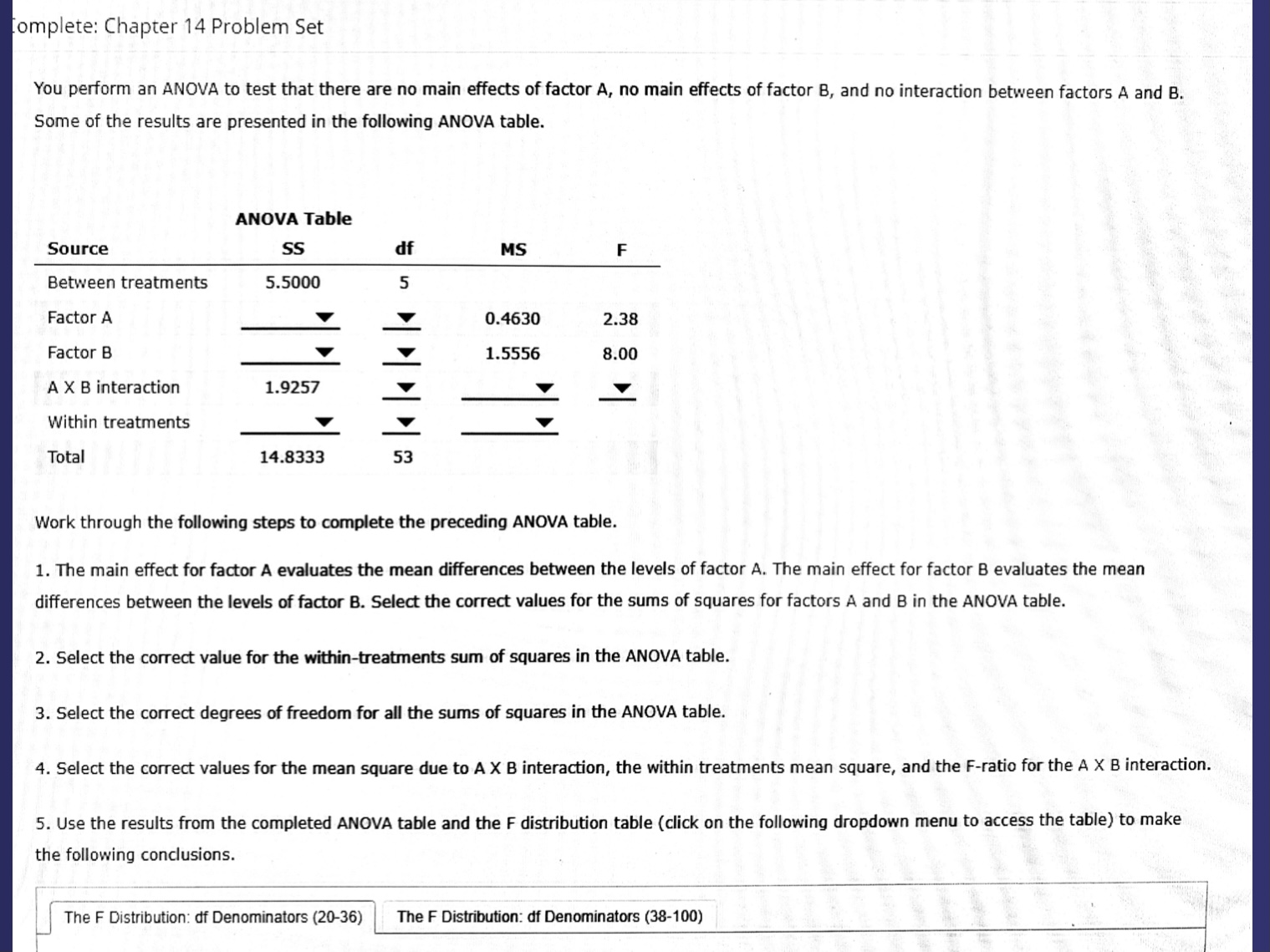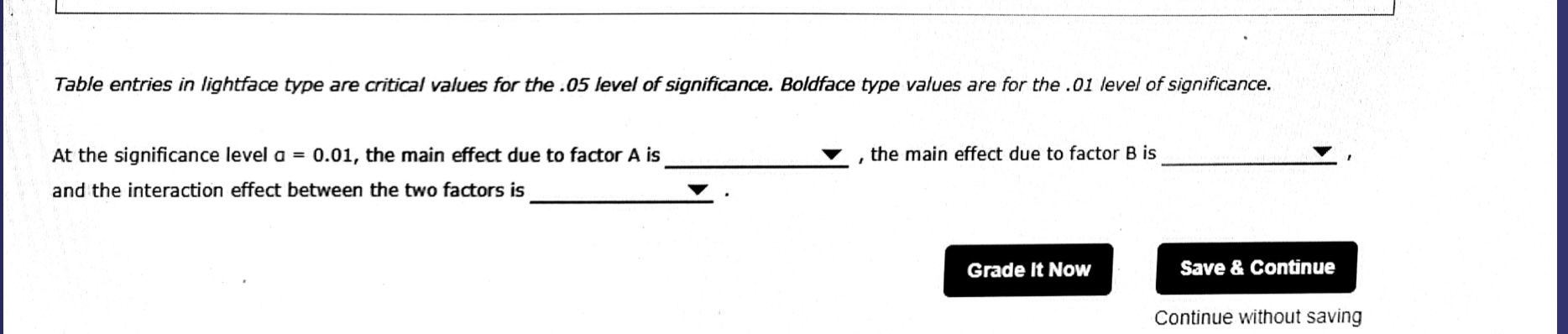
complete: Chapter 14 Problem Set 2. Two-factor ANOVA - Emphasis on calculations W. Thomas Boyce, a professor and pediatrician at the University of British Columbia, Vancouver, has studied interactions between individual differences in physiology and differences in experience in determining health and well-being. Dr. Boyce found that some children are more sensitive to their environments. They do exceptionally well when the environment is supportive but are much more likely to have mental and physical health problems when the environment has challenges. You decide to do a similar study, conducting a factorial experiment to test the effectiveness of one environmental factor and one physiological factor on a physical health outcome. As the environmental factor, you choose two levels of stress. As the physiological factor, you choose three levels of cardiovascular reactivity. The outcome is number of injuries in the previous 12 months, and the research participants are rhesus monkeys. You conduct a two-factor ANOVA on the data. The two-factor ANOVA involves several hypothesis tests. Which of the following are null hypotheses that you could use this ANOVA to test? Check all that apply. Cardiovascular reactivity has no effect on number of injuries. O The effect of stress on number of injuries is no different from the effect of cardiovascular reactivity. There is no interaction between stress and cardiovascular reactivity. Stress has no effect on number of injuries. The results of your study are summarized by the corresponding sample means below. Each cell reports the average number of injuries for 9 rhesus monkeys. Factor B: Cardiovascular Reactivity Low Medium High M = 3.22 M = 2.44 M = 2.22 Low T = 29 T = 22 T = 20 TROWI = 71 SS = 1.5556 SS = 2.2222 SS = 1.5556 Factor A: Stress EX2 = 415 M = 2.89 M = 2.78 M = 2.78 High T = 26 T = 25 T - 25 TROW2 = 76 SS = 0.8889 SS = 1.5556 SS = 1.5556 TCOLI = 55 TOOL2 = 47 TOOL3 = 45omplete: Chapter 14 Problem Set You perform an ANOVA to test that there are no main effects of factor A, no main effects of factor B, and no interaction between factors A and B. Some of the results are presented in the following ANOVA table. ANOVA Table Source SS df MS F Between treatments 5.5000 5 Factor A 0.4630 2.38 Factor B 1.5556 8.00 A X B interaction 1.9257 Y Within treatments Total 14.8333 53 Work through the following steps to complete the preceding ANOVA table. 1. The main effect for factor A evaluates the mean differences between the levels of factor A. The main effect for factor B evaluates the mean differences between the levels of factor B. Select the correct values for the sums of squares for factors A and B in the ANOVA table. 2. Select the correct value for the within-treatments sum of squares in the ANOVA table. 3. Select the correct degrees of freedom for all the sums of squares in the ANOVA table. 4. Select the correct values for the mean square due to A X B interaction, the within treatments mean square, and the F-ratio for the A X B interaction. 5. Use the results from the completed ANOVA table and the F distribution table (click on the following dropdown menu to access the table) to make the following conclusions. The F Distribution: df Denominators (20-36) The F Distribution: df Denominators (38-100)Table entries in lightface type are critical values for the .05 level of significance. Boldface type values are for the . 01 level of significance. At the significance level a = 0.01, the main effect due to factor A is , the main effect due to factor B is and the interaction effect between the two factors is Grade It Now Save & Continue Continue without saving