Computer Science Data Science.
I will give thumb up, Thank you!
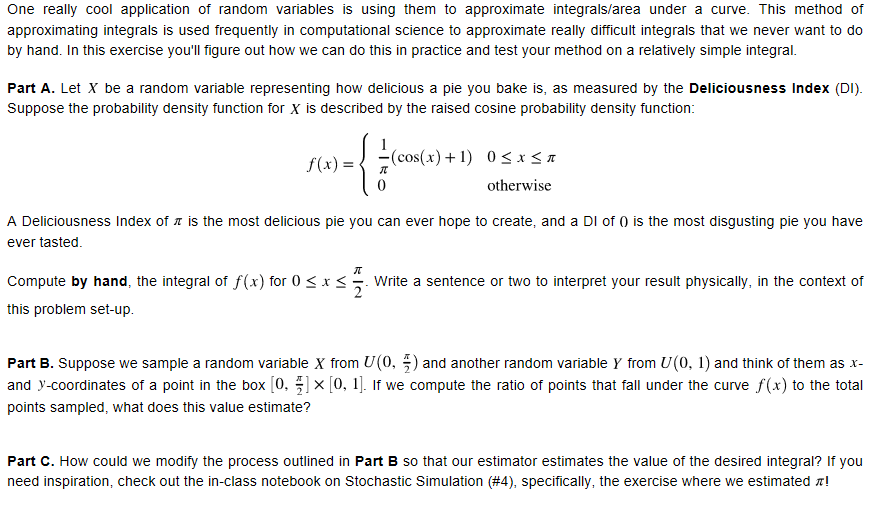
One really cool application of random variables is using them to approximate integrals/area under a curve. This method of approximating integrals is used frequently in computational science to approximate really difficult integrals that we never want to do by hand. In this exercise you'll figure out how we can do this in practice and test your method on a relatively simple integral. Part A. Let X be a random variable representing how delicious a pie you bake is, as measured by the Deliciousness Index (DI). Suppose the probability density function for X is described by the raised cosine probability density function: f(x)=12(cos(x) + 1) OSxs+ 0 otherwise A Deliciousness index of is the most delicious pie you can ever hope to create, and a DI of 0 is the most disgusting pie you have ever tasted. Compute by hand, the integral of f(x) for 0 this problem set-up. x , write a sentence or two to interpret your result physically, in the context of Part B. Suppose we sample a random variable X from U(0, and another random variable Y from U(0, 1) and think of them as x- and y-coordinates of a point in the box [O] x0, i]. If we compute the ratio of points that fall under the curve f(x) to the total points sampled, what does this value estimate? Part C. How could we modify the process outlined in Part B so that our estimator estimates the value of the desired integral? If you (#4), specifically, the exercise where we One really cool application of random variables is using them to approximate integrals/area under a curve. This method of approximating integrals is used frequently in computational science to approximate really difficult integrals that we never want to do by hand. In this exercise you'll figure out how we can do this in practice and test your method on a relatively simple integral. Part A. Let X be a random variable representing how delicious a pie you bake is, as measured by the Deliciousness Index (DI). Suppose the probability density function for X is described by the raised cosine probability density function: f(x)=12(cos(x) + 1) OSxs+ 0 otherwise A Deliciousness index of is the most delicious pie you can ever hope to create, and a DI of 0 is the most disgusting pie you have ever tasted. Compute by hand, the integral of f(x) for 0 this problem set-up. x , write a sentence or two to interpret your result physically, in the context of Part B. Suppose we sample a random variable X from U(0, and another random variable Y from U(0, 1) and think of them as x- and y-coordinates of a point in the box [O] x0, i]. If we compute the ratio of points that fall under the curve f(x) to the total points sampled, what does this value estimate? Part C. How could we modify the process outlined in Part B so that our estimator estimates the value of the desired integral? If you (#4), specifically, the exercise where we







