Question
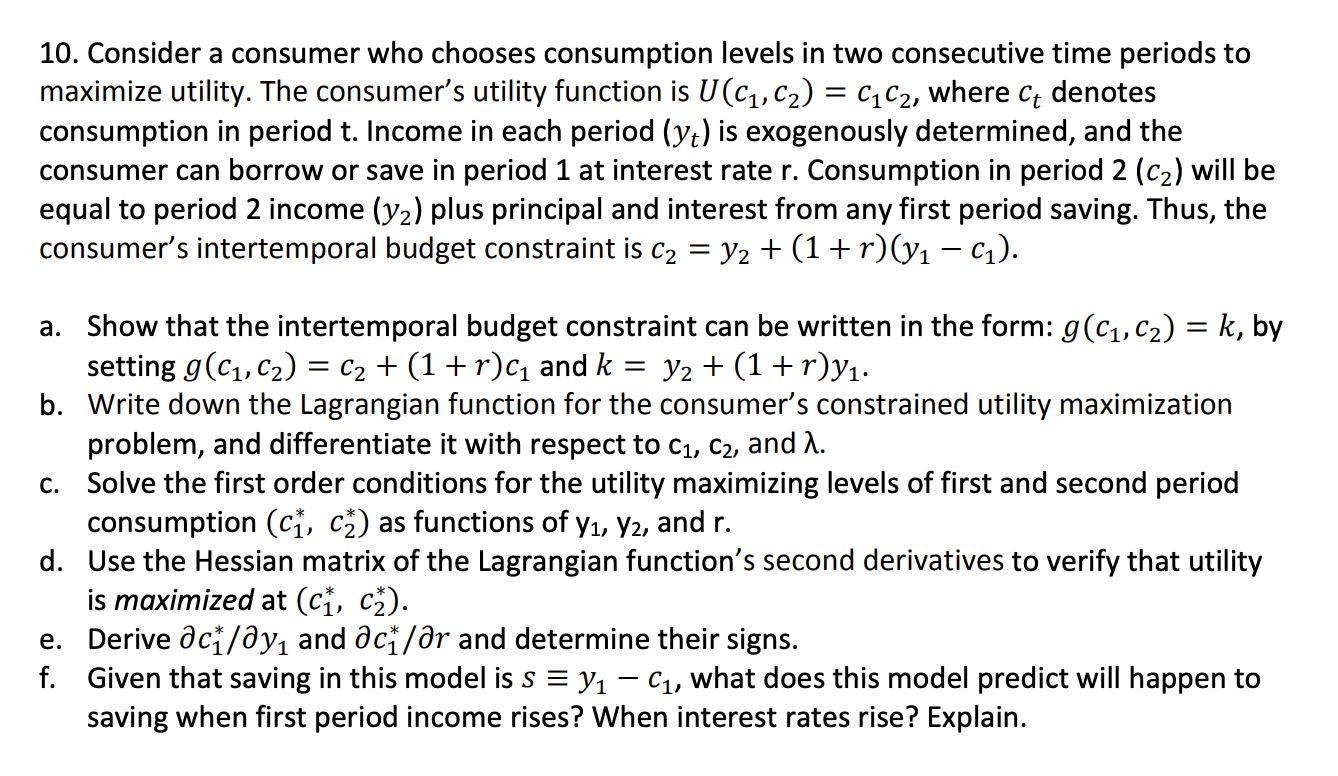
Consider a consumer who chooses consumption levels in two consecutive time periods to maximize utility. The consumer's utility function is ?(?_1, ?_2 ) = (?_1)(?_2),
Consider a consumer who chooses consumption levels in two consecutive time periods to maximize utility. The consumer's utility function is ?(?_1, ?_2 ) = (?_1)(?_2), where ?_? denotes consumption in period t. Income in each period (?_? ) is exogenously determined, and the consumer can borrow or save in period 1 at interest rate r. Consumption in period 2 (?_2) will be equal to period 2 income (?_2) plus principal and interest from any first period saving. Thus, the consumer's intertemporal budget constraint is ?_2 = ?_2 + (1 + ?)(?_1 ? ?_1 ).
a. Show that the intertemporal budget constraint can be written in the form: ?(?_1, ?_2) = ?, by setting ?(?_1, ?_2 ) = ?_2 + (1 + ?)?_1 and ? = ?_2 + (1 + ?)?_1.
b. Write down the Lagrangian function for the consumer's constrained utility maximization problem, and differentiate it with respect to c_1, c_2, and ?.
c. Solve the first order conditions for the utility maximizing levels of first and second period consumption (?_1* , ?_2*) as functions of y_1, y_2, and r.
d. Use the Hessian matrix of the Lagrangian function's second derivatives to verify that utility is maximized at (?_1* , ?_2* ).
e. Derive ??_1*/??1 and ??_1*/?? and determine their signs.
f. Given that saving in this model is ? ? ?_1 ? ?_
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


