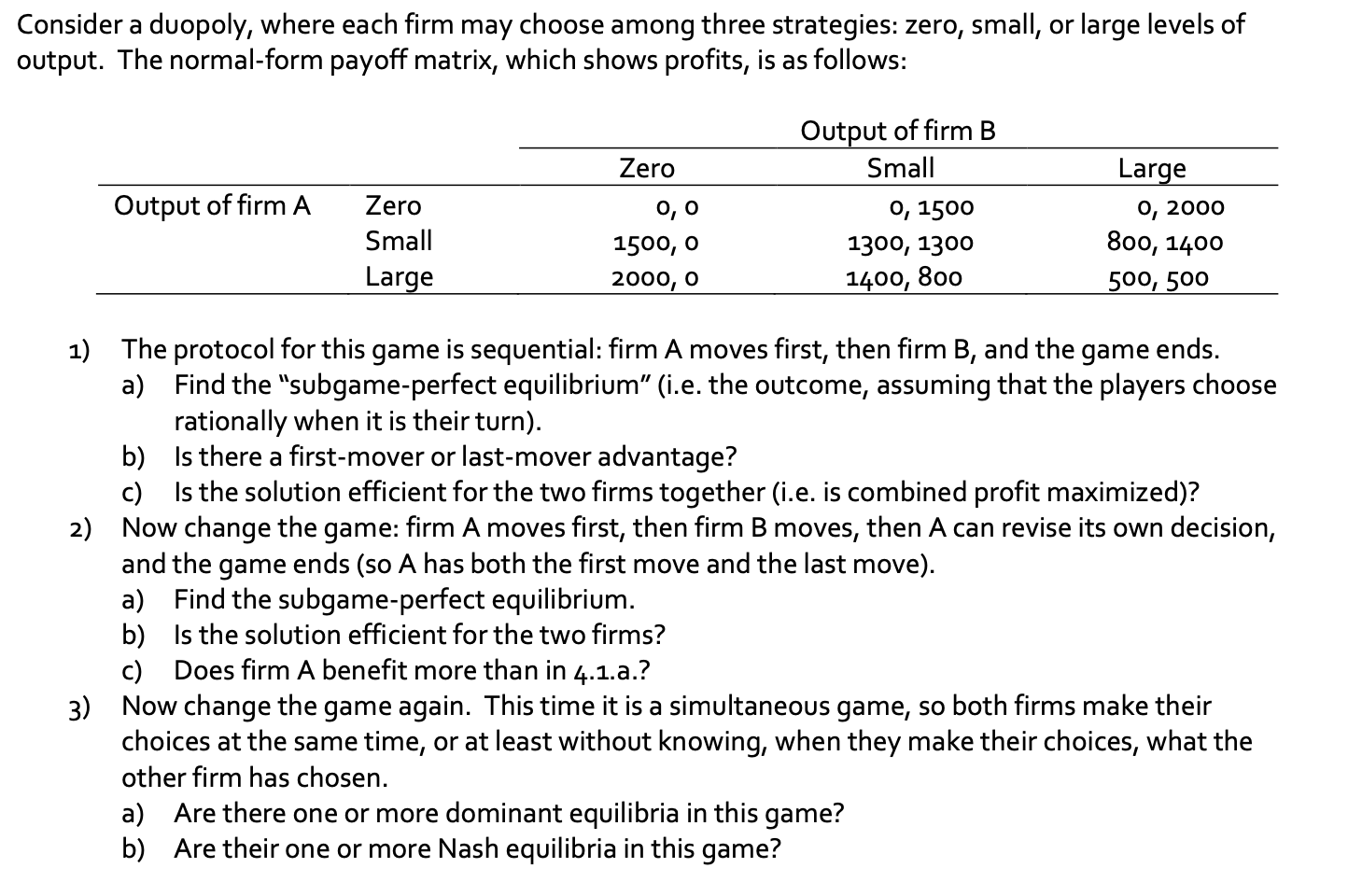
Consider a duopoly, where each firm may choose among three strategies: zero, small, or large levels of sutput. The normal-form payoff matrix, which shows profits, is as follows: 1) The protocol for this game is sequential: firm A moves first, then firm B, and the game ends. a) Find the "subgame-perfect equilibrium" (i.e. the outcome, assuming that the players choose rationally when it is their turn). b) Is there a first-mover or last-mover advantage? c) Is the solution efficient for the two firms together (i.e. is combined profit maximized)? 2) Now change the game: firm A moves first, then firm B moves, then A can revise its own decision, and the game ends (so A has both the first move and the last move). a) Find the subgame-perfect equilibrium. b) Is the solution efficient for the two firms? c) Does firm A benefit more than in 4.1.a.? 3) Now change the game again. This time it is a simultaneous game, so both firms make their choices at the same time, or at least without knowing, when they make their choices, what the other firm has chosen. a) Are there one or more dominant equilibria in this game? b) Are their one or more Nash equilibria in this game? Consider a duopoly, where each firm may choose among three strategies: zero, small, or large levels of sutput. The normal-form payoff matrix, which shows profits, is as follows: 1) The protocol for this game is sequential: firm A moves first, then firm B, and the game ends. a) Find the "subgame-perfect equilibrium" (i.e. the outcome, assuming that the players choose rationally when it is their turn). b) Is there a first-mover or last-mover advantage? c) Is the solution efficient for the two firms together (i.e. is combined profit maximized)? 2) Now change the game: firm A moves first, then firm B moves, then A can revise its own decision, and the game ends (so A has both the first move and the last move). a) Find the subgame-perfect equilibrium. b) Is the solution efficient for the two firms? c) Does firm A benefit more than in 4.1.a.? 3) Now change the game again. This time it is a simultaneous game, so both firms make their choices at the same time, or at least without knowing, when they make their choices, what the other firm has chosen. a) Are there one or more dominant equilibria in this game? b) Are their one or more Nash equilibria in this game