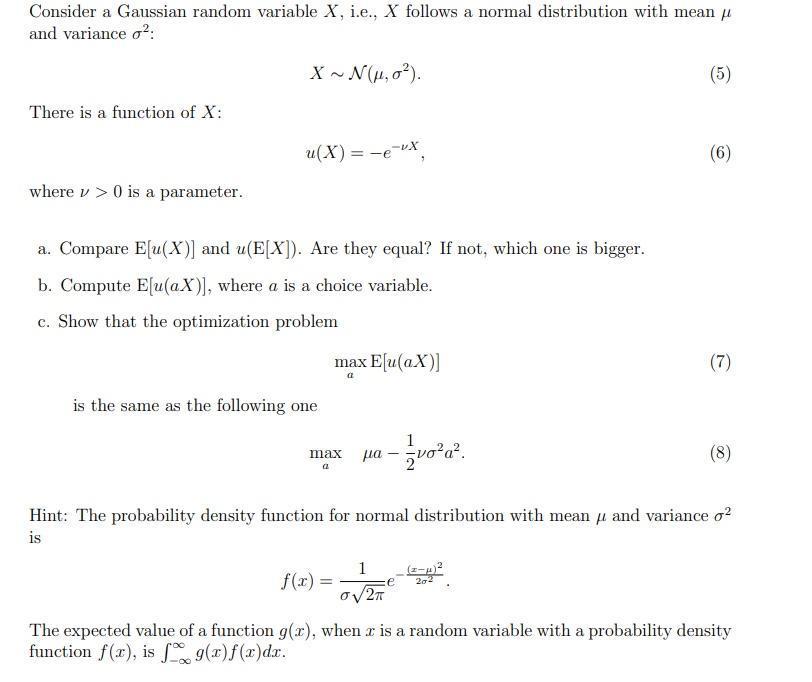
Consider a Gaussian random variable X, i.e., X follows a normal distribution with mean and variance o: There is a function of X: wherev> 0 is a parameter. X~ N(0). u(X) = eux, a. Compare E[u(X)] and u(E[X]). Are they equal? If not, which one is bigger. b. Compute E[u(aX)], where a is a choice variable. c. Show that the optimization problem is the same as the following one max E[u(aX)] a max a f(x) = a -voa. - (5) 1 O2 (6) (7) Hint: The probability density function for normal distribution with mean and variance 0 is (8) The expected value of a function g(x), when r is a random variable with a probability density function f(x), is f g(x)f(x)dx.