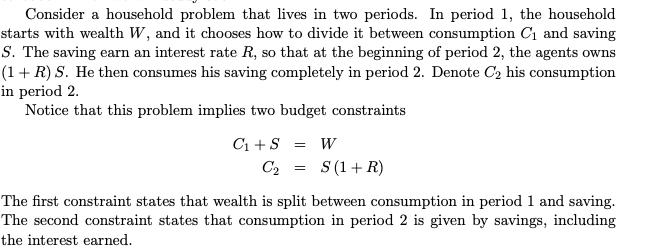
Consider a household problem that lives in two periods. In period 1, the household starts with wealth W, and it chooses how to divide it between consumption C and saving S. The saving earn an interest rate R, so that at the beginning of period 2, the agents owns (1 + R) S. He then consumes his saving completely in period 2. Denote C his consumption in period 2. Notice that this problem implies two budget constraints C + S = W C = S (1 + R) The first constraint states that wealth is split between consumption in period 1 and saving. The second constraint states that consumption in period 2 is given by savings, including the interest earned. Question 1.2 Now consider the utility of the household. Let us assume that the utility takes the form U = In C + 3 ln C where is the time preference coefficient. 1 Use the intertemporal budget constraint to substitute out C from the utility U. Then write down the first-order condition for maximization of U with respect to C. Notice that the first-order condition contains the term W-, which is equal to C. Substitute C back and show that you can write the first-order condition as 1 C This equation is called the consumption Euler equation. 1 = 8 (1 + R) (1)