.

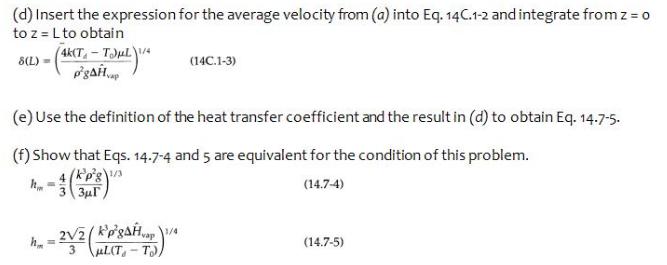
Consider a laminar film of condensate flowing down a vertical wall, and assume that this liquid film constitutes the sole heat transfer resistance on the vapor side of the wall. Further assume that (i) the shear stress between liquid and vapor may be neglected; (ii) the physical properties in the film may be evaluated at the arithmetic mean of vapor and cooling surface temperatures and that the cooling-surface temperature may be assumed constant; (iii) acceleration of fluidelements in the film may be neglected compared to the gravitational and viscous forces; (iv) sensible heat changes, CpdT, in the condensate film are unimportant compared to the latent heat transferred through it; and (v) the heat flux is very nearly normal to the wall surface. (a) Recall from $2.2 that the average velocity of a film of constant thickness is average v, = pg8:|34. Assume that this relation is valid for any value of z. (b) Write the energy equation for the film, neglecting film curvature and convection. Show that the heat flux through the film toward the cold surface is (T.-T. (14C.1-1) (c)As the film proceeds down the wall, it picks up additional material by the condensation process. In this process, heat is liberated to the extent of AHvap per unitmass of material that undergoes the change in state. Show that equating the heat liberatio nby condensation with the heat flowing through the film ina segment dz of the film leads to (T.-To dz = kl (14C.1-2)