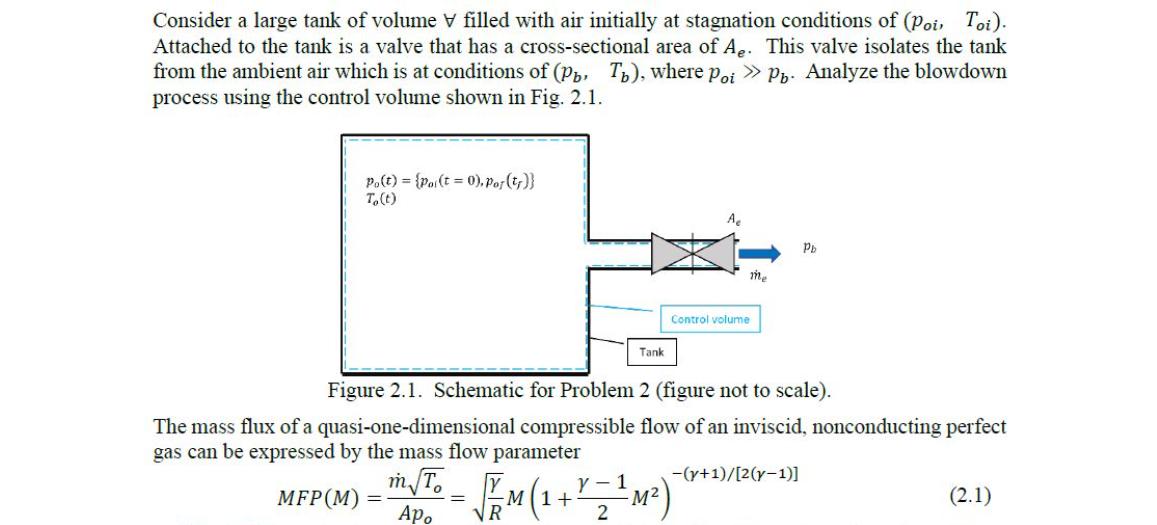
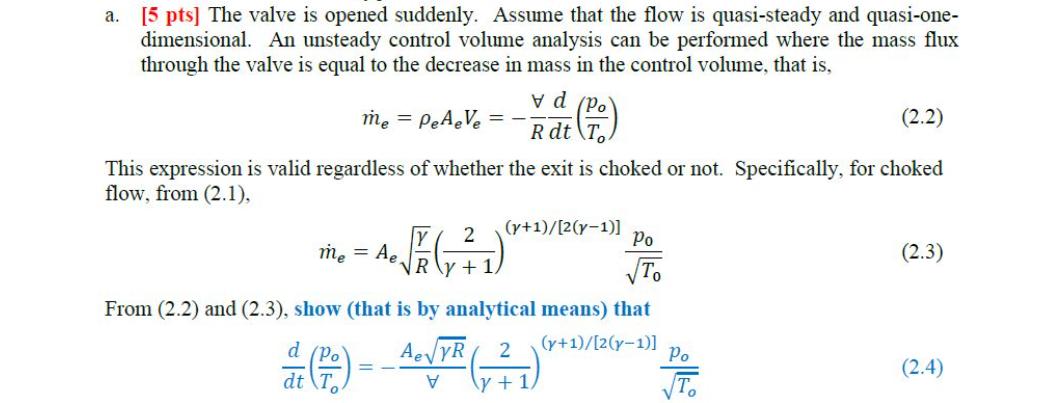
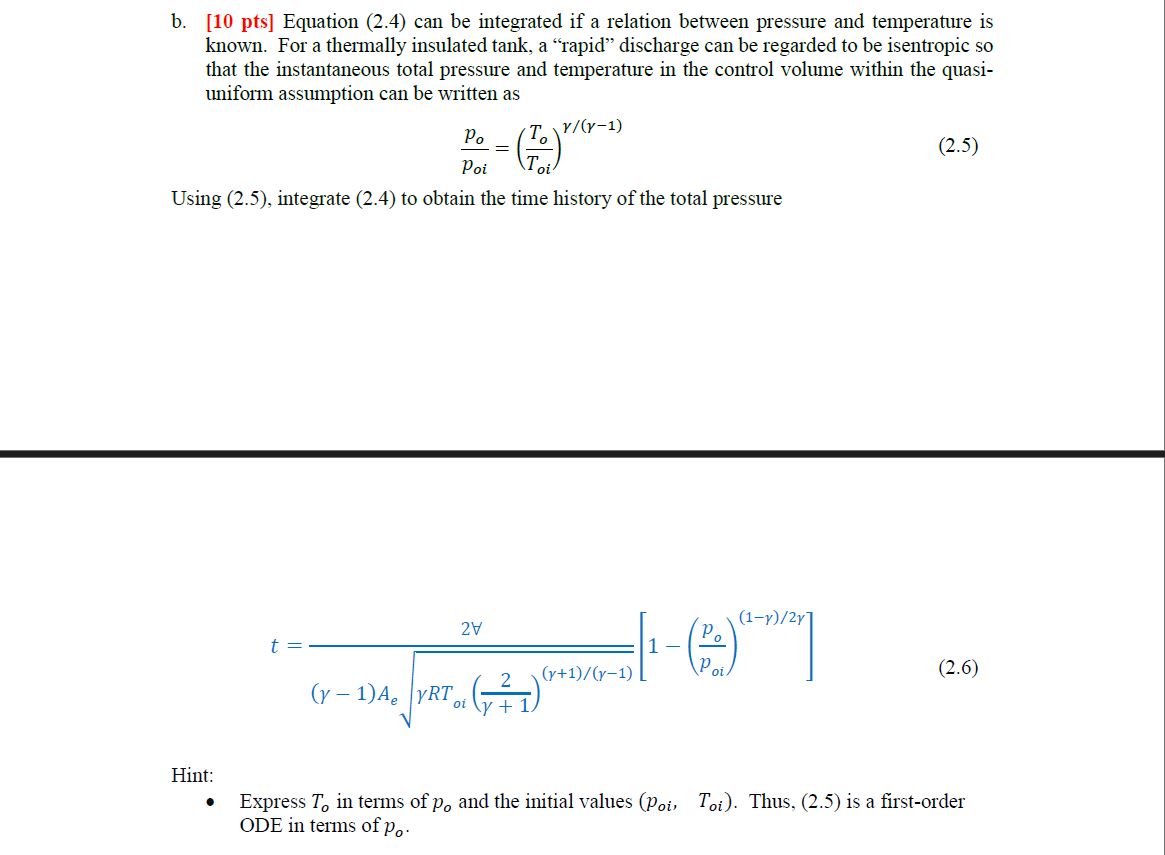
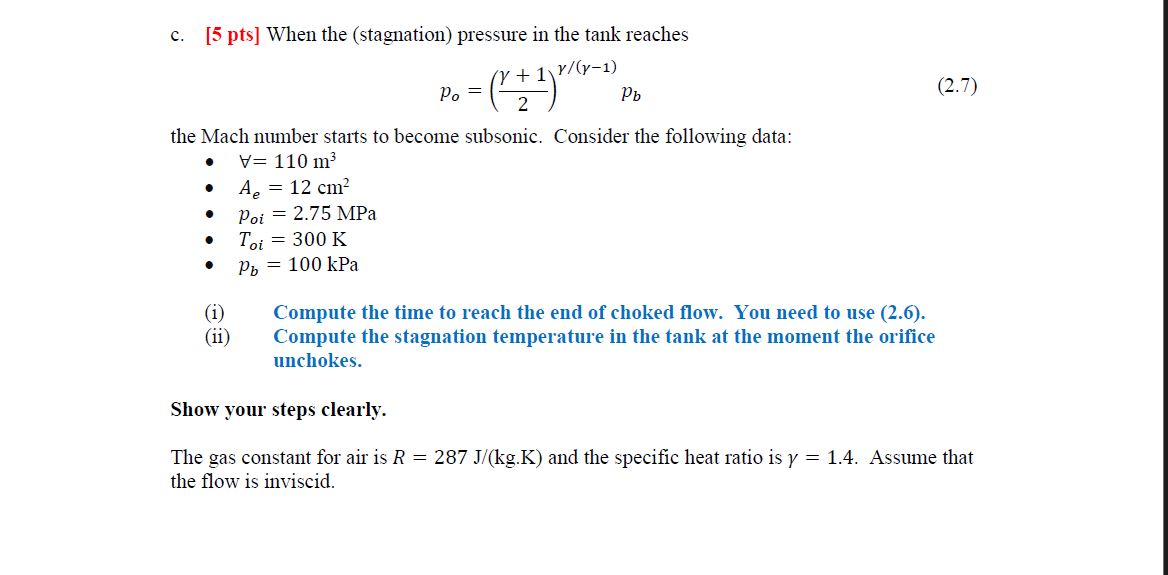
Consider a large tank of volume V filled with air initially at stagnation conditions of (Poi, Toi). Attached to the tank is a valve that has a cross-sectional area of Ag. This valve isolates the tank from the ambient air which is at conditions of (Pb. Tb), where poi Pb. Analyze the blowdown process using the control volume shown in Fig. 2.1. Po(t) = {Poi(t = 0), Por(tr)} T(t) MFP (M) Tank Ae Control volume me Pb Figure 2.1. Schematic for Problem 2 (figure not to scale). The mass flux of a quasi-one-dimensional compressible flow of an inviscid, nonconducting perfect gas can be expressed by the mass flow parameter -(y+1)/[2(y-1)] 1 To = F M (1 + = M) Y 1 = Apo NR 2 (2.1) a. [5 pts] The valve is opened suddenly. Assume that the flow is quasi-steady and quasi-one- dimensional. An unsteady control volume analysis can be performed where the mass flux through the valve is equal to the decrease in mass in the control volume, that is, me = Pe AeVe (2.2) This expression is valid regardless of whether the exit is choked or not. Specifically, for choked flow, from (2.1), == = 5 / (+2+12) R\y 1, me = Ae. d dt T Po Rdt To Po To From (2.2) and (2.3), show (that is by analytical means) that (y+1)/[2(y-1)] (P)-AYR (2) 1 (y+1)/[2(y-1)] Po To (2.3) (2.4) b. [10 pts] Equation (2.4) can be integrated if a relation between pressure and temperature is known. For a thermally insulated tank, a "rapid" discharge can be regarded to be isentropic so that the instantaneous total pressure and temperature in the control volume within the quasi- uniform assumption can be written as To Toi Using (2.5), integrate (2.4) to obtain the time history of the total pressure Hint: t Po Poi 20 - Y/(x-1) 2 (y-1)A YRT oi y + 1/ (y+1)/(x-1) Pa [-(2) 1 P oi (1-y)/2y] (2.5) (2.6) Express To in terms of p, and the initial values (Poi, Toi). Thus, (2.5) is a first-order ODE in terms of po. c. [5 pts] When the (stagnation) pressure in the tank reaches (Y+1\Y/(x-1) 2 the Mach number starts to become subsonic. Consider the following data: V 110 m (1) (11) A = 12 cm Poi 2.75 MPa Toi = 300 K Pb = 100 kPa Po = Pb (2.7) Compute the time to reach the end of choked flow. You need to use (2.6). Compute the stagnation temperature in the tank at the moment the orifice unchokes. Show your steps clearly. The gas constant for air is R = 287 J/(kg.K) and the specific heat ratio is y = 1.4. Assume that the flow is inviscid.