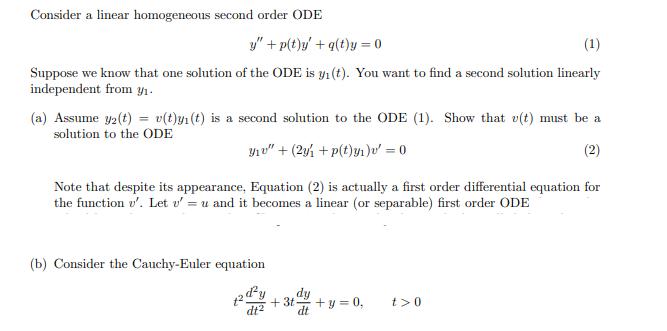
Consider a linear homogeneous second order ODE y" +p(t)y' +q(t)y=0 (1) Suppose we know that one solution of the ODE is yi (t). You want to find a second solution linearly independent from 3. (a) Assume y2(t) = v(t)yi (t) is a second solution to the ODE (1). Show that u(t) must be a solution to the ODE yv" + (2y + p(t) y) v' = 0 (2) Note that despite its appearance, Equation (2) is actually a first order differential equation for the function v'. Let v' = u and it becomes a linear (or separable) first order ODE (b) Consider the Cauchy-Euler equation dt2 +3t dy dt+y=0, t> 0 (ii) Use the method of reduction of order described in part (a) and find a second solution 32 for the ODE. (Note that in the method of reduction of order the coefficient of y" is 1.)