Question
Consider a scenario in which we have three cells as illustrated below. A and B are adjacent; B and C are adjacent. A user can
Consider a scenario in which we have three cells as illustrated below. A and B are adjacent; B and C are adjacent. A user can not go from A to C without crossing B. Suppose that we have about 250 users on average in each cell and each user has a chance of 0.2 to get a call in one hour. On average within one hour we have about 50 users who cross the border between A, B. Similarly, on average 50 users cross the border between B, C in an hour. (Even with people moving we still assume that the average number of people in each cell stays the same, 200. Suppose these users do not move outside the three cells.) The cost of each location update is 1. The cost of paging is equivalent to the number of cells one needs to check. Now we want to minimize the total cost as the sum of the paging and location update costs. Please decide how to partition them into location areas such that total cost is the lowest (you need to decide how many location areas you use and how the cells are grouped).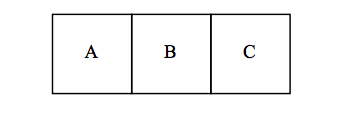
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


