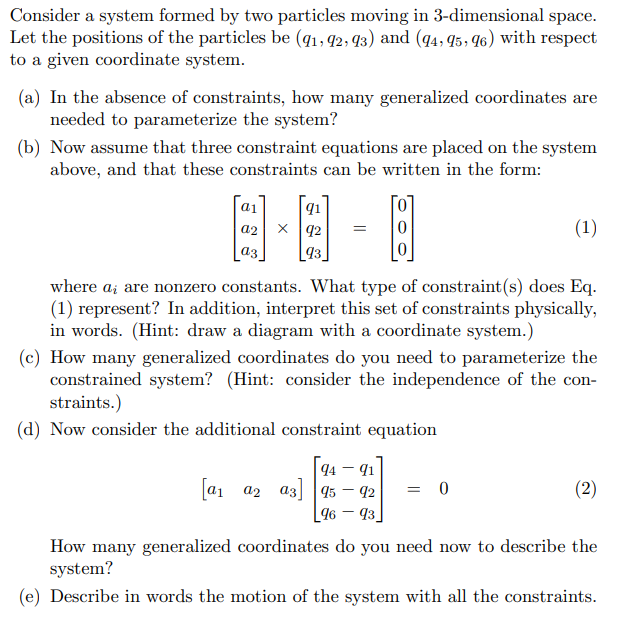
Consider a system formed by two particles moving in 3-dimensional space. Let the positions of the particles be (91, 92, 93) and (94, 95, 96) with respect to a given coordinate system. (a) In the absence of constraints, how many generalized coordinates are needed to parameterize the system? (b) Now assume that three constraint equations are placed on the system above, and that these constraints can be written in the form: a1 91 a2 92 = [93_ (1) where a; are nonzero constants. What type of constraint(s) does Eq. (1) represent? In addition, interpret this set of constraints physically, in words. (Hint: draw a diagram with a coordinate system.) (c) How many generalized coordinates do you need to parameterize the constrained system? (Hint: consider the independence of the con- straints.) (d) Now consider the additional constraint equation 94-91 [a1 a2 03] 95-92 = 0 9693 (2) How many generalized coordinates do you need now to describe the system? (e) Describe in words the motion of the system with all the constraints.