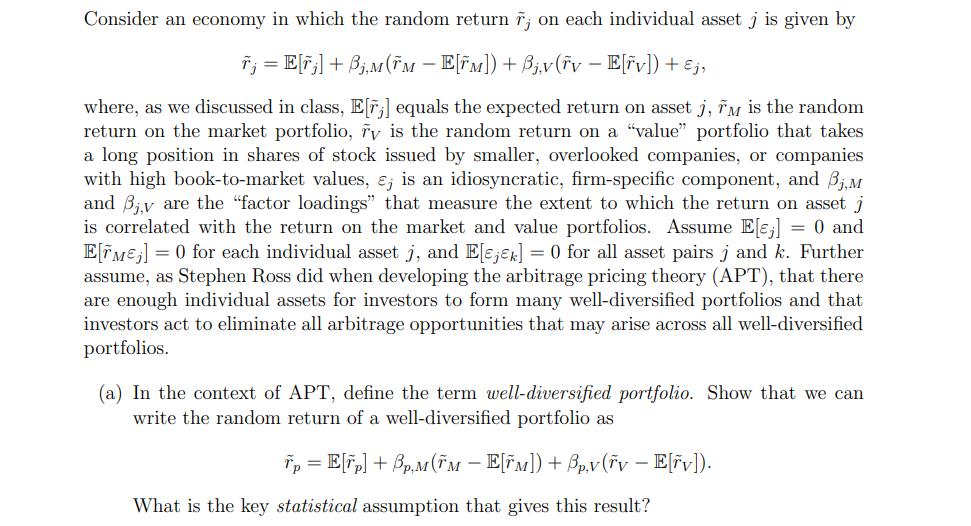
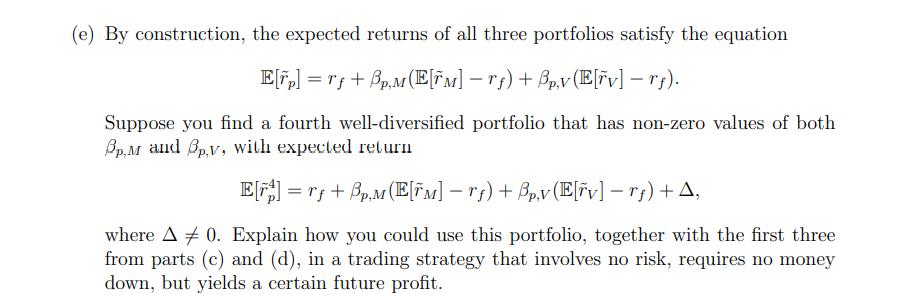
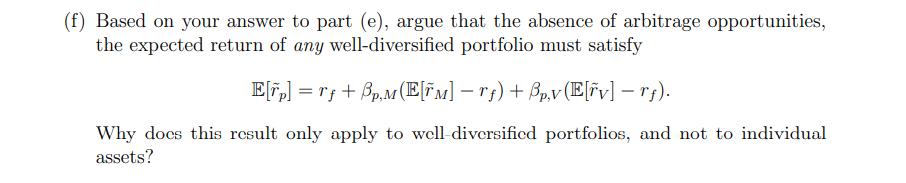
Consider an economy in which the random return r; on each individual asset j is given by T = E[rj] + B,M (FM - E[M]) + B,v(rv - E[rv]) + Ej, where, as we discussed in class, E[7] equals the expected return on asset j, TM is the random return on the market portfolio, ry is the random return on a "value" portfolio that takes a long position in shares of stock issued by smaller, overlooked companies, or companies with high book-to-market values, &;, is an idiosyncratic, firm-specific component, and B.M and By are the "factor loadings" that measure the extent to which the return on asset j is correlated with the return on the market and value portfolios. Assume E[;] = 0 and E[ME] =0 for each individual asset j, and E[jk] = 0 for all asset pairs j and k. Further assume, as Stephen Ross did when developing the arbitrage pricing theory (APT), that there are enough individual assets for investors to form many well-diversified portfolios and that investors act to eliminate all arbitrage opportunities that may arise across all well-diversified portfolios. (a) In the context of APT, define the term well-diversified portfolio. Show that we can write the random return of a well-diversified portfolio as Tp = E[p] + Bp.M (FM - E[M]) + Bp.v(Tv - E[rv]). What is the key statistical assumption that gives this result? (b) Consider two well-diversified portfolios such that: = E[]+Bp.M (FM - E[M]) + Bp.v(Tv - E[rv]), E[]+BP.M(ME[M]) + Bp.v(Tv - E[rv]), - meaning they have the same factor loadings on the market and value portfolios. Sup- pose that E[] = E[] + A. Show the absence of arbitrage opportunities requires that A = 0. (c) Now consider a well-diversified portfolio that has p.M = Bp.v = 0. Write down the equation, implied by this version of the APT, that links the expected return E[] of this portfolio to the return rf on a portfolio of risk-free assets. (d) Next, consider two more well-diversified portfolios: portfolio 2, with 3p,M = 1 and Bp.v = 0, and portfolio 3, with p,M = 0 and 3p,v = 1. Write down the equations implied by this version of the APT, that link the expected returns E[] and E[7] on each of these two portfolios to E[M] and E[v]. (e) By construction, the expected returns of all three portfolios satisfy the equation E[p] = Tf+Pp.M (E[M] - rf) + Bp.v (E[v] - 1 -rf). Suppose you find fourth well-diversified portfolio that has non-zero values of both Bp.M and Bp.v, with expected return E[r]=rf + Bp.M (E[M] - rf) + pv (E[v] - r) + A, where A 0. Explain how you could use this portfolio, together with the first three from parts (c) and (d), in a trading strategy that involves no risk, requires no money down, but yields a certain future profit. (f) Based on your answer to part (e), argue that the absence of arbitrage opportunities, the expected return of any well-diversified portfolio must satisfy E[p] = rf + Bp.M (E[M] -rf) + Bp,v (E[v] - rf). Why does this result only apply to well-diversified portfolios, and not to individual assets?