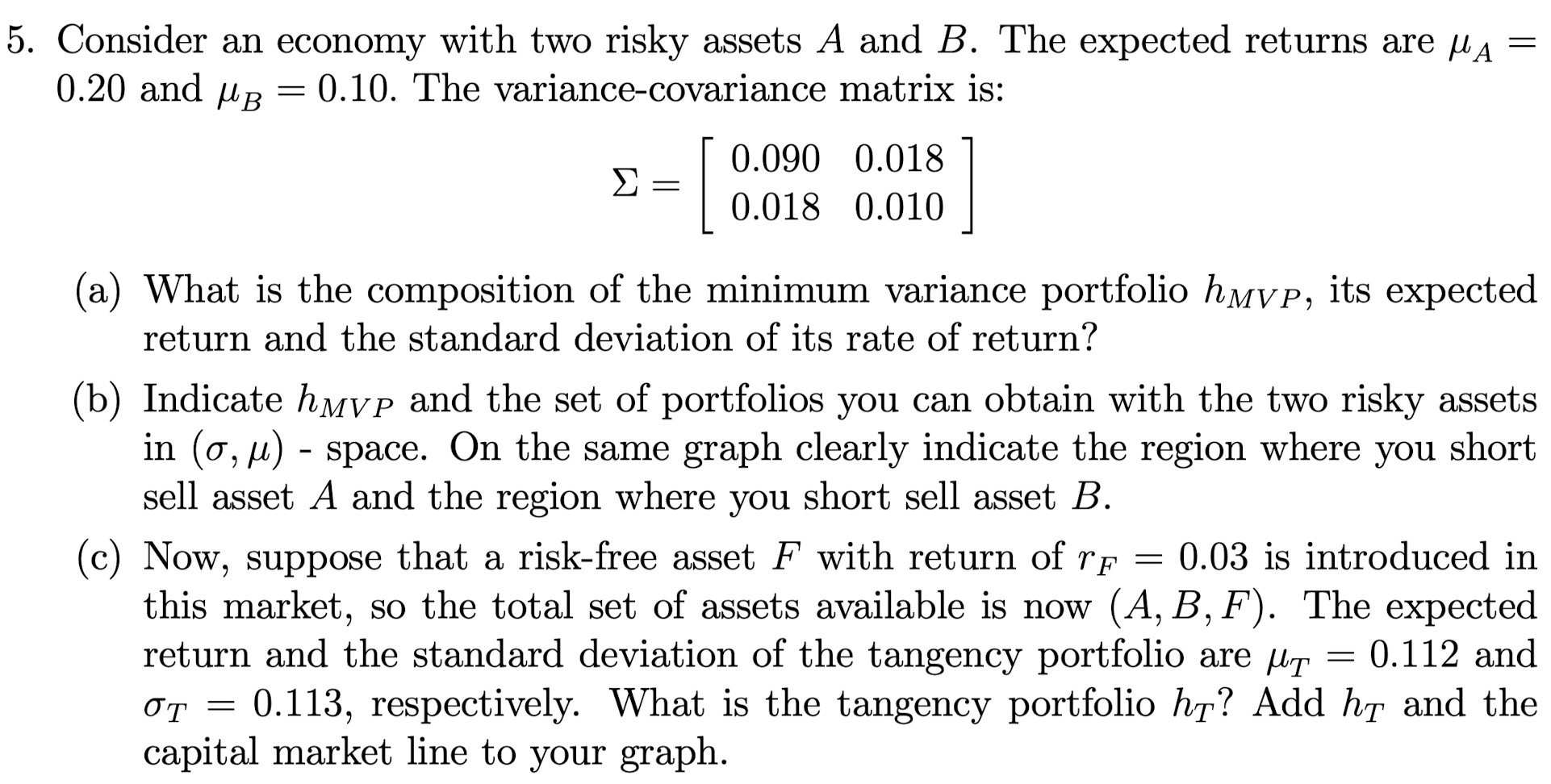
Question
Consider an economy with two risky assets A and B . The expected returns are mu _(A)= 0.20 and mu _(B)=0.10 . The variance-covariance
Consider an economy with two risky assets
Aand
B. The expected returns are
\\\\mu _(A)=\ 0.20 and
\\\\mu _(B)=0.10. The variance-covariance matrix is:\
\\\\Sigma =[[0.090,0.018],[0.018,0.010]]\ (a) What is the composition of the minimum variance portfolio
h_(MVP), its expected\ return and the standard deviation of its rate of return?\ (b) Indicate
h_(MVP)and the set of portfolios you can obtain with the two risky assets\ in
(\\\\sigma ,\\\\mu )- space. On the same graph clearly indicate the region where you short\ sell asset
Aand the region where you short sell asset
B.\ (c) Now, suppose that a risk-free asset
Fwith return of
r_(F)=0.03is introduced in\ this market, so the total set of assets available is now
(A,B,F). The expected\ return and the standard deviation of the tangency portfolio are
\\\\mu _(T)=0.112and\
\\\\sigma _(T)=0.113, respectively. What is the tangency portfolio
h_(T)? Add
h_(T)and the\ capital market line to your graph.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


