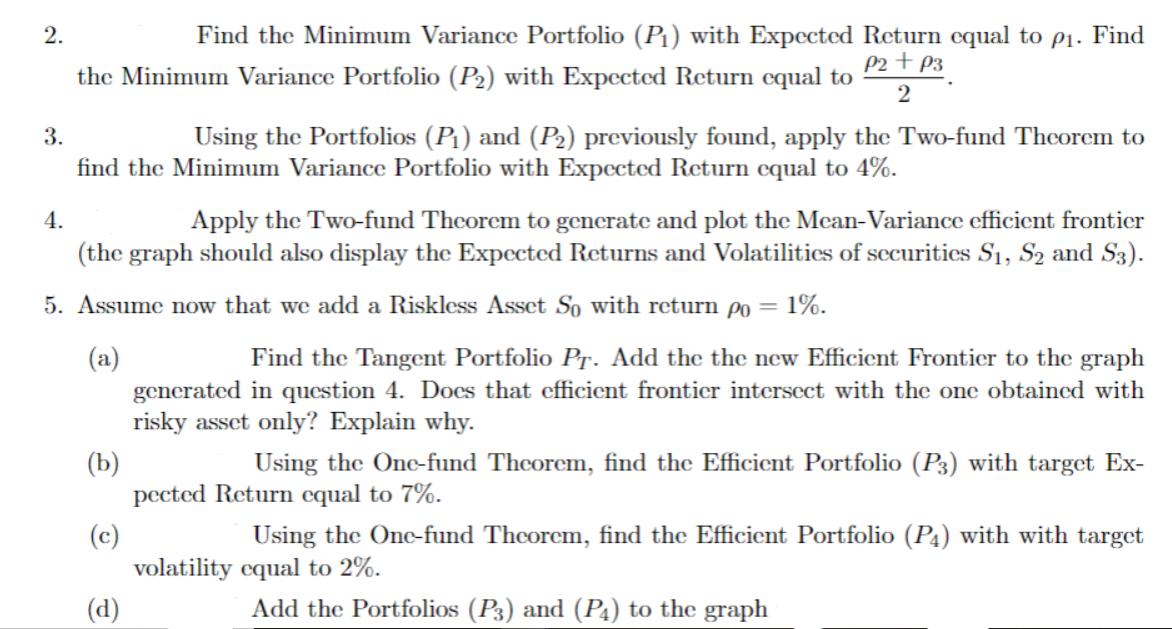
Consider an Investment Universe made of 3 stocks S1, S2 and S3 with the following characteristics: 0.010 0.002 0.001 Covariance matrix: = 0.002 0.011 0.003 0.001 0.003 0.020, P1 4.27% Expected Return vector: p = P2 0.15% P3 2.85% Find the Global Minimal Variance Portfolio. 2. 3. 4. Find the Minimum Variance Portfolio (P1) with Expected Return equal to p. Find P2+P3 the Minimum Variance Portfolio (P2) with Expected Return equal to 2 Using the Portfolios (P1) and (P2) previously found, apply the Two-fund Theorem to find the Minimum Variance Portfolio with Expected Return equal to 4%. Apply the Two-fund Theorem to generate and plot the Mean-Variance efficient frontier (the graph should also display the Expected Returns and Volatilities of securities S1, S2 and S3). 5. Assume now that we add a Riskless Asset So with return po = 1%. (a) Find the Tangent Portfolio Pr. Add the the new Efficient Frontier to the graph generated in question 4. Does that efficient frontier intersect with the one obtained with risky asset only? Explain why. (b) Using the One-fund Theorem, find the Efficient Portfolio (P3) with target Ex- pected Return equal to 7%. (c) (d) Using the One-fund Theorem, find the Efficient Portfolio (P4) with with target volatility equal to 2%. Add the Portfolios (P3) and (P4) to the graph